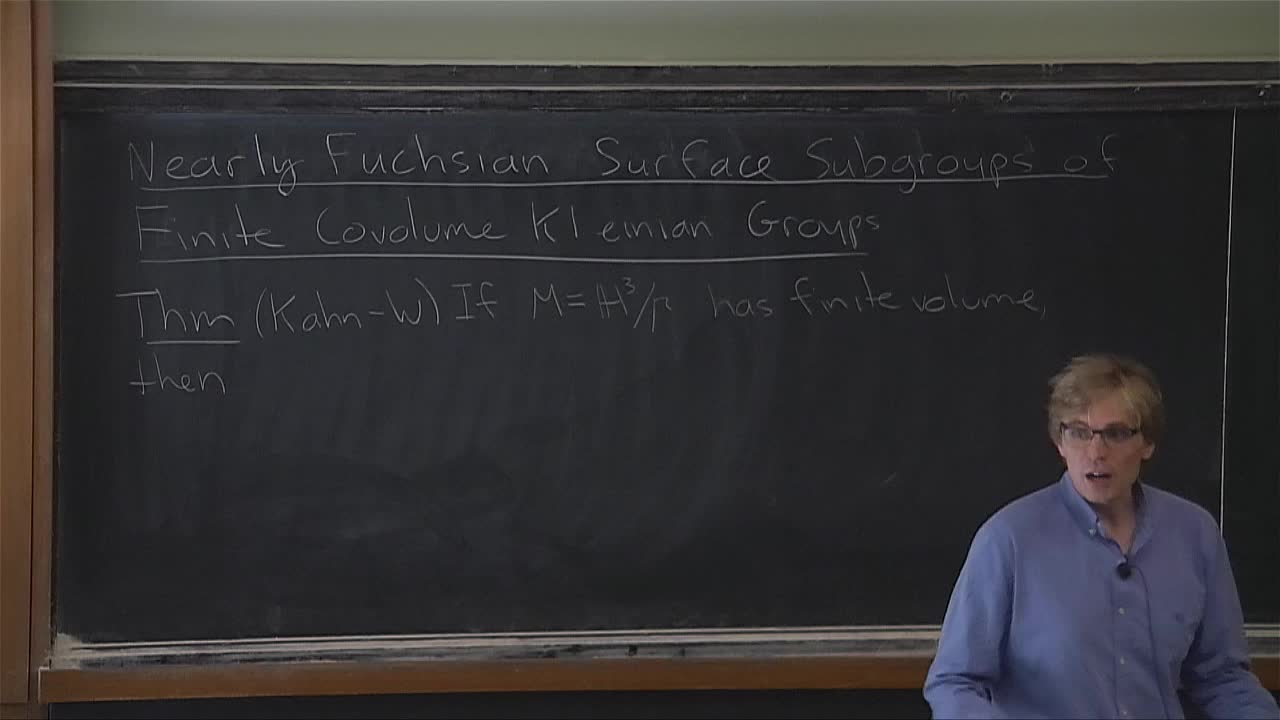Wright, Alexander (19..-.... ; enseignant-chercheur en astrophysique des particules)
- Mathématiques
- Grenoble
- eem2018
- Teichmüller dynamics mapping class groups and applications
- surface subgroups
- covolume Kleinian groups
- Grenoble
- eem2018
- Teichmüller dynamics mapping class groups and applications
- Mirzakhani
- earthquakes
- Grenoble
- eem2018
- Teichmüller dynamics mapping class groups and applications
- Mirzakhani
Vidéos
A. Wright - Mirzakhani's work on Earthquakes (Part 3)
WRIGHT Alexander
We will give the proof of Mirzakhani's theorem that the earthquake flow and Teichmuller unipotent flow are measurably isomorphic. We will assume some familiarity with quadratic differentials, but no
A. Wright - Mirzakhani's work on Earthquakes (Part 1)
WRIGHT Alexander
We will give the proof of Mirzakhani's theorem that the earthquake flow and Teichmuller unipotent flow are measurably isomorphic. We will assume some familiarity with quadratic differentials, but no
A. Wright - Mirzakhani's work on Earthquakes (Part 2)
WRIGHT Alexander
We will give the proof of Mirzakhani's theorem that the earthquake flow and Teichmuller unipotent flow are measurably isomorphic. We will assume some familiarity with quadratic differentials, but no
A. Wright - Nearly Fuchsian surface subgroups of finite covolume Kleinian groups
WRIGHT Alexander
Multicurves have played a fundamental role in the study of mapping class groups of surfaces since the work of Dehn. A beautiful method of describing such systems on the n-punctured disk is given by