Notice
Calista Bernard - Applications of twisted homology operations for E_n-algebras
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
An E_n-algebra is a space equipped with a multiplication that is commutative up to homotopy. Such spaces arise naturally in geometric topology, number theory, and mathematical physics; some examples include classifying spaces of braid groups, spaces of long knots, and classifying spaces of general linear groups. The structure of an E_n-algebra gives rise to operations on the homology of a space, and these operations prove quite useful for studying homology. In the 70s F. Cohen and J.P. May gave a complete description of operations on the mod p homology of E_n-algebras, and more recently I have worked on generalizing their results to homology with twisted coefficients. In this talk I will give a brief introduction to E_n-algebras and the theory of operations, and I will then discuss work in progress on applications of this theory to studying the homology of special linear groups SL_n(Z) and to studying the twisted homology of mixed braid groups.
Thème
Sur le même thème
-
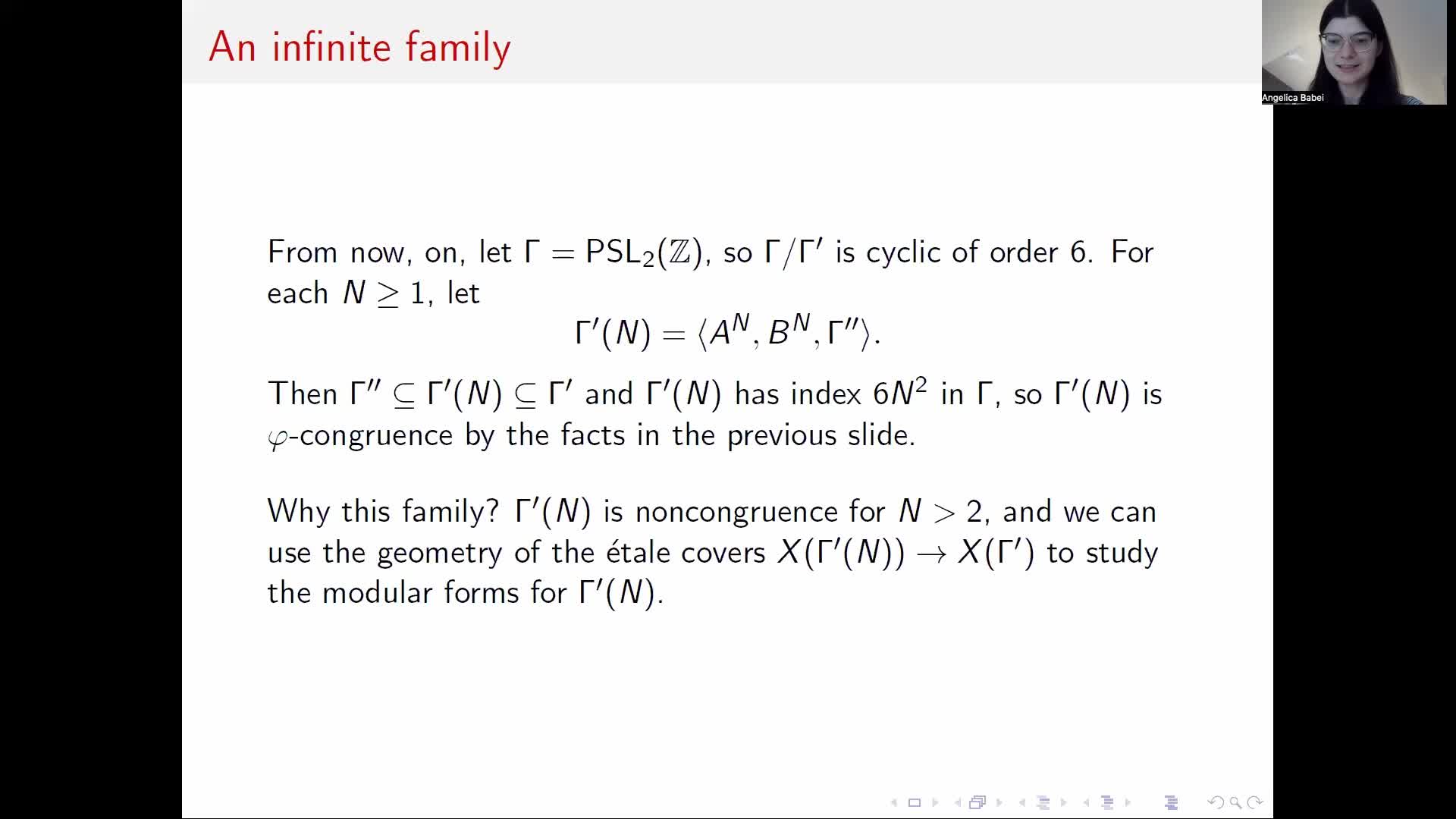
Angelica Babei - A family of $\phi$-congruence subgroups of the modular group
A family of $\phi$-congruence subgroups of the modular group
-
Discussion autour du film "Grenoble par ses bords" (Réalisation : Naïm Aït Sidhoum, 2022)
AïT-SIDHOUM Naïm
TIXIER Nicolas
Rencontre autour du film "Grenoble par ses bords" de Naïm Aït-Sidhoum.