Schulze, Felix (19..-....)
- Mathématiques
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
- weak mean curvature flow
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
- weak mean curvature flow
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
Vidéos
F. Schulze - Mean curvature flow with generic initial data
Mean curvature flow is the gradient flow of the area functional and constitutes a natural geometric heat equation on the space of hypersurfaces in an ambient Riemannian manifold. It is believed,
F. Schulze - An introduction to weak mean curvature flow 1
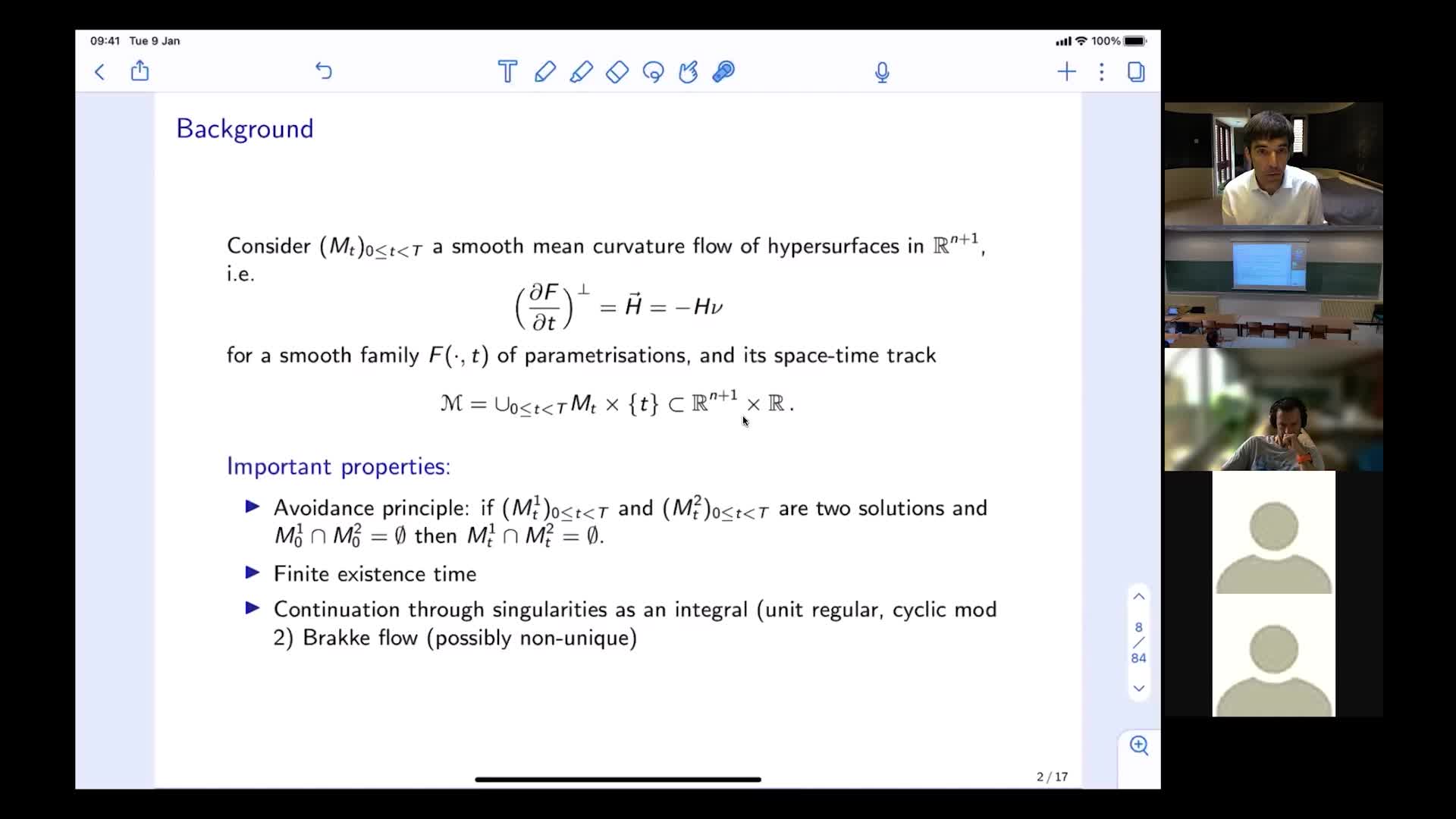
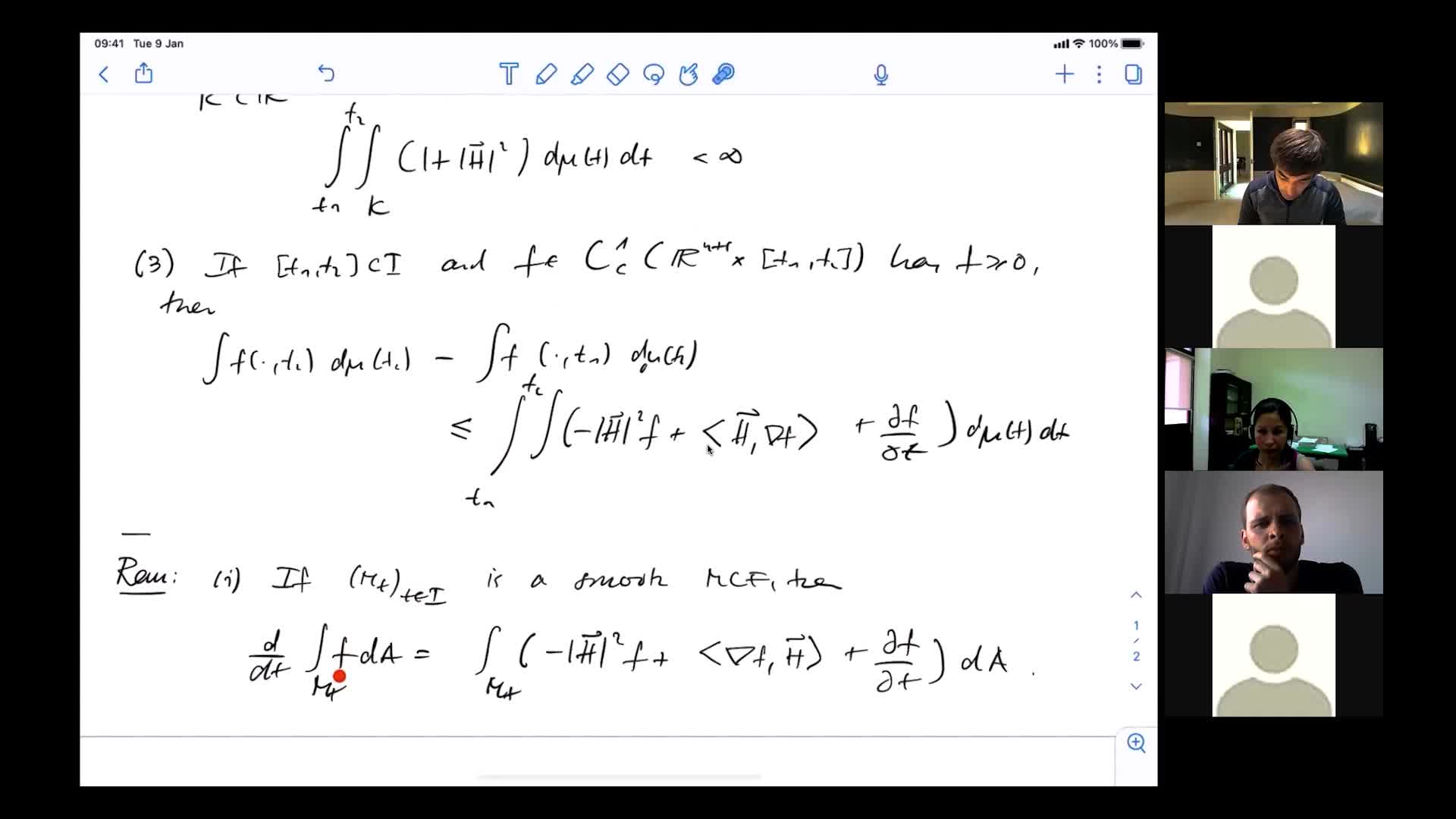
It has become clear in recent years that to understand mean curvature flow through singularities it is essential to work with weak solutions to mean curvature flow. We will give a brief
F. Schulze - An introduction to weak mean curvature flow 2
It has become clear in recent years that to understand mean curvature flow through singularities it is essential to work with weak solutions to mean curvature flow. We will give a brief
F. Schulze - An introduction to weak mean curvature flow 4
It has become clear in recent years that to understand mean curvature flow through singularities it is essential to work with weak solutions to mean curvature flow. We will give a brief
F. Schulze - An introduction to weak mean curvature flow 3
It has become clear in recent years that to understand mean curvature flow through singularities it is essential to work with weak solutions to mean curvature flow. We will give a brief