Notice
Central Limit theorem for quasi-local statistics of point processes with fast decay of correlations (workshop ERC Nemo Processus ponctuels et graphes aléatoires unimodulaires)
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
We shall consider Euclidean stationary point processes which have fast decay of correlations i.e., their correlation functions factorize upto an additive error decaying exponentially in the separation distance. By a quasi-local statistic of the point process, we refer to statistics that can be expressed as sum of contributions from the points and the contribution of every point being determined by a random ball around the point whose radius has an exponential tail. There are many well-known point processes and statistics that satisfy these conditions and I will present a generic central limit theorem for the same assuming some ‘verifiable’ moment conditions and a ‘non-trivial’ variance lower bound. This is based on a joint work with B. Blaszczyszyn and J. E. Yukich. We will then discuss a similar generic central limit theorems for point processes on discrete Cayley graphs with polynomial growth. These results apply for quasi-local statistics of many off-critical spin models on such graphs. For example, the off-critical Ising model and level sets of the Massive Gaussian free field. This is joint work with T. R. Reddy and S. Vadlamani. In view of these results and more results on asymptotic normality for statistics of other random graph models, one is lead to ask the same question about local or quasi-local statistics on unimodular random graphs.
Thème
Documentation
Documents pédagogiques
Dans la même collection
-
Absence of percolation for Poisson outdegree-one graphs (workshop ERC Nemo Processus ponctuels et g…
CoupierDavidA Poisson outdegree-one graph is a directed graph based on a marked Poisson point process such that each vertex has only one outgoing edge. We state the absence of percolation for such graphs
-
Spectral embedding for graph classification (workshop ERC Nemo Processus ponctuels et graphes aléat…
LelargeMarcLearning on graphs requires a graph feature representation able to discriminate among different graphs while being amenable to fast computation. The graph isomorphism problem tells us that no
-
A stable marriage between order and disorder (workshop ERC Nemo Processus ponctuels et graphes aléa…
LastGünterStable matchings were introduced in a seminal paper by Gale and Shapley (1962) and play an important role in economics. Following closely Holroyd, Pemantle, Peres and Schramm (2009), we shall
-
A notion of entropy for limits of sparse marked graphs (workshop ERC Nemo Processus ponctuels et gr…
AnantharamVenkatBordenave and Caputo (2014) defined a notion of entropy for probability distributions on rooted graphs with finite expected degree at the root. When such a probability distribution \rho has finite BC
-
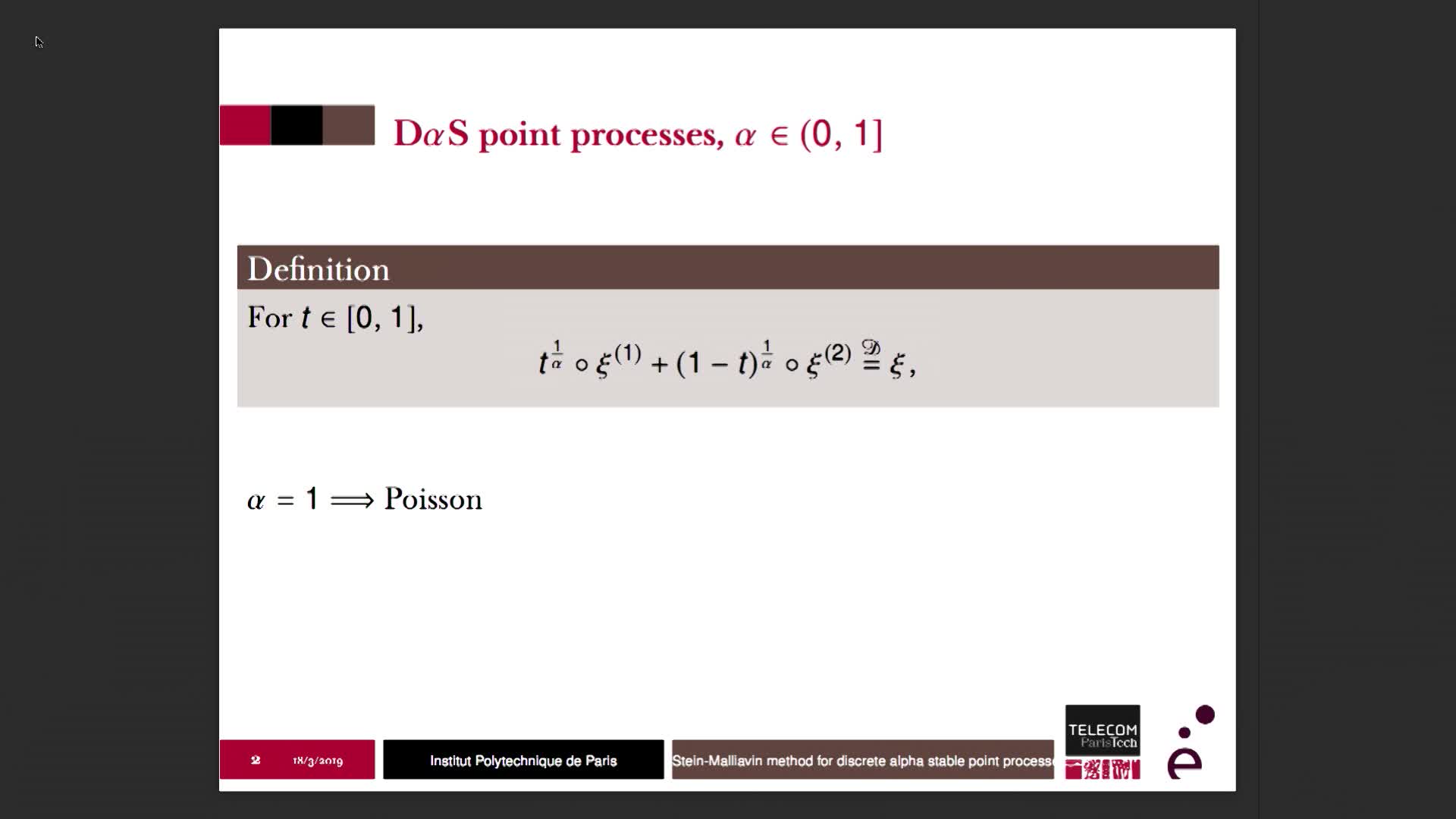
Stein-Malliavin method for discrete alpha stable point processes (workshop ERC Nemo Processus ponct…
DecreusefondLaurentThe notion of discrete alpha-stable point processes generalizes to point processes the notion of stable distribution. It has been introduced and studied by Davydov, Molchanov and Zuyev a few
-
Emergence of extended states at zero in the spectrum of sparse random graphs (workshop ERC Nemo Pro…
SalezJustinWe confirm the long-standing prediction that c=e≈2.718 is the threshold for the emergence of a non-vanishing absolutely continuous part (extended states) at zero in the limiting spectrum of the
-
Sampling cluster point processes: a review (workshop ERC Nemo Processus ponctuels et graphes aléato…
BrémaudPierreThe theme of this talk is the sampling of cluster and iterated cluster point processes. It is partially a review, mainly of the Brix–Kendall exact sampling method for cluster point processes and
-
Point processes, cost and the growth of rank for locally compact groups (workshop ERC Nemo Processu…
AbertMiklósThe cost of a vertex transitive graph is the infimum of the expected degree of an invariant random wiring of the graph. Similarly, one can define the cost of a point process on a homogeneous
-
On the notion of dimension of unimodular discrete spaces (workshop ERC Nemo Processus ponctuels et …
KhezeliAliIn this talk we will define notions of dimension for unimodular random graphs and point-stationary point processes. These notions are in spirit similar to the Minkowski dimension and the
-
-
Subdiffusivity of random walks on random planar maps, via stationarity (workshop ERC Nemo Processus…
CurienNicolasRandom planar maps have been the subject of numerous studies over the last years. They are instance of stationary and reversible random planar maps exhibiting a non-conventional geometry at
-
Strict monotonicity of percolation thresholds under covering maps (workshop ERC Nemo Processus ponc…
MartineauSébastienPercolation is a model for propagation in porous media that as introduced in 1957 by Broadbent and Hammersley. An infinite graph G models the geometry of the situation and a parameter p