Bamler, Richard H. (19..-.... ; mathématicien)
- Mathématiques
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
- Grenoble
- eem2021
Vidéos
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.
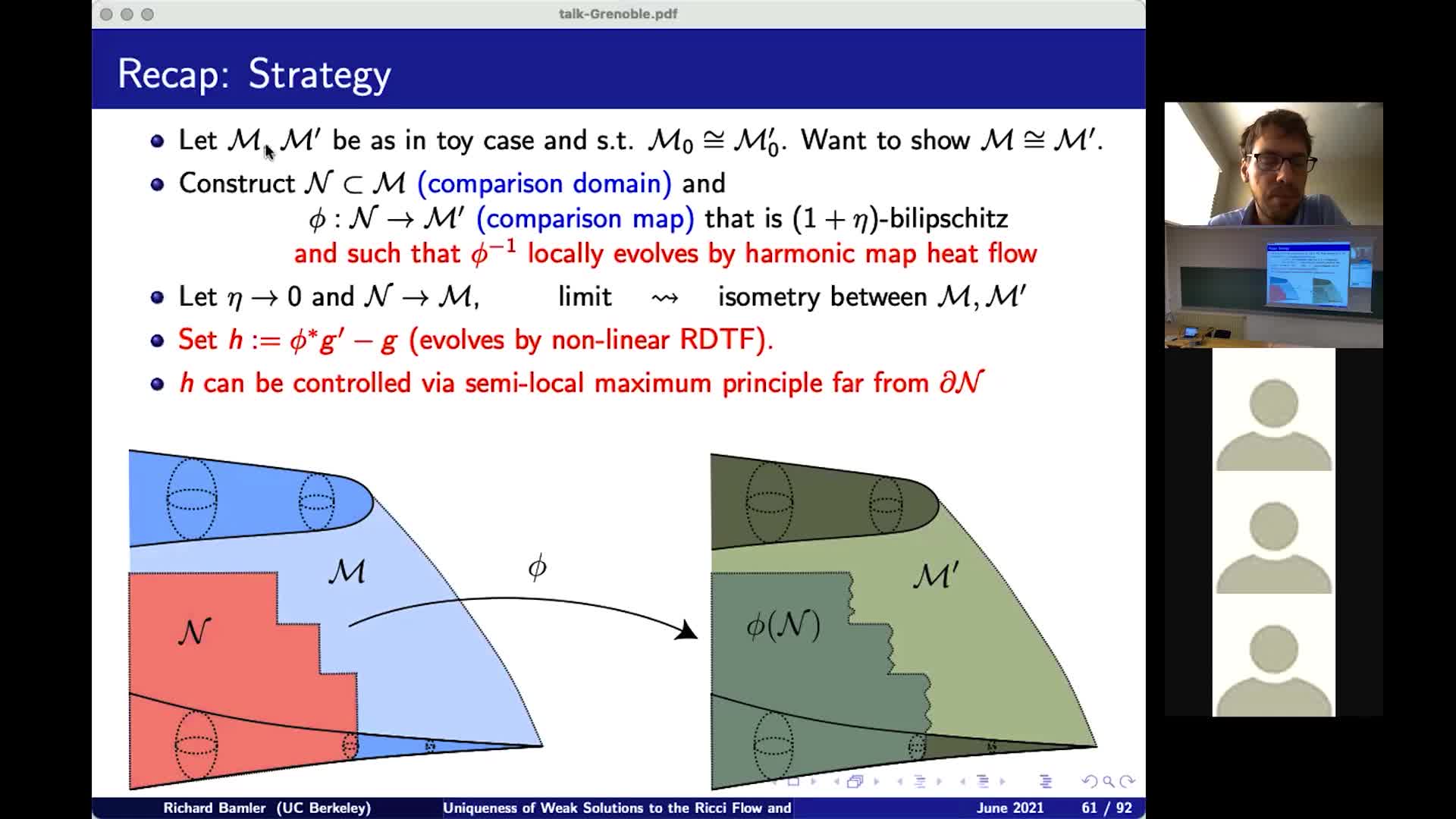
R. Bamler - Uniqueness of Weak Solutions to the Ricci Flow and Topological Applications 3
I will present recent work with Kleiner in which we verify two topological conjectures using Ricci flow. First, we classify the homotopy type of every 3-dimensional spherical space form. This
R. Bamler - Uniqueness of Weak Solutions to the Ricci Flow and Topological Applications 1
I will present recent work with Kleiner in which we verify two topological conjectures using Ricci flow. First, we classify the homotopy type of every 3-dimensional spherical space form. This
R. Bamler - Uniqueness of Weak Solutions to the Ricci Flow and Topological Applications 4
I will present recent work with Kleiner in which we verify two topological conjectures using Ricci flow. First, we classify the homotopy type of every 3-dimensional spherical space form. This
R. Bamler - Uniqueness of Weak Solutions to the Ricci Flow and Topological Applications 2
I will present recent work with Kleiner in which we verify two topological conjectures using Ricci flow. First, we classify the homotopy type of every 3-dimensional spherical space form. This