Song, Antoine (19..-....)
- Mathématiques
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
- minimal volume
- Einstein 4-manifolds
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
- curvature constraints and spaces of metrics
- minimal volume
- Grenoble
- eem2021
- contraintes de courbures et espaces métriques
Vidéos
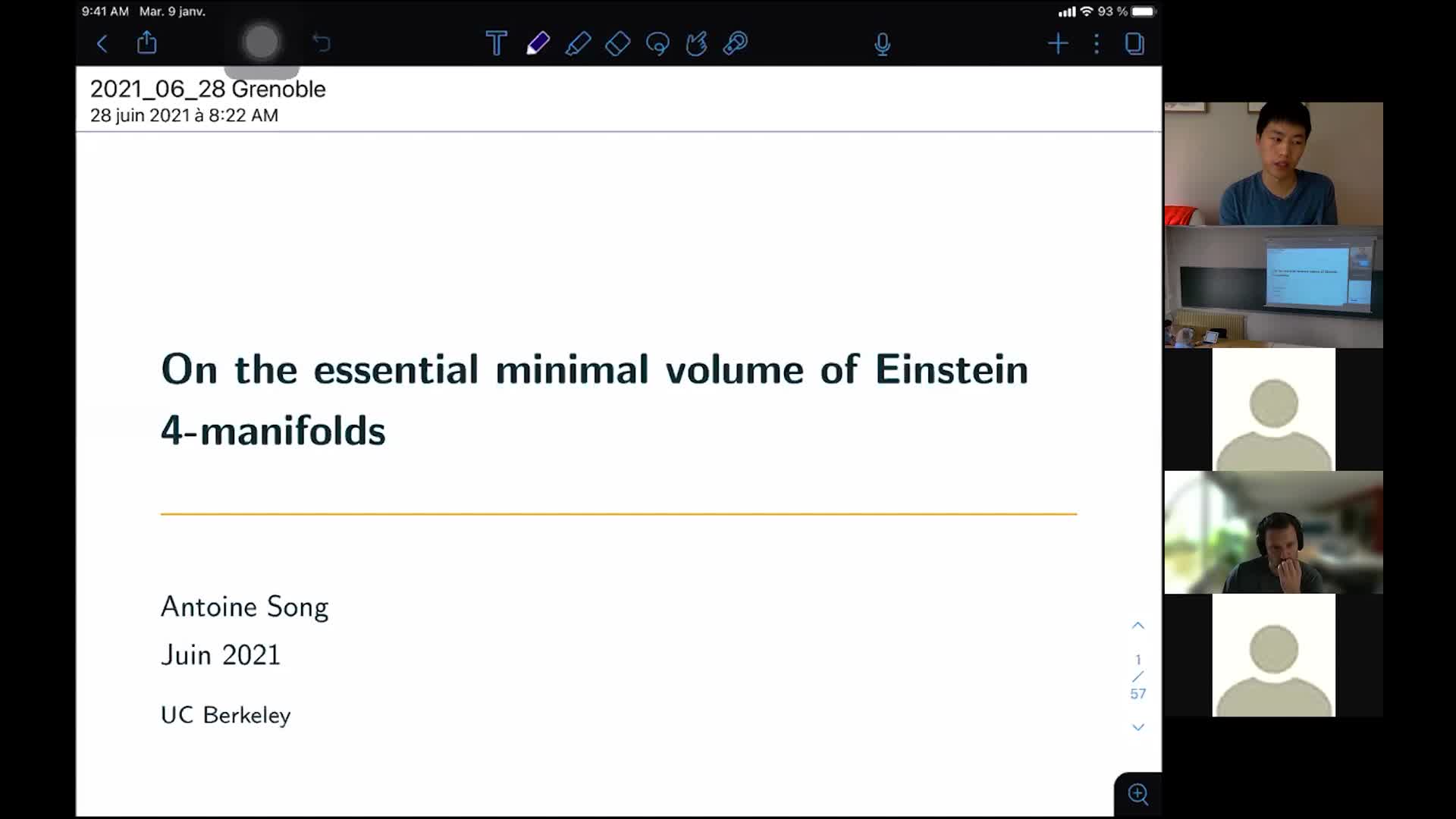
A. Song - On the essential minimal volume of Einstein 4-manifolds
SONG Antoine
Given a positive epsilon, a closed Einstein 4-manifold admits a natural thick-thin decomposition. I will explain how, for any delta, one can modify the Einstein metric to a bounded sectional
A. Song - What is the (essential) minimal volume? 4
SONG Antoine
I will discuss the notion of minimal volume and some of its variants. The minimal volume of a manifold is defined as the infimum of the volume over all metrics with sectional curvature between
A. Song - What is the (essential) minimal volume? 2
SONG Antoine
I will discuss the notion of minimal volume and some of its variants. The minimal volume of a manifold is defined as the infimum of the volume over all metrics with sectional curvature between
A. Song - What is the (essential) minimal volume? 3
SONG Antoine
I will discuss the notion of minimal volume and some of its variants. The minimal volume of a manifold is defined as the infimum of the volume over all metrics with sectional curvature between
A. Song - What is the (essential) minimal volume? 1
SONG Antoine
I will discuss the notion of minimal volume and some of its variants. The minimal volume of a manifold is defined as the infimum of the volume over all metrics with sectional curvature between