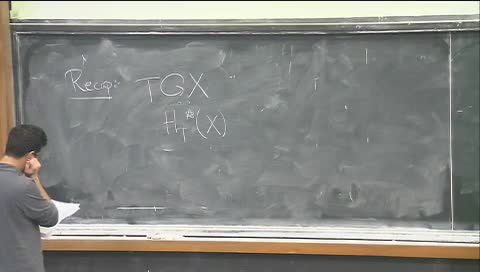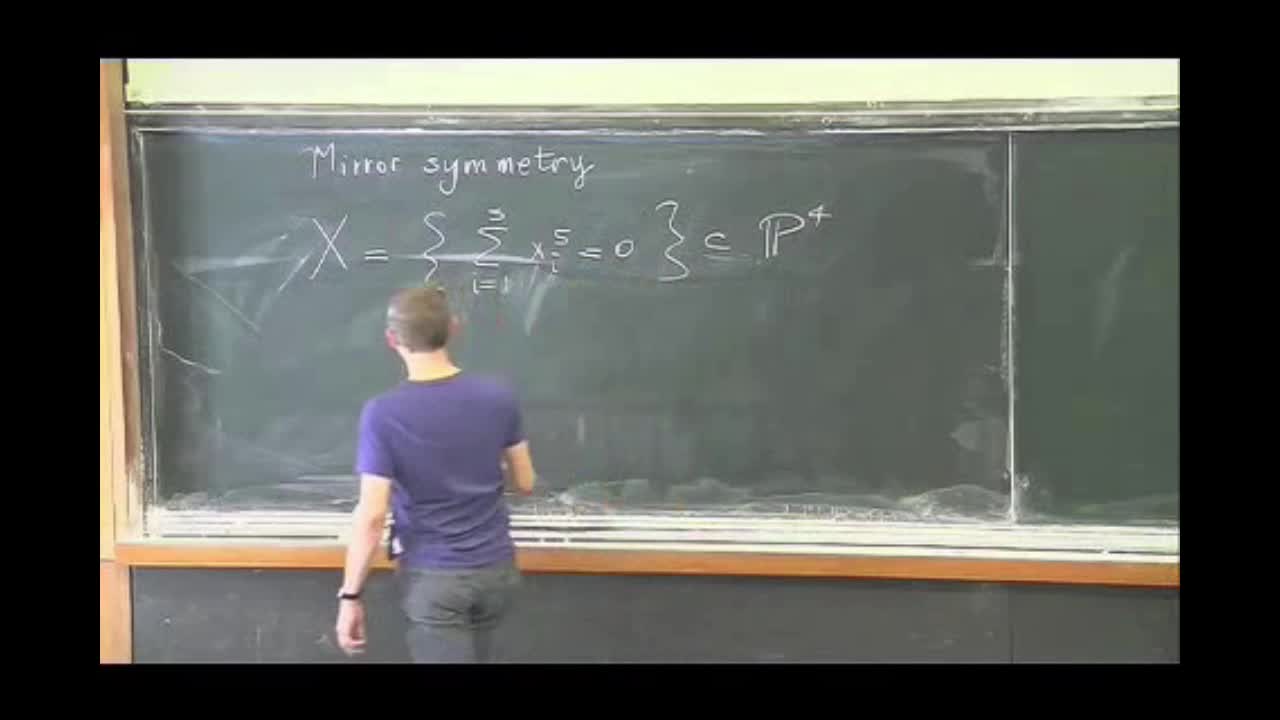2011

Vidéos
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
LEE Yuan-Pin
In these lectures, Gromov-Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be explained. Some
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
LEE Yuan-Pin
In these lectures, Gromov-Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be explained. Some
Davesh Maulik - Introduction to Donaldson-Thomas theory (Part 3)
MAULIK Davesh
We will give an introduction to Donaldson-Thomas theory and some basic tools and computations. In the last lecture, we hope to explain some aspects of the proof of the GW/DT correspondence for toric
Nicolas Perrin - Quantum K-theory of some homogeneous spaces
PERRIN Nicolas
Quantum K-theory is as quantum cohomology a generalisation of the classical coho- mology algebra of a variety X . In this talk I will explain the connection between the geometry of the moduli
Etienne Mann - Quantum D-module for hypersurfaces
MANN Etienne
Out of the quantum product of a projective smooth variety, we can construct a vector bundle with a at connection and a pairing, these data are called quantum D-modules. In a recent paper of
Laurent Manivel - The Satake correspondence in quantum cohomology
MANIVEL Laurent
The Satake isomorphism identi es the irreducible representations of a semisimple algebraic group with the intersection cohomologies of the Schubert varieties in the a ne Grassmannian of
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
In these lectures, Gromov{Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be
Aaron Pixton - The stable pairs equivariant descendent vertex
PIXTON Aaron
The counting function associated to the moduli space of stable pairs on a 3-fold X is conjectured to give the Laurent expansion of a rational function. For toric X , this conjecture can be
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
LEE Yuan-Pin
In these lectures, Gromov{Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be
Rahul Pandharipande - Stable quotients and relations in the tautological ring
PANDHARIPANDE Rahul
The topic concerns relations among the kappa classes in the tautological ring of the moduli space of genus g curves. After a discussion of classical constructions in Wick form, we derive an
Mulase Motohico - Counting the lattice points on the moduli space of curves
MULASE Motohico
In this talk I will explain the idea of the Laplace transform that connects a counting problem in the A-model side with a recursion formula based on complex analysis in the B-model side, using a
Davesh Maulik - Introduction to Donaldson-Thomas theory (Part 1)
MAULIK Davesh
We will give an introduction to Donaldson-Thomas theory and some basic tools and computations. In the last lecture, we hope to explain some aspects of the proof of the GW/DT
Alessandro Chiodo - Towards global mirror symmetry (Part 3)
CHIODO Alessandro
Mirror symmetry is a phenomenon which inspired fundamental progress in a wide range of disciplines in mathe- matics and physics in the last twenty years; we will review here a number of results
Alessandro Chiodo - Towards global mirror symmetry (Part 1)
CHIODO Alessandro
Mirror symmetry is a phenomenon which inspired fundamental progress in a wide range of disciplines in mathematics and physics in the last twenty years; we will review here a number of results going
Alessandro Chiodo - Towards global mirror symmetry (Part 2)
CHIODO Alessandro
Mirror symmetry is a phenomenon which inspired fundamental progress in a wide range of disciplines in mathe- matics and physics in the last twenty years; we will review here a number of results
Intervenants et intervenantes
Professeur des Universités à Sorbonne université (en 2025). Membre de l'UMR7586 - Institut de Mathématiques de Jussieu-Paris Rive Gauche
Mathématicien. Titulaire d'un doctorat en mathématiques de l'université Berkeley (1999). Enseignant chercheur à l'université de l'Utah (2011)
En poste à l'Institut Fourier, Grenoble I (en 1998)
En 2018, en poste à l'institut de Mathématiques de Toulouse. Directeur d'une thèse en Mathématiques. Géométrie algébrique complexe à Aix-Marseille en 2018
Titulaire d'un Doctorat de Mathématiques (Strasbourg 1, 2005)
Mathématicien. En poste : Université d'Angers, Département de mathématiques, France (en 2021)
Mathémacien. Spécialiste de la géométrie algébrique
En poste : Department of Mathematics, Massachusetts Institute of Technology, USA (en 2018)
Department of mathematics, University of California, Davis CA (en 1993)
Mathématicien, en poste à l'ETH Zürich (2017)
Auteur d'une thèse de doctorat en Mathématiques à Nice en 2013
Mathématicien. Chercheur à l'université du Michigan. Spécialité : géométrie énumérative. Joueur d'échecs (2011)