Notice
Nicolas Perrin - Quantum K-theory of some homogeneous spaces
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
Quantum K-theory is as quantum cohomology a generalisation of the classical coho-
mology algebra of a varietyX. In this talk I will explain the connection between the geometry of themoduli space of stable maps, in particular rational connectedness properties, and the computation ofstructure constants forXa rational homogeneous space. This is based on a joint work with A. Buch,
P.-E. Chaput and L. Mihalcea
Intervention / Responsable scientifique
Thème
Documentation
Liens
Dans la même collection
-
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
LeeYuan-PinIn these lectures, Gromov-Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be explained. Some
-
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
LeeYuan-PinIn these lectures, Gromov-Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be explained. Some
-
Davesh Maulik - Introduction to Donaldson-Thomas theory (Part 3)
MaulikDaveshWe will give an introduction to Donaldson-Thomas theory and some basic tools and computations. In the last lecture, we hope to explain some aspects of the proof of the GW/DT correspondence for toric
-
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
In these lectures, Gromov{Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be
-
Etienne Mann - Quantum D-module for hypersurfaces
MannEtienneOut of the quantum product of a projective smooth variety, we can construct a vector bundle with a at connection and a pairing, these data are called quantum D-modules. In a recent paper of
-
Aaron Pixton - The stable pairs equivariant descendent vertex
PixtonAaronThe counting function associated to the moduli space of stable pairs on a 3-fold X is conjectured to give the Laurent expansion of a rational function. For toric X , this conjecture can be
-
Laurent Manivel - The Satake correspondence in quantum cohomology
ManivelLaurentThe Satake isomorphism identi es the irreducible representations of a semisimple algebraic group with the intersection cohomologies of the Schubert varieties in the a ne Grassmannian of
-
Yuan-Pin Lee - Introduction to Gromov-Witten theory and the crepant transformation conjecture (Part…
LeeYuan-PinIn these lectures, Gromov{Witten theory will be introduced, assuming only basic moduli theory covered in the rst week of the School. Then the Crepant Transformation Conjecture will be
-
Rahul Pandharipande - Stable quotients and relations in the tautological ring
PandharipandeRahulThe topic concerns relations among the kappa classes in the tautological ring of the moduli space of genus g curves. After a discussion of classical constructions in Wick form, we derive an
-
Mulase Motohico - Counting the lattice points on the moduli space of curves
MulaseMotohicoIn this talk I will explain the idea of the Laplace transform that connects a counting problem in the A-model side with a recursion formula based on complex analysis in the B-model side, using a
-
Davesh Maulik - Introduction to Donaldson-Thomas theory (Part 1)
MaulikDaveshWe will give an introduction to Donaldson-Thomas theory and some basic tools and computations. In the last lecture, we hope to explain some aspects of the proof of the GW/DT
-
Alessandro Chiodo - Towards global mirror symmetry (Part 2)
ChiodoAlessandroMirror symmetry is a phenomenon which inspired fundamental progress in a wide range of disciplines in mathe- matics and physics in the last twenty years; we will review here a number of results
Sur le même thème
-
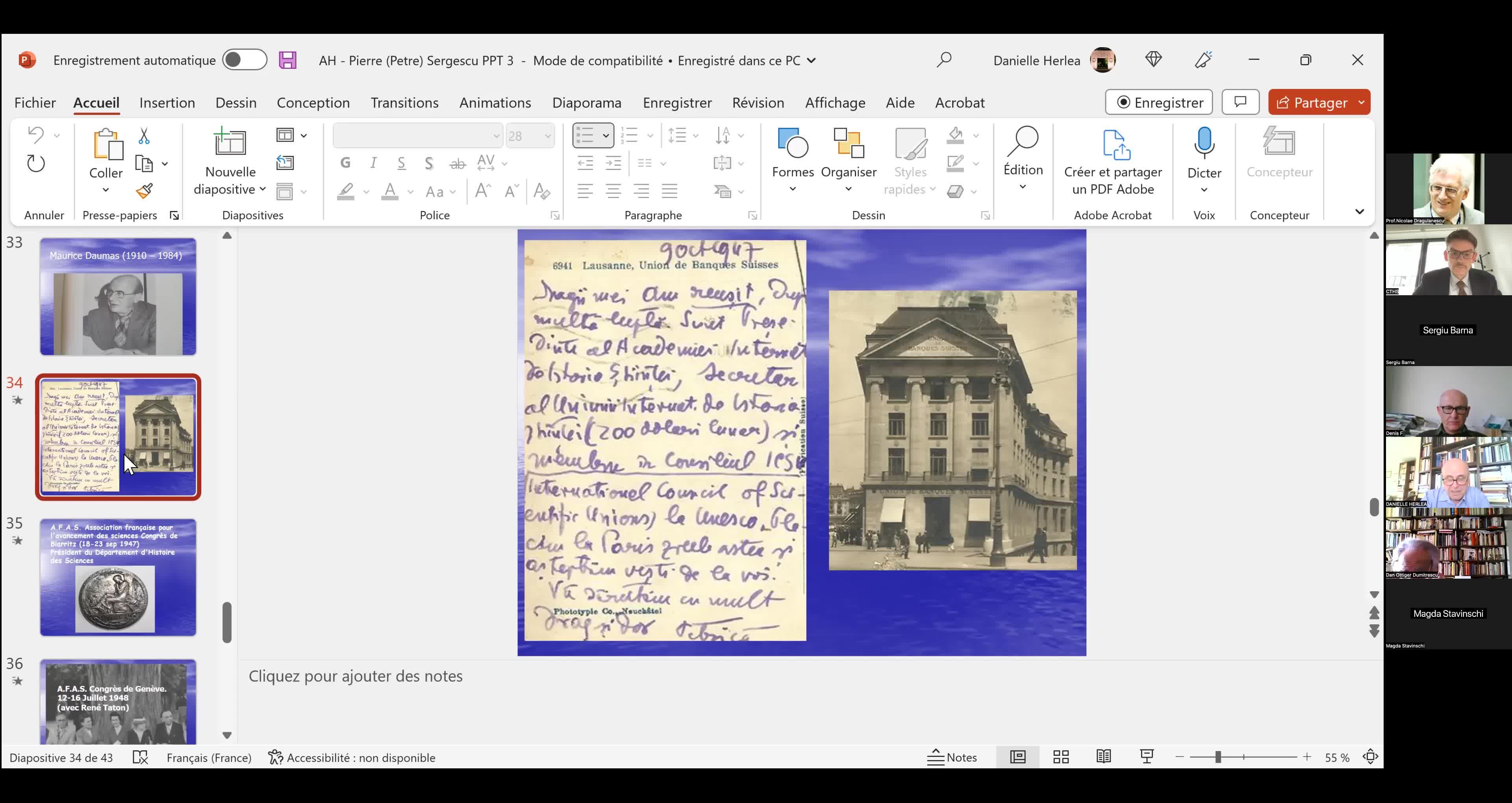
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HerléaAlexandreAlexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
Webinaire sur la rédaction des PGD
LouvetViolaineRédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
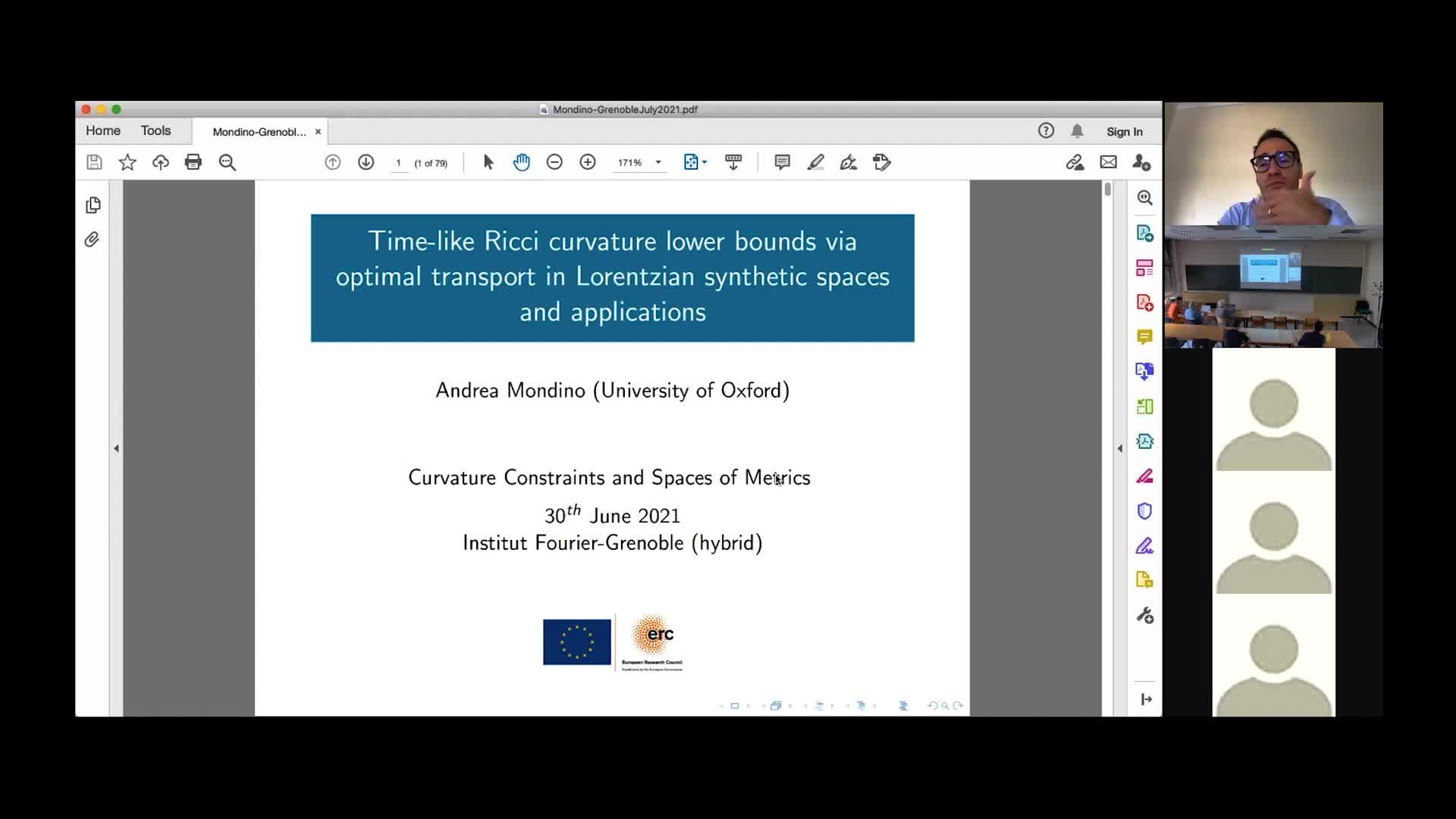
A. Mondino - Time-like Ricci curvature bounds via optimal transport
MondinoAndreaThe goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
-
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass Theorem
LesourdMartinThe study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in
-
J. Wang - Topological rigidity and positive scalar curvature
WangJianIn this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
R. Perales - Recent Intrinsic Flat Convergence Theorems
PeralesRaquelThéorèmes récents de convergence plane intrinsèque
-
J. Fine - Knots, minimal surfaces and J-holomorphic curves
FineJoëlI will describe work in progress, parts of which are joint with Marcelo Alves. Let L be a knot or link in the 3-sphere. I will explain how one can count minimal surfaces in hyperbolic 4-space
-
D. Semola - Boundary regularity and stability under lower Ricci bounds
SemolaDanieleThe theory of non smooth spaces with lower Ricci Curvature bounds has undergone huge developments in the last thirty years. On the one hand the impetus came from Gromov’s precompactness theorem
-
D. Stern - Harmonic map methods in spectral geometry
SternDanielOver the last fifty years, the problem of finding sharp upper bounds for area-normalized Laplacian eigenvalues on closed surfaces has attracted the attention of many geometers, due in part to
-
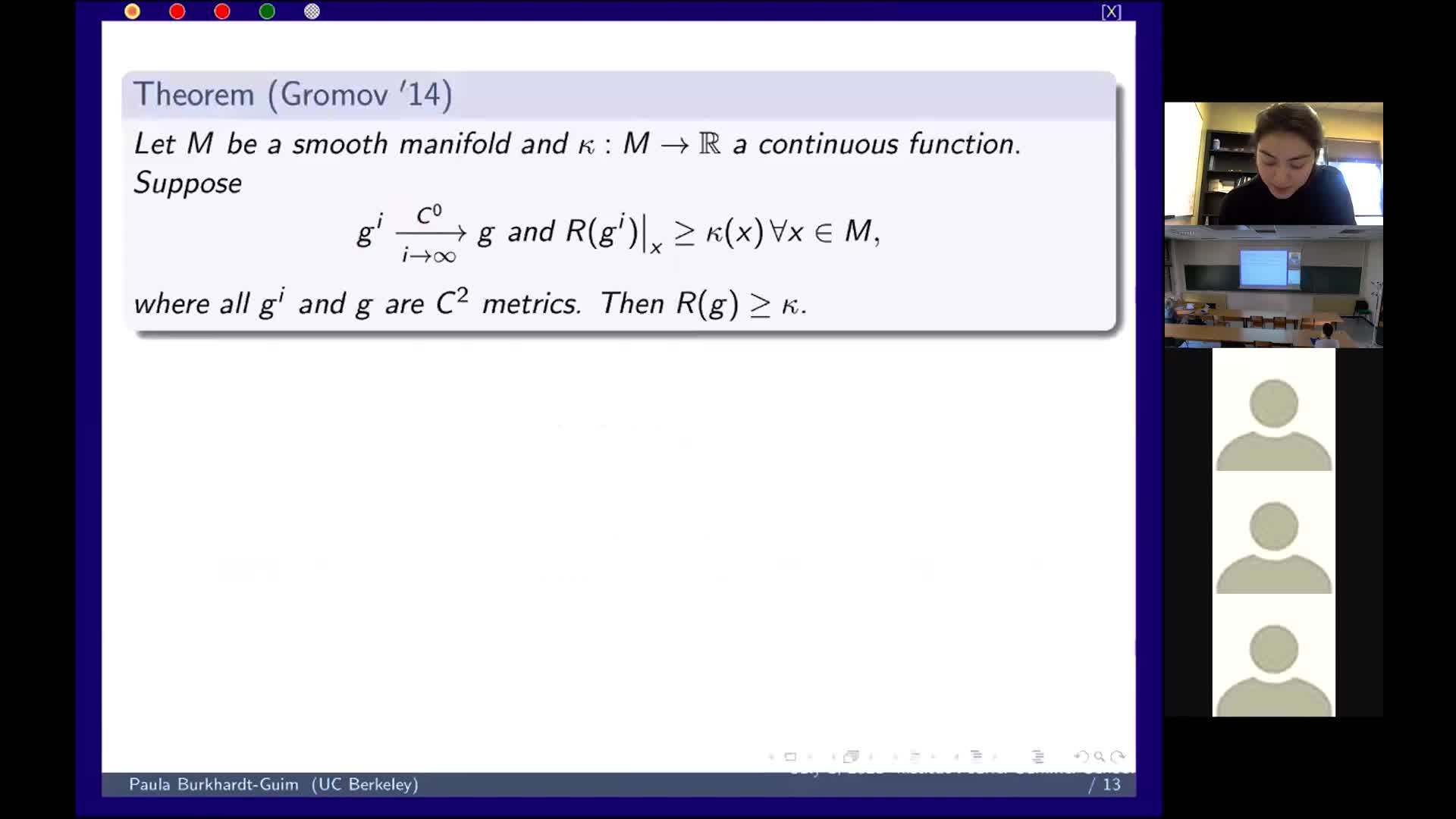
P. Burkhardt - Pointwise lower scalar curvature bounds for C0 metrics via regularizing Ricci flow
Burkhardt-GuimPaulaWe propose a class of local definitions of weak lower scalar curvature bounds that is well defined for C0 metrics. We show the following: that our definitions are stable under greater-than-second
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BamlerRichard H.We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.