Notice
Bettina EICK - Computational group theory, cohomology of groups and topological methods 3
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Intervention / Responsable scientifique
Thème
Avec les mêmes intervenants et intervenantes
-
Bettina EICK - Computational group theory, cohomology of groups and topological methods 4
EickBettinaThe lecture series will give an introduction to the computer algebra system GAP, focussing on calculations involving cohomology. We will describe the mathematics underlying the algorithms, and how to
-
Bettina EICK - Computational group theory, cohomology of groups and topological methods 5
EickBettinaThe lecture series will give an introduction to the computer algebra system GAP, focussing on calculations involving cohomology. We will describe the mathematics underlying the algorithms, and how to
-
Bettina EICK - Computational group theory, cohomology of groups and topological methods 1
EickBettinaThe lecture series will give an introduction to the computer algebra system GAP, focussing on calculations involving cohomology. We will describe the mathematics underlying the algorithms, and how to
Sur le même thème
-
Aurel PAGE - Cohomology of arithmetic groups and number theory: geometric, asymptotic and computati…
PageAurel regisIn this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)
-
Phong NGUYEN - Recent progress on lattices's computations 2
NguyenPhong Q.This is an introduction to the mysterious world of lattice algorithms, which have found many applications in computer science, notably in cryptography. We will explain how lattices are represented by
-
Zachary Himes - On not the rational dualizing module for $\text{Aut}(F_n)$
Bestvina--Feighn proved that $\text{Aut}(F_n)$ is a rational duality group, i.e. there is a $\mathbb{Q}[\text{Aut}(F_n)]$-module, called the rational dualizing module, and a form of Poincar\'e duality
-
Tobias Moede - Coclass theory for nilpotent associative algebras
The coclass of a finite p-group of order p^n and class c is defined as n-c. Using coclass as the primary invariant in the investigation of finite p-groups turned out to be a very fruitful approach.
-
Oussama Hamza - Hilbert series and mild groups
Let $p$ be an odd prime number and $G$ a finitely generated pro-$p$ group. Define $I(G)$ the augmentation ideal of the group algebra of $G$ over $F_p$ and define the Hilbert series of $G$ by: $G(t):
-
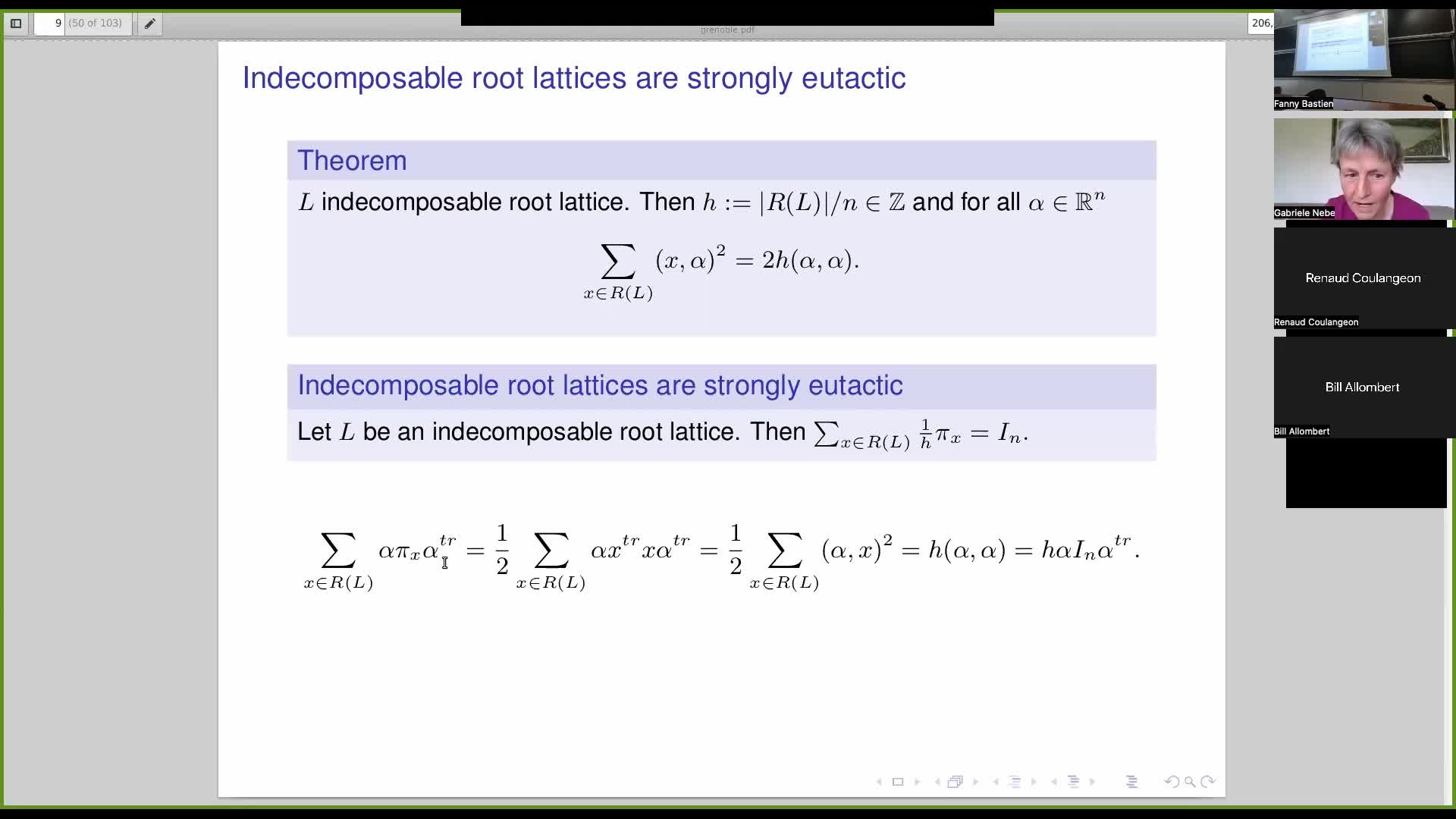
Gabriele NEBE - Lattices, Perfects lattices, Voronoi reduction theory, modular forms, computations …
NebeGabrieleThe talks of Coulangeon will introduce the notion of perfect, eutactic and extreme lattices and the Voronoi's algorithm to enumerate perfect lattices (both Eulcidean and Hermitian). The talk of Nebe
-
Alexander HULPKE - Computational group theory, cohomology of groups and topological methods 4
The lecture series will give an introduction to the computer algebra system GAP, focussing on calculations involving cohomology. We will describe the mathematics underlying the algorithms, and how to
-
Alexander HULPKE - Computational group theory, cohomology of groups and topological methods 5
The lecture series will give an introduction to the computer algebra system GAP, focussing on calculations involving cohomology. We will describe the mathematics underlying the algorithms, and how to
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
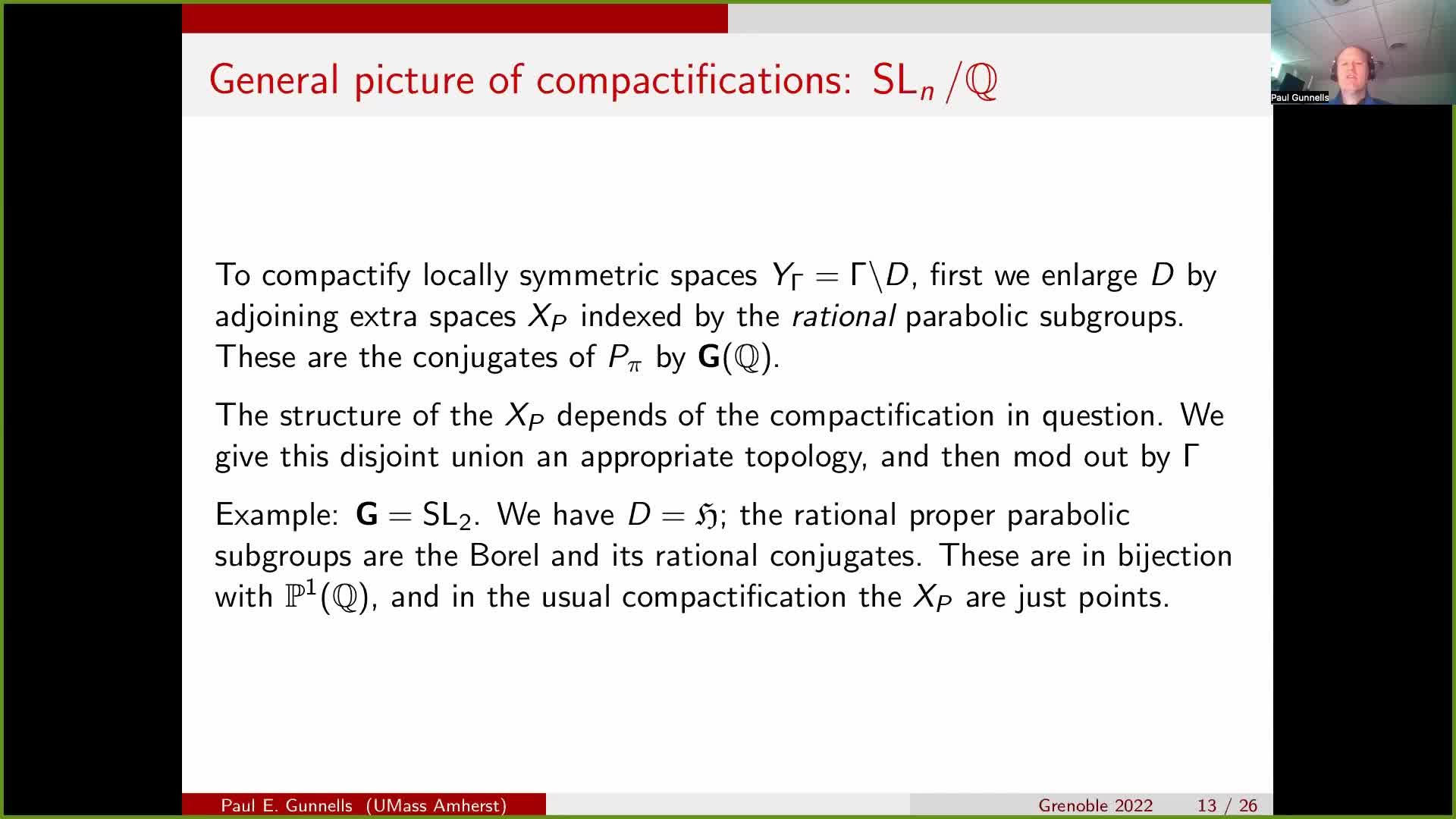
Paul GUNNELLS - Cohomology of arithmetic groups and number theory: geometric, asymptotic and comput…
GunnellsPaul E.In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)
-
Paul GUNNELLS - Cohomology of arithmetic groups and number theory: geometric, asymptotic and comput…
GunnellsPaul E.In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)
-
Paul GUNNELLS - Cohomology of arithmetic groups and number theory: geometric, asymptotic and comput…
GunnellsPaul E.In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)