Notice
A stable marriage between order and disorder (workshop ERC Nemo Processus ponctuels et graphes aléatoires unimodulaires)
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
Stable matchings were introduced in a seminal paper by Gale and Shapley (1962) and play an important role in economics. Following closely Holroyd, Pemantle, Peres and Schramm (2009), we shall first discuss a few basic properties of stable matchings between two discrete point sets (resp. point processes) in Euclidean space, where the points prefer to be close to each other. For comparison we also discuss stable transports from Lebesgue measure to point processes. In the second part of the talk we consider a stable matching between the cubic lattice and a stationary Poisson process (or a determinantal point process) with higher intensity. The matched points form a stationary and ergodic (under lattice shifts) point process with unit intensity that has many remarkable properties. If the intensity of the Poisson process is close to one, then it very much resembles a Poisson process, while for large intensities it approaches the lattice. Moreover, the matched points form a hyperuniform and number rigid point process, in sharp contrast to a Poisson process. Still the pair correlation decays exponentially fast. The talk is based on joint work with M. Klatt and D. Yogeshwaran.
Intervention
Thème
Documentation
Documents pédagogiques
Dans la même collection
-
Eternal family trees and dynamics on unimodular random graphs (workshop ERC Nemo Processus ponctuel…
Haji MirsadeghiMir OmidThis talk is centered on covariant dynamics on unimodular random graphs and random networks (marked graphs), namely maps from the set of vertices to itself which are preserved by graph or
-
-
Subdiffusivity of random walks on random planar maps, via stationarity (workshop ERC Nemo Processus…
CurienNicolasRandom planar maps have been the subject of numerous studies over the last years. They are instance of stationary and reversible random planar maps exhibiting a non-conventional geometry at
-
Strict monotonicity of percolation thresholds under covering maps (workshop ERC Nemo Processus ponc…
MartineauSébastienPercolation is a model for propagation in porous media that as introduced in 1957 by Broadbent and Hammersley. An infinite graph G models the geometry of the situation and a parameter p
-
Comments and problems regarding large graphs. (workshop ERC Nemo Processus ponctuels et graphes alé…
BenjaminiItaiWe will discuss a couple of results and questions regarding the structure of large graphs. These include vertex transitive graphs, expanders and random graphs.
-
Central Limit theorem for quasi-local statistics of point processes with fast decay of correlations…
We shall consider Euclidean stationary point processes which have fast decay of correlations i.e., their correlation functions factorize upto an additive error decaying exponentially in the
-
On the modified Palm version (workshop ERC Nemo Processus ponctuels et graphes aléatoires unimodula…
ThorissonHermannThe Palm version of a stationary random measure is an important tool in probability. It is however not well known that there are in fact two Palm versions, with related but different
-
Absence of percolation for Poisson outdegree-one graphs (workshop ERC Nemo Processus ponctuels et g…
CoupierDavidA Poisson outdegree-one graph is a directed graph based on a marked Poisson point process such that each vertex has only one outgoing edge. We state the absence of percolation for such graphs
-
Spectral embedding for graph classification (workshop ERC Nemo Processus ponctuels et graphes aléat…
LelargeMarcLearning on graphs requires a graph feature representation able to discriminate among different graphs while being amenable to fast computation. The graph isomorphism problem tells us that no
-
On the notion of dimension of unimodular discrete spaces (workshop ERC Nemo Processus ponctuels et …
KhezeliAliIn this talk we will define notions of dimension for unimodular random graphs and point-stationary point processes. These notions are in spirit similar to the Minkowski dimension and the
-
A notion of entropy for limits of sparse marked graphs (workshop ERC Nemo Processus ponctuels et gr…
AnantharamVenkatBordenave and Caputo (2014) defined a notion of entropy for probability distributions on rooted graphs with finite expected degree at the root. When such a probability distribution \rho has finite BC
-
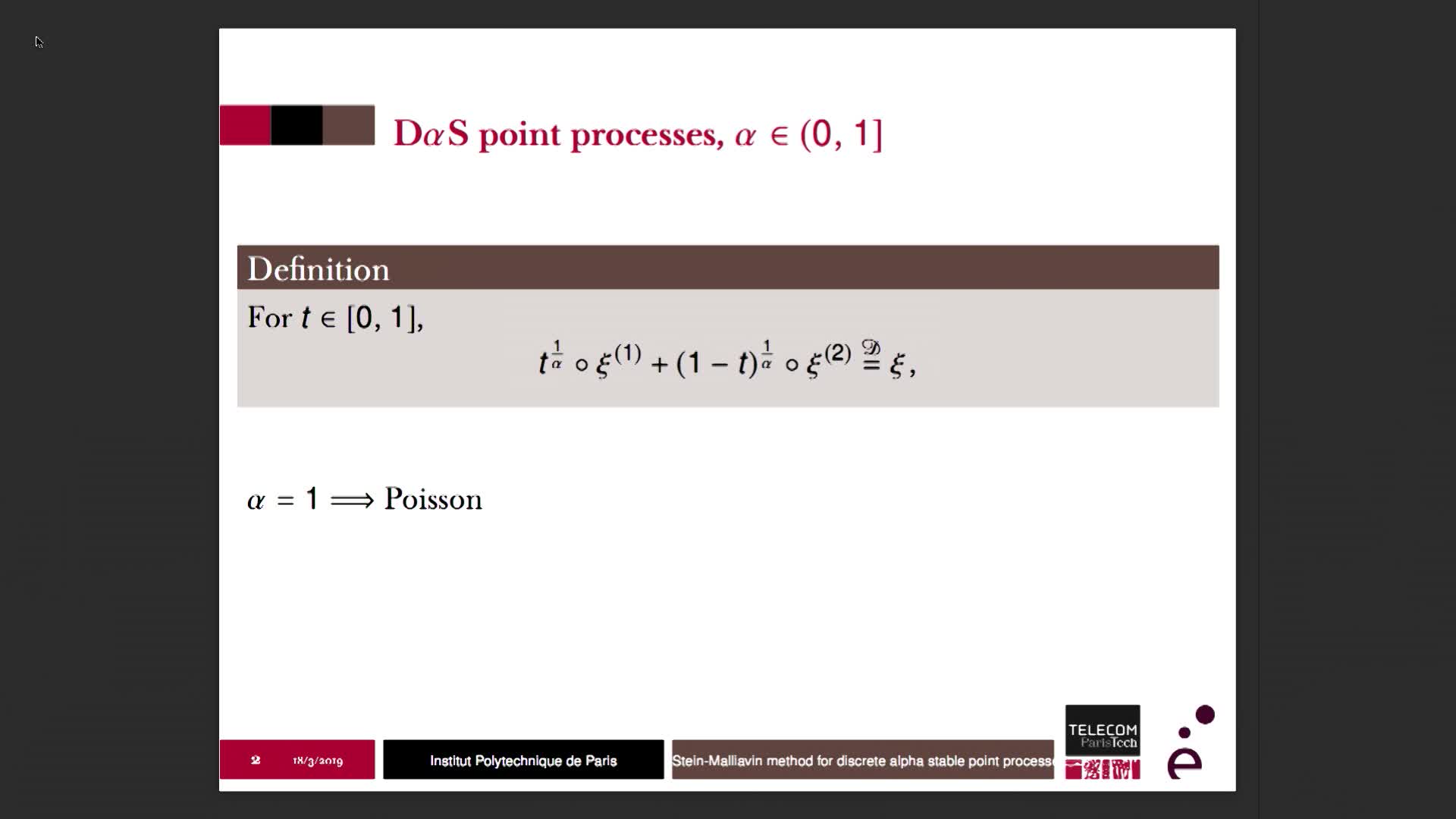
Stein-Malliavin method for discrete alpha stable point processes (workshop ERC Nemo Processus ponct…
DecreusefondLaurentThe notion of discrete alpha-stable point processes generalizes to point processes the notion of stable distribution. It has been introduced and studied by Davydov, Molchanov and Zuyev a few