Mondino, Andrea (1984-....)
Vidéos
A. Mondino - Time-like Ricci curvature bounds via optimal transport
The goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 1
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who
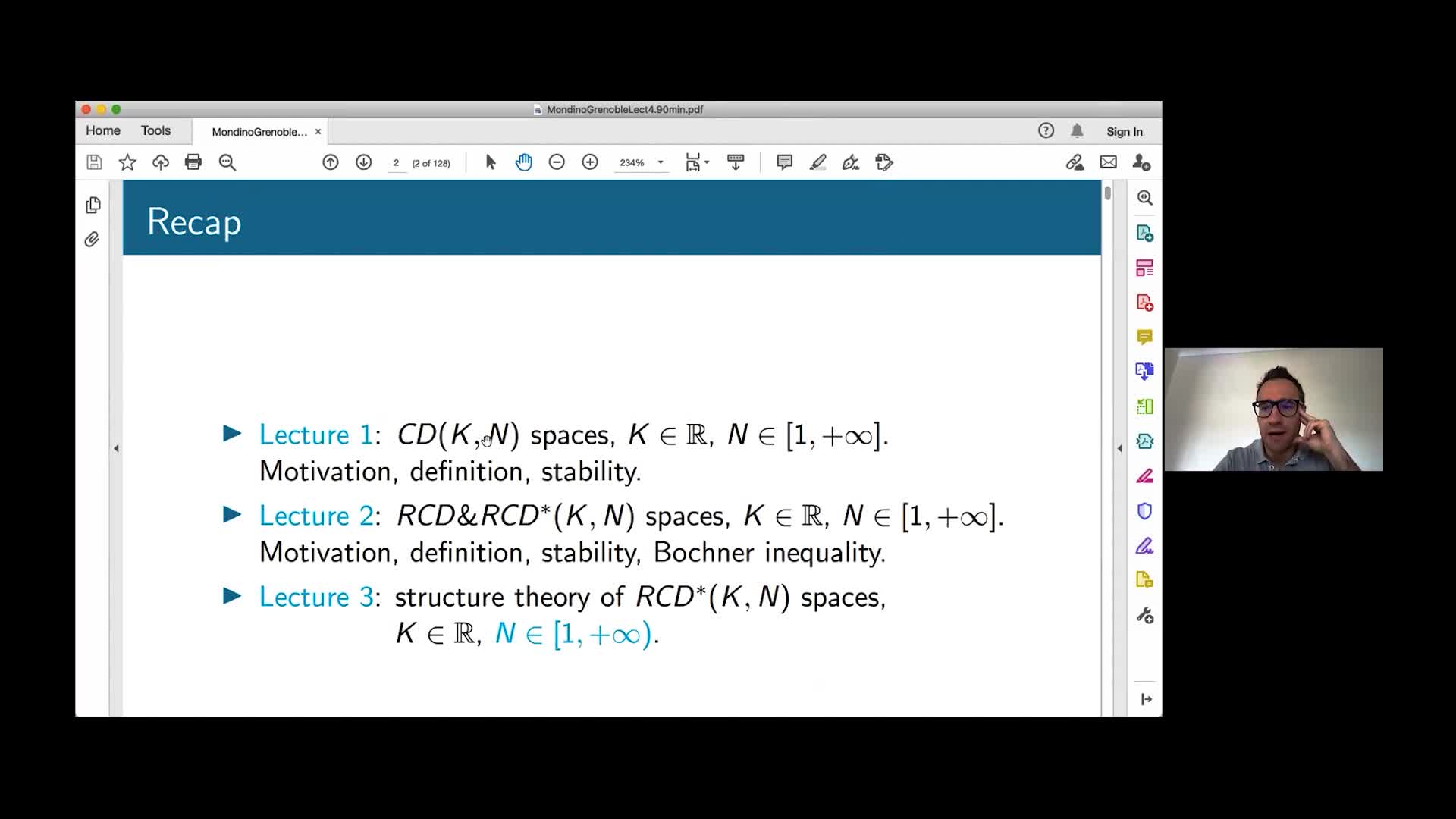
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 4
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 2
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 3
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who