Notice
A. Mondino - Time-like Ricci curvature bounds via optimal transport
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
The goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the celebrated Lott-Sturm-Villani theory of CD(K,N) metric measure spaces. The key idea being to analyse convexity properties of Entropy functionals along future directed timelike geodesics (with respect to a suitable Lorentzian Wasserstein distance) of probability measures. The smooth Lorentzian setting was previously investigated by McCann and Mondino-Suhr.
After recalling the general setting of Lorentzian synthetic spaces (including remarkable examples fitting the framework), I will discuss some basics of optimal transport theory thereof in order to define "timelike Ricci curvature bounded below and dimension bounded above'' for a (possibly non-smooth) Lorentzian space. The notion of "timelike Ricci curvature bounded below and dimension bounded above'' for a (possibly non-smooth) Lorentzian space is stable under a suitable weak convergence of Lorentzian synthetic spaces, giving a glimpse on the strength of the proposed approach.
As an application of the optimal transport approach to timelike Ricci curvature lower bounds, I will discuss an extension of the Hawking's Singularity Theorem (in sharp form) to the synthetic setting.
Thème
Documentation
Dans la même collection
-
J. Wang - Topological rigidity and positive scalar curvature
WANG Jian
In this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
J. Fine - Knots, minimal surfaces and J-holomorphic curves
FINE Joël
I will describe work in progress, parts of which are joint with Marcelo Alves. Let L be a knot or link in the 3-sphere. I will explain how one can count minimal surfaces in hyperbolic 4-space
-
D. Semola - Boundary regularity and stability under lower Ricci bounds
SEMOLA Daniele
The theory of non smooth spaces with lower Ricci Curvature bounds has undergone huge developments in the last thirty years. On the one hand the impetus came from Gromov’s precompactness theorem
-
D. Stern - Harmonic map methods in spectral geometry
STERN Daniel
Over the last fifty years, the problem of finding sharp upper bounds for area-normalized Laplacian eigenvalues on closed surfaces has attracted the attention of many geometers, due in part to
-
T. Ozuch - Noncollapsed degeneration and desingularization of Einstein 4-manifolds
OZUCH Tristan
We study the noncollapsed singularity formation of Einstein 4-manifolds. We prove that any smooth Einstein 4-manifold close to a singular one in a mere Gromov-Hausdorff (GH) sense is the result
-
Y. Lai - A family of 3d steady gradient Ricci solitons that are flying wings
LAI Yi
We find a family of 3d steady gradient Ricci solitons that are flying wings. This verifies a conjecture by Hamilton. For a 3d flying wing, we show that the scalar curvature does not vanish at
-
D. Tewodrose - Limits of Riemannian manifolds satisfying a uniform Kato condition
TEWODROSE David
Presentation of a joint work with G. Carron and I. Mondello where we study Kato limit spaces.
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BAMLER Richard H.
We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.
-
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass Theorem
LESOURD Martin
The study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in
-
C. Li - Classifying sufficiently connected PSC manifolds in 4 and 5 dimensions
LI Chao
In this talk, I will discuss some recent developments on the topology of closed manifolds admitting Riemannian metrics of positive scalar curvature. In particular, we will prove if a closed PSC
-
P. Burkhardt - Pointwise lower scalar curvature bounds for C0 metrics via regularizing Ricci flow
BURKHARDT-GUIM Paula
We propose a class of local definitions of weak lower scalar curvature bounds that is well defined for C0 metrics. We show the following: that our definitions are stable under greater-than-second
-
R. Perales - Recent Intrinsic Flat Convergence Theorems
PERALES Raquel
Théorèmes récents de convergence plane intrinsèque
Avec les mêmes intervenants et intervenantes
-
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 1
MONDINO Andrea
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who
-
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 4
MONDINO Andrea
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who
-
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 2
MONDINO Andrea
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who
-
A. Mondino - Metric measure spaces satisfying Ricci curvature lower bounds 3
MONDINO Andrea
The idea of compactifying the space of Riemannian manifolds satisfying Ricci curvature lower bounds goes back to Gromov in the '80ies and was pushed by Cheeger-Colding in the ‘90ies, who
Sur le même thème
-
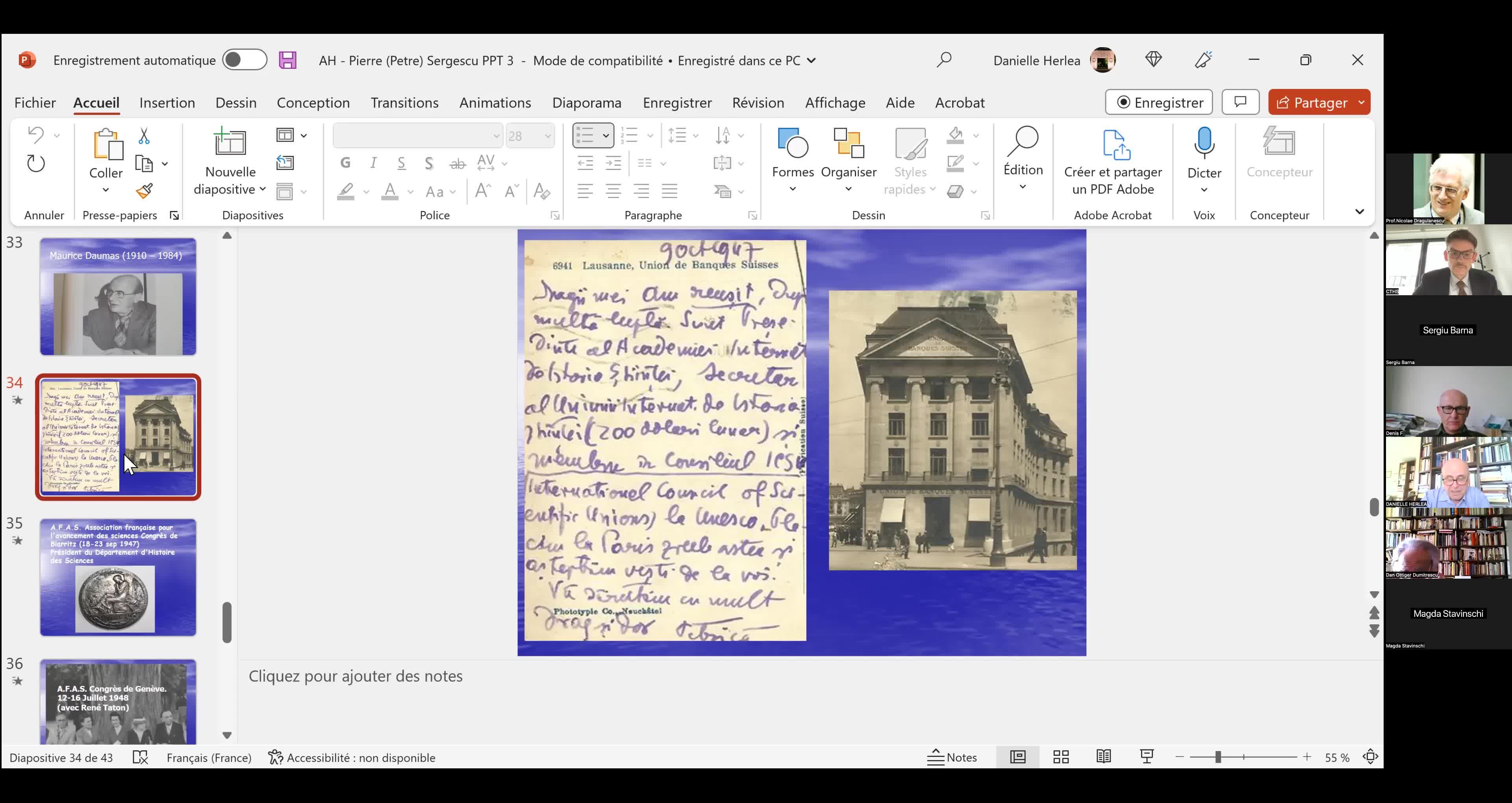
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HERLéA Alexandre
Alexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
Webinaire sur la rédaction des PGD
LOUVET Violaine
Rédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BAMLER Richard H.
We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.
-
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass Theorem
LESOURD Martin
The study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in
-
J. Wang - Topological rigidity and positive scalar curvature
WANG Jian
In this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
T. Ozuch - Noncollapsed degeneration and desingularization of Einstein 4-manifolds
OZUCH Tristan
We study the noncollapsed singularity formation of Einstein 4-manifolds. We prove that any smooth Einstein 4-manifold close to a singular one in a mere Gromov-Hausdorff (GH) sense is the result
-
J. Fine - Knots, minimal surfaces and J-holomorphic curves
FINE Joël
I will describe work in progress, parts of which are joint with Marcelo Alves. Let L be a knot or link in the 3-sphere. I will explain how one can count minimal surfaces in hyperbolic 4-space
-
D. Semola - Boundary regularity and stability under lower Ricci bounds
SEMOLA Daniele
The theory of non smooth spaces with lower Ricci Curvature bounds has undergone huge developments in the last thirty years. On the one hand the impetus came from Gromov’s precompactness theorem
-
D. Stern - Harmonic map methods in spectral geometry
STERN Daniel
Over the last fifty years, the problem of finding sharp upper bounds for area-normalized Laplacian eigenvalues on closed surfaces has attracted the attention of many geometers, due in part to
-
P. Burkhardt - Pointwise lower scalar curvature bounds for C0 metrics via regularizing Ricci flow
BURKHARDT-GUIM Paula
We propose a class of local definitions of weak lower scalar curvature bounds that is well defined for C0 metrics. We show the following: that our definitions are stable under greater-than-second
-
R. Perales - Recent Intrinsic Flat Convergence Theorems
PERALES Raquel
Théorèmes récents de convergence plane intrinsèque