Notice
Mathématiques savonneuses
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
Qui n'a pas été émerveillé par des bulles de savon ? A partir de quelques expériences simples et d'un peu de curiosité, nous allons nous plonger dans le monde des films de savon pour ressortir avec des surfaces minimales... après passage dans le monde mathématique. L'objectif sera de partir des questions naturelles qui se posent en regardant ces film de savon et de décrire la démarche (idéalisée) du mathématicien pour en tirer des questions mathématiques. Nous ne pourrons nous empêcher de décrire quelques outils nécessaires à leur résolution même si cela ne veut (et ne doit) pas être le sujet essentiel de l'exposé. Cet exposé se veut la forme épurée d'expériences multiples pratiquées en collège et lycée puisque les expérimentations sont tirées du projet "MathALyon", série de manipulations mathématiques qui s'installent dans les classes de collège et lycée accompagnées de chercheurs. Nous partirons donc de questions que des collégiens et lycéens peuvent (doivent ?) se poser en faisant ces expériences. Nous tenterons de montrer comment les accompagner pour "mathématiser" ces interrogations. Nous irons sans doute un tout petit peu plus loin qu'avec les élèves puisque nous aurons un peu plus de temps....
Dans la même collection
-
Ressources numériques, pratiques enseignantes et dynamique de la salle de classe.
SACRISTAN Ana Isabel
On discute les usages des ressources numériques dans l’enseignement et apprentissage des mathématiques et l’importance d’un modèle pédagogique pour pouvoir mieux profiter du potentiel de ces
-
Quelles maths faut-il apprendre pour répondre aux questions des élèves et traiter leurs erreurs ?
MERCIER Alain
Les travaux sur les erreurs des élèves ont montré que leur forme est constante, indépendante des enseignements, et qu'elles apparaissent longtemps encore après les commencements, de manière quasi
-
Ma thématique, la didactique, l'informatique aussi
Les mathématiques sont, pour la didactique de cette discipline, un objet d'étude sous les contraintes particulières des problématiques de l'apprentissage et de l'enseignement. Elles peuvent aussi
Sur le même thème
-
Phong NGUYEN - Recent progress on lattices's computations 2
NGUYEN Phong Q.
This is an introduction to the mysterious world of lattice algorithms, which have found many applications in computer science, notably in cryptography. We will explain how lattices are represented by
-
Aurel PAGE - Cohomology of arithmetic groups and number theory: geometric, asymptotic and computati…
PAGE Aurel regis
In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)
-
Tobias Moede - Coclass theory for nilpotent associative algebras
The coclass of a finite p-group of order p^n and class c is defined as n-c. Using coclass as the primary invariant in the investigation of finite p-groups turned out to be a very fruitful approach.
-
Oussama Hamza - Hilbert series and mild groups
Let $p$ be an odd prime number and $G$ a finitely generated pro-$p$ group. Define $I(G)$ the augmentation ideal of the group algebra of $G$ over $F_p$ and define the Hilbert series of $G$ by: $G(t):
-
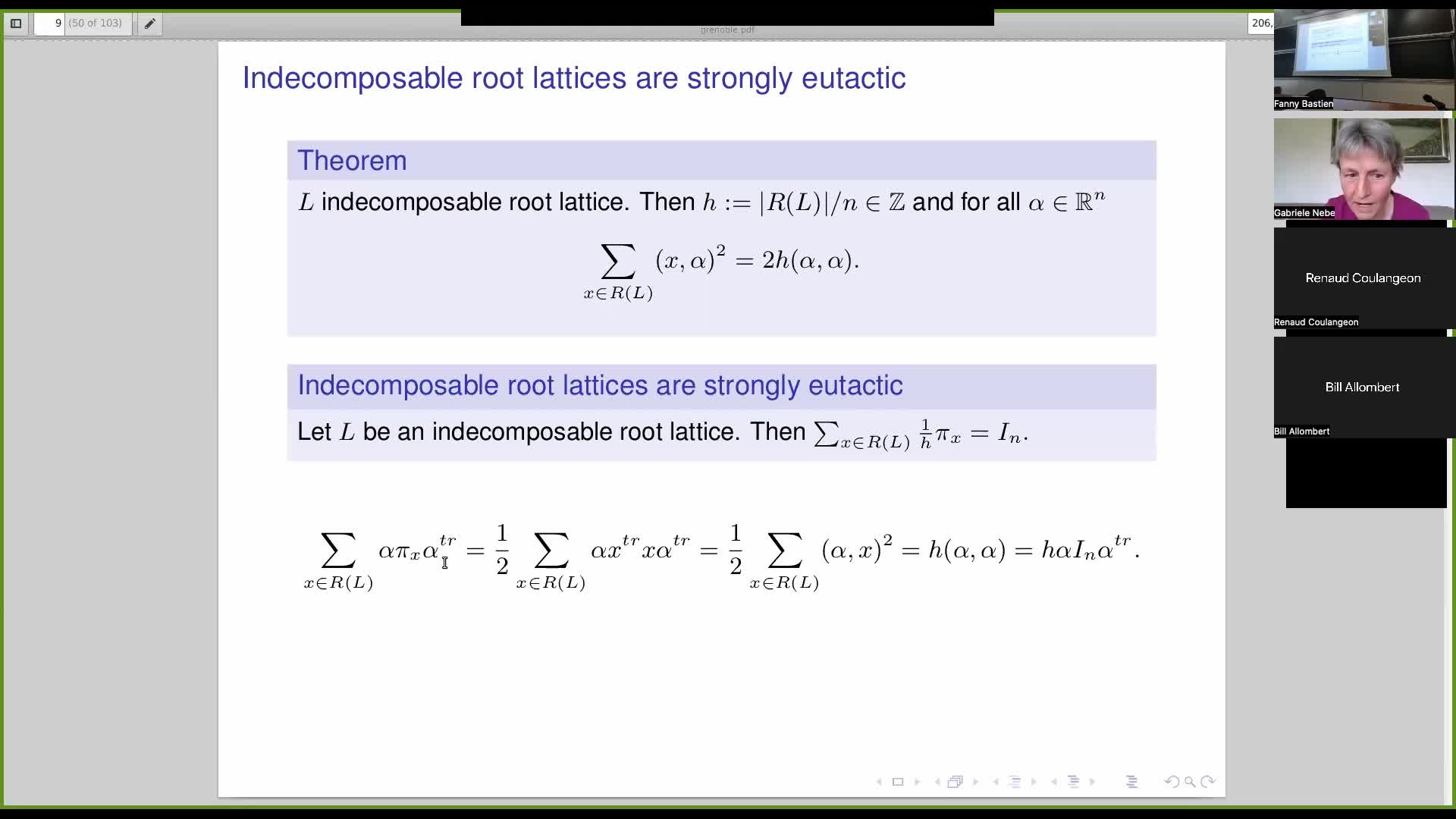
Gabriele NEBE - Lattices, Perfects lattices, Voronoi reduction theory, modular forms, computations …
NEBE Gabriele
The talks of Coulangeon will introduce the notion of perfect, eutactic and extreme lattices and the Voronoi's algorithm to enumerate perfect lattices (both Eulcidean and Hermitian). The talk of Nebe
-
Alexander HULPKE - Computational group theory, cohomology of groups and topological methods 4
The lecture series will give an introduction to the computer algebra system GAP, focussing on calculations involving cohomology. We will describe the mathematics underlying the algorithms, and how to
-
Alexander HULPKE - Computational group theory, cohomology of groups and topological methods 5
The lecture series will give an introduction to the computer algebra system GAP, focussing on calculations involving cohomology. We will describe the mathematics underlying the algorithms, and how to
-
Zachary Himes - On not the rational dualizing module for $\text{Aut}(F_n)$
Bestvina--Feighn proved that $\text{Aut}(F_n)$ is a rational duality group, i.e. there is a $\mathbb{Q}[\text{Aut}(F_n)]$-module, called the rational dualizing module, and a form of Poincar\'e duality
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
Paul GUNNELLS - Cohomology of arithmetic groups and number theory: geometric, asymptotic and comput…
GUNNELLS Paul E.
In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)
-
Paul GUNNELLS - Cohomology of arithmetic groups and number theory: geometric, asymptotic and comput…
GUNNELLS Paul E.
In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)
-
Paul GUNNELLS - Cohomology of arithmetic groups and number theory: geometric, asymptotic and comput…
GUNNELLS Paul E.
In this lecture series, the first part will be dedicated to cohomology of arithmetic groups of lower ranks (e.g., Bianchi groups), their associated geometric models (mainly from hyperbolic geometry)