DYOGENE/ERC NEMO 2022 : Seminar series
Descriptif
Usually held on Monday, the DYOGENE/ERC NEMO Seminar Series invites researchers to present their work on stochastic processes, random graphs and point processes. Here is the list of the talks from 2021 till now.
Vidéos
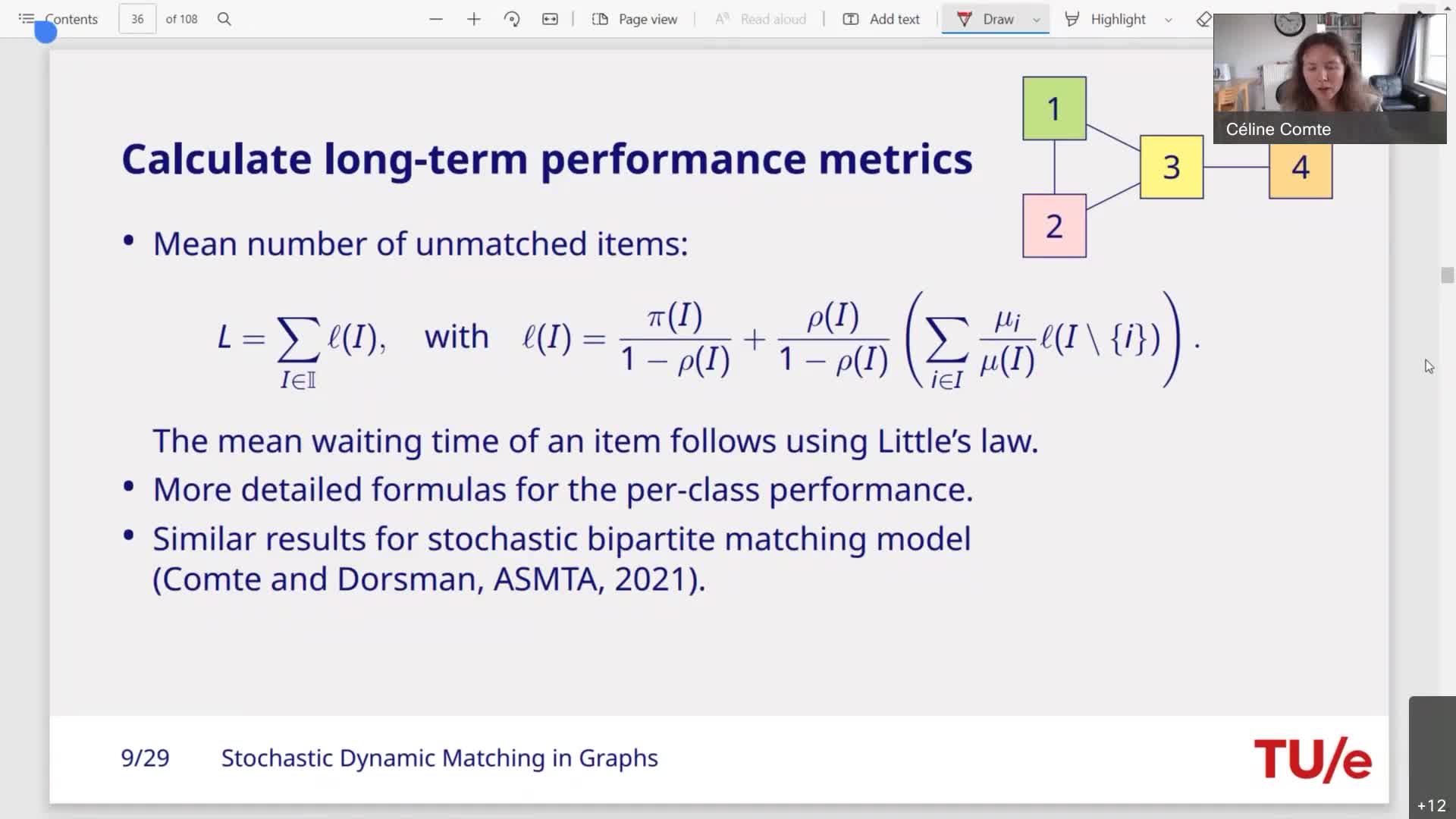
Stochastic Dynamic Matching in Graphs
We will consider a stochastic dynamic matching model, in which items of different classes arrive according to independent Poisson processes...
The geometry of random contours and its impact on the correlations
I will talk about the models of statistical physics which can be expressed as collections of random contours (such as the Ising model).
On the nearest neighbor tree
Let points X_1,X_2, … be i.d.d. uniform over [0,1]^d. When a new point X_n arrives, it connects to the nearest point in X1,…,X_{n-1}.
The cost of point processes
Every invariant point process on a topological group has a numerical invariant associated to it...
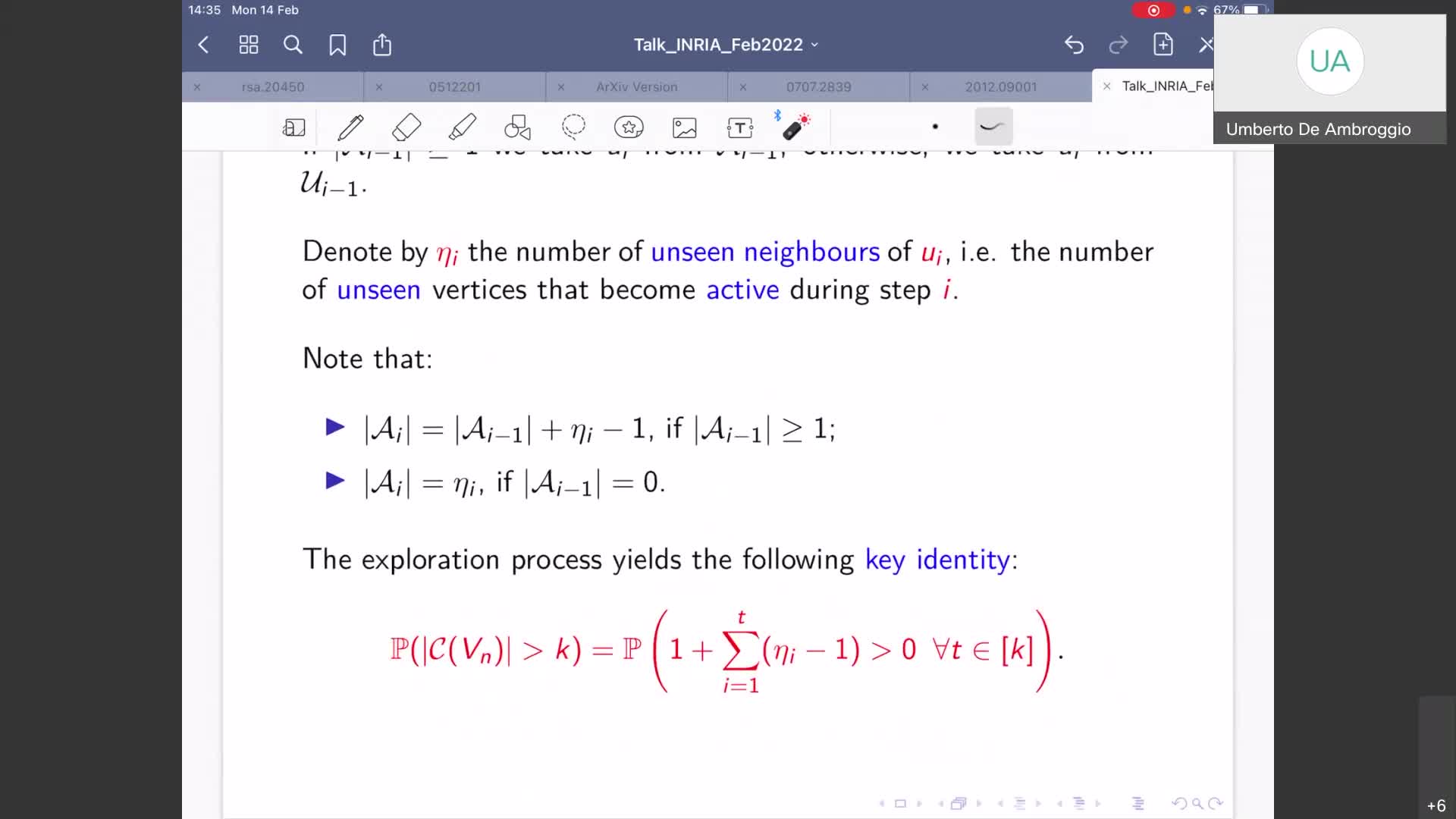
The probability of unusually large components in critical random graphs via ballot theorems
We consider the classical Erdős-Rényi random graph as well as percolation on a random regular graph...
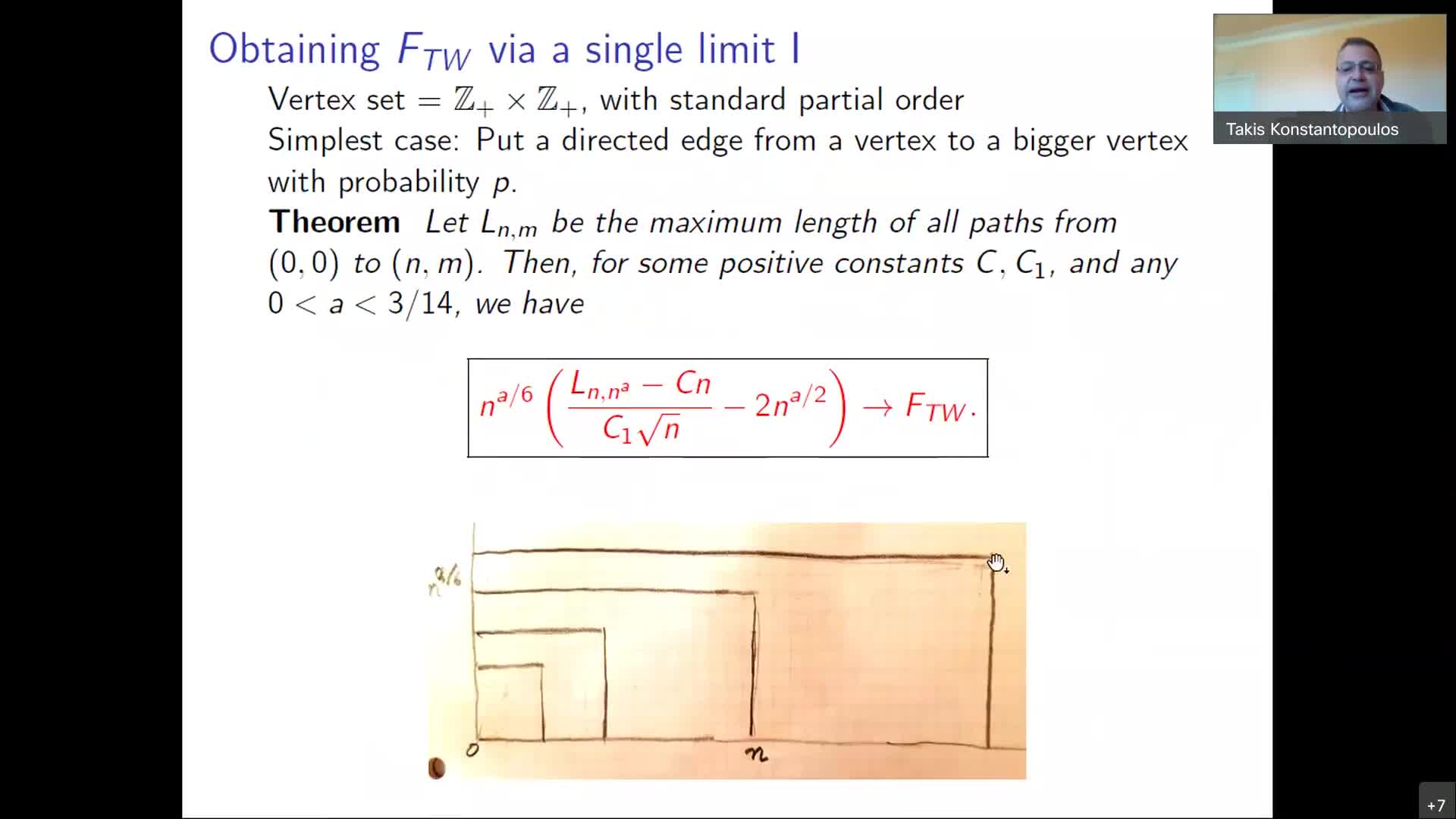
Longest and heaviest paths in random directed graphs
I will give an overview of research in the area of random directed graphs with possibly random edge weights.
Spectral properties and applications of Kirchhoff's forest
Random spanning forests, which are efficiently sampled by Wilson's algorithm, enjoy both abelian...
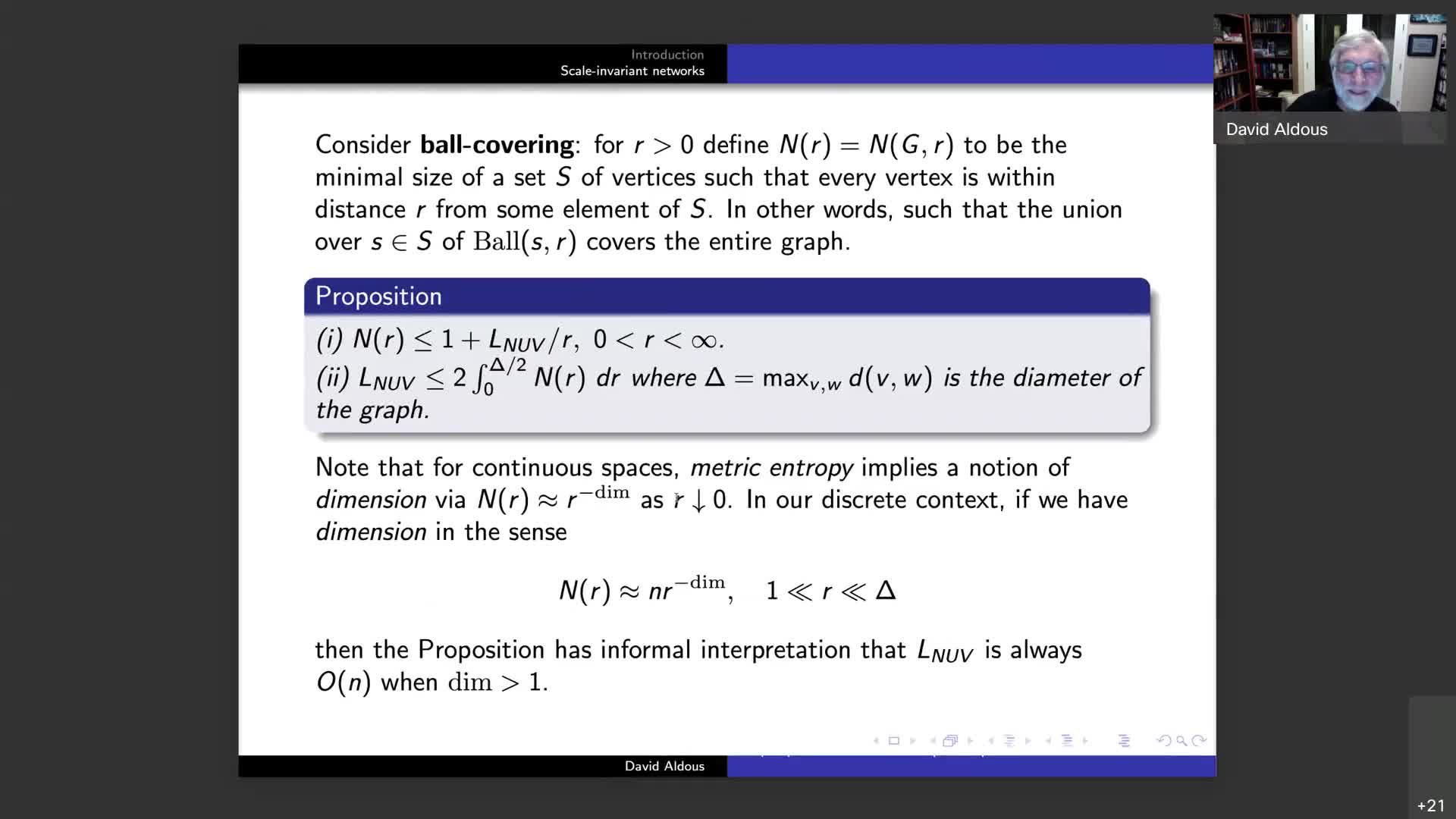
Open problems within three topics in spatial networks: scale-invariance, the nearest unvisited vert…
(1) Some of my old work involved an axiomatization of the SIRSN class of networks...
Perfect simulation of interacting point processes with infinite interaction range
I will present a (partly) new method for perfectly sampling from the stationary regime of point processes having an infinite number of interacting components.
Forward and backward limits
We start by considering irreducible aperiodic positive recurrent Markov chains, and the proof of the main limit theorem...
Structural properties of a conditioned random walk on the integer lattice with local constraints
We consider a random walk on a one/multidimensional integer lattice with random bounds on local times, conditioned on the event that it hits a high level before its death.
The unreasonable effectiveness of determinantal processes
In 1960, Wigner published an article famously titled “The Unreasonable Effectiveness of Mathematics in the Natural Sciences.
Fluctuations of random convex interfaces
We consider the convex hull of a point set constituted with independent and uniformly distributed points in a smooth convex body K of R^d.
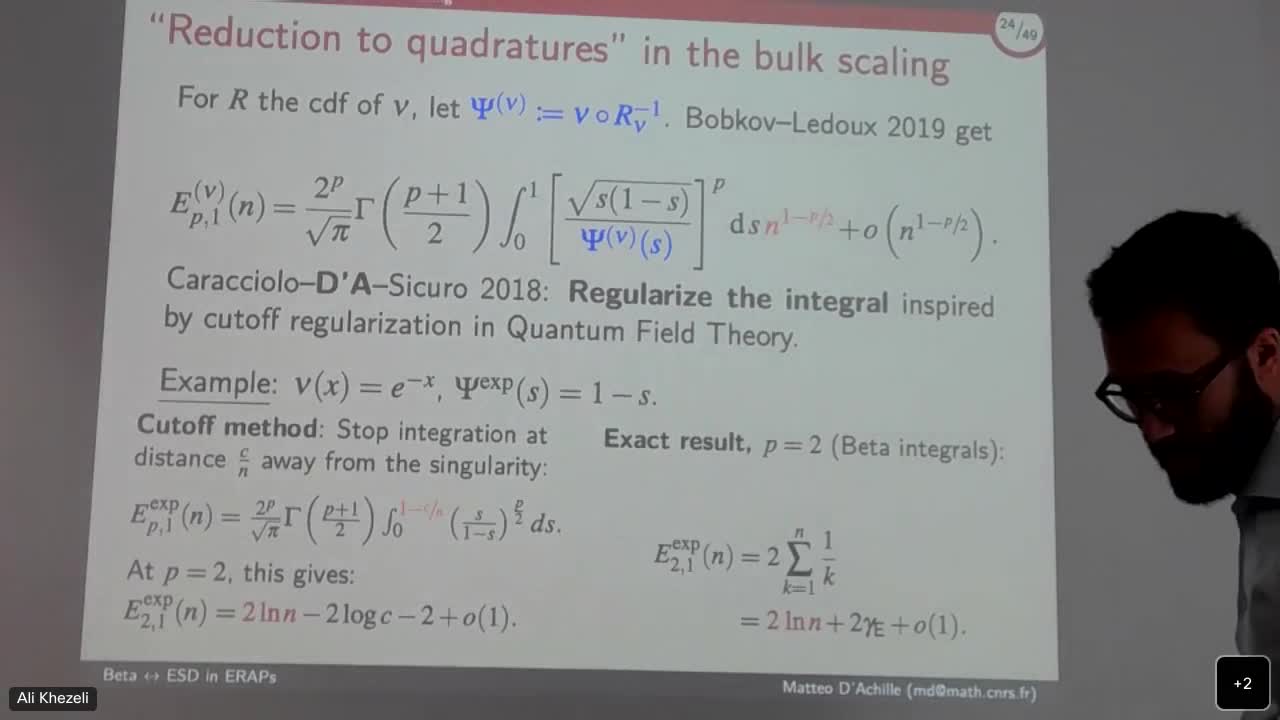
Back and forth between the beta distribution and edge stochastic domination in ERAPs
I will survey recent and less recent results on the phase diagram of Euclidean Random Assignment Problems (ERAPs)...
Random Tessellation Forests
Random forests are a popular class of algorithms used for regression and classification.
Sofic entropy of processes on infinite random trees
This is a joint work with Agnes Backhausz et Balasz Szegedy. We define a natural notion of micro-state entropy...
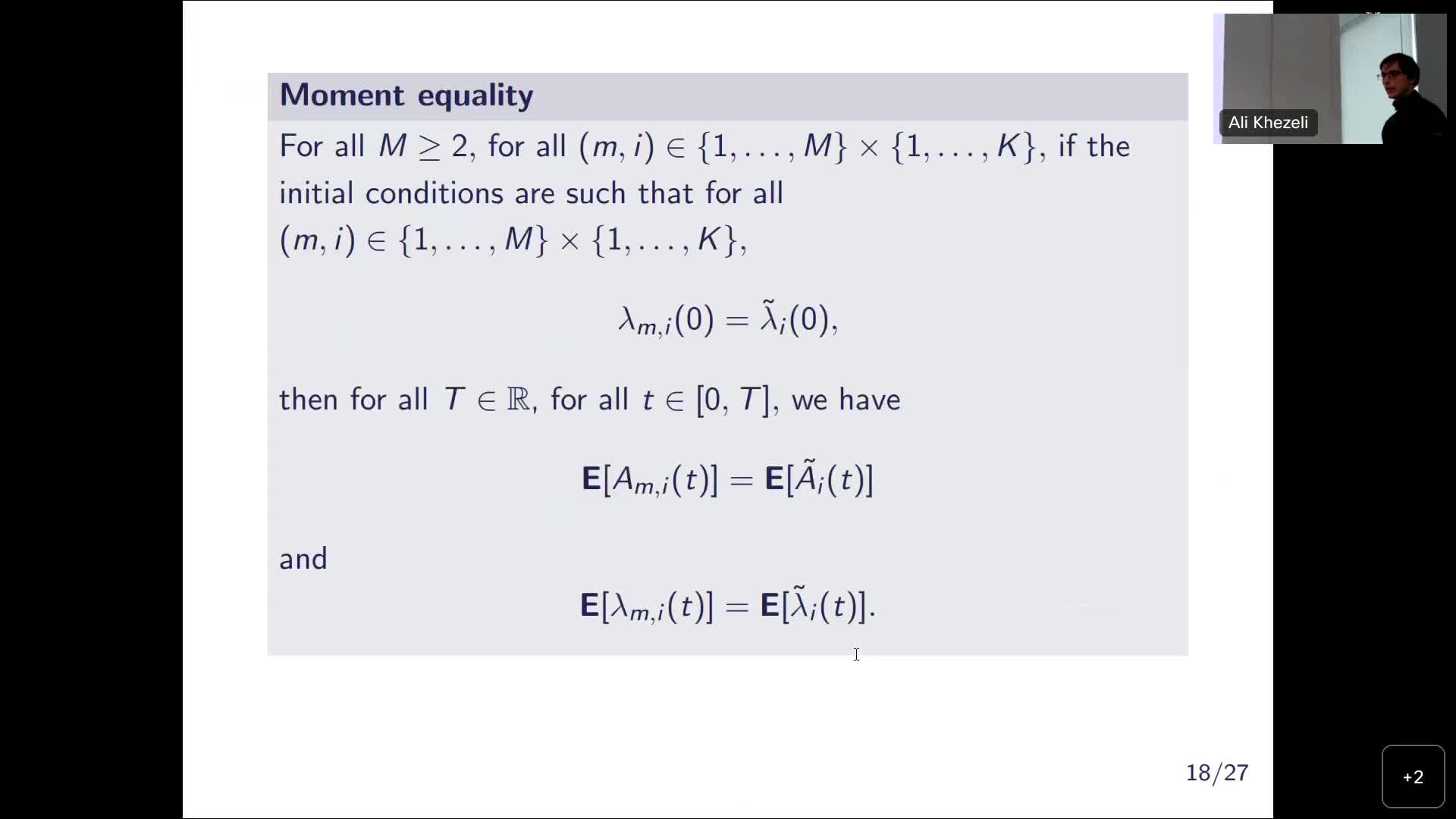
Propagation of chaos and Poisson Hypothesis for replica mean-field models of intensity-based neural…
Neural computations arising from myriads of interactions between spiking neurons can be modeled as network dynamics with punctuate interactions.
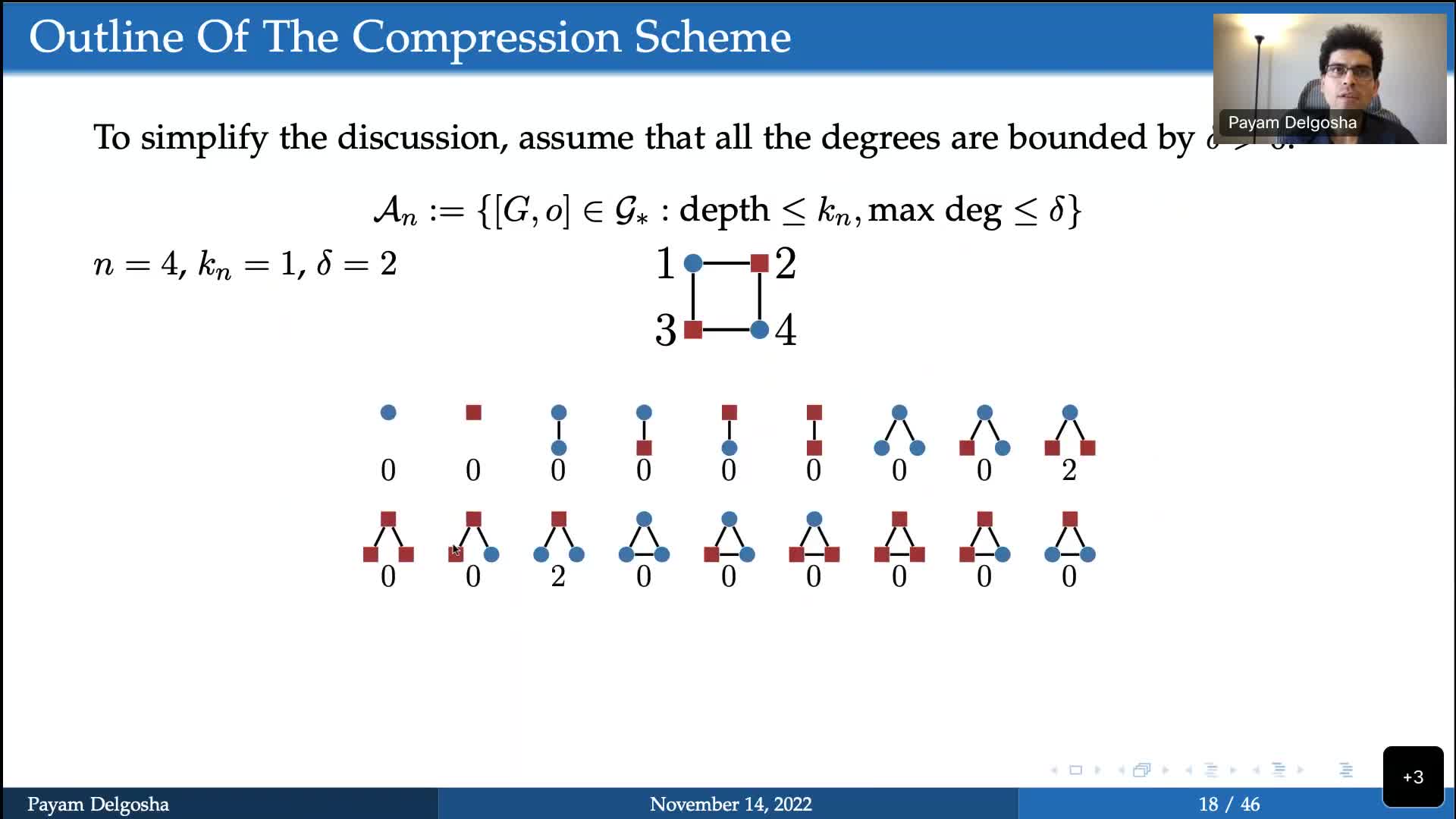
A Notion of Entropy for Sparse Marked Graphs and its Applications in Graphical Data Compression
Many modern data sources arising from social networks, biological data, etc. are best viewed...
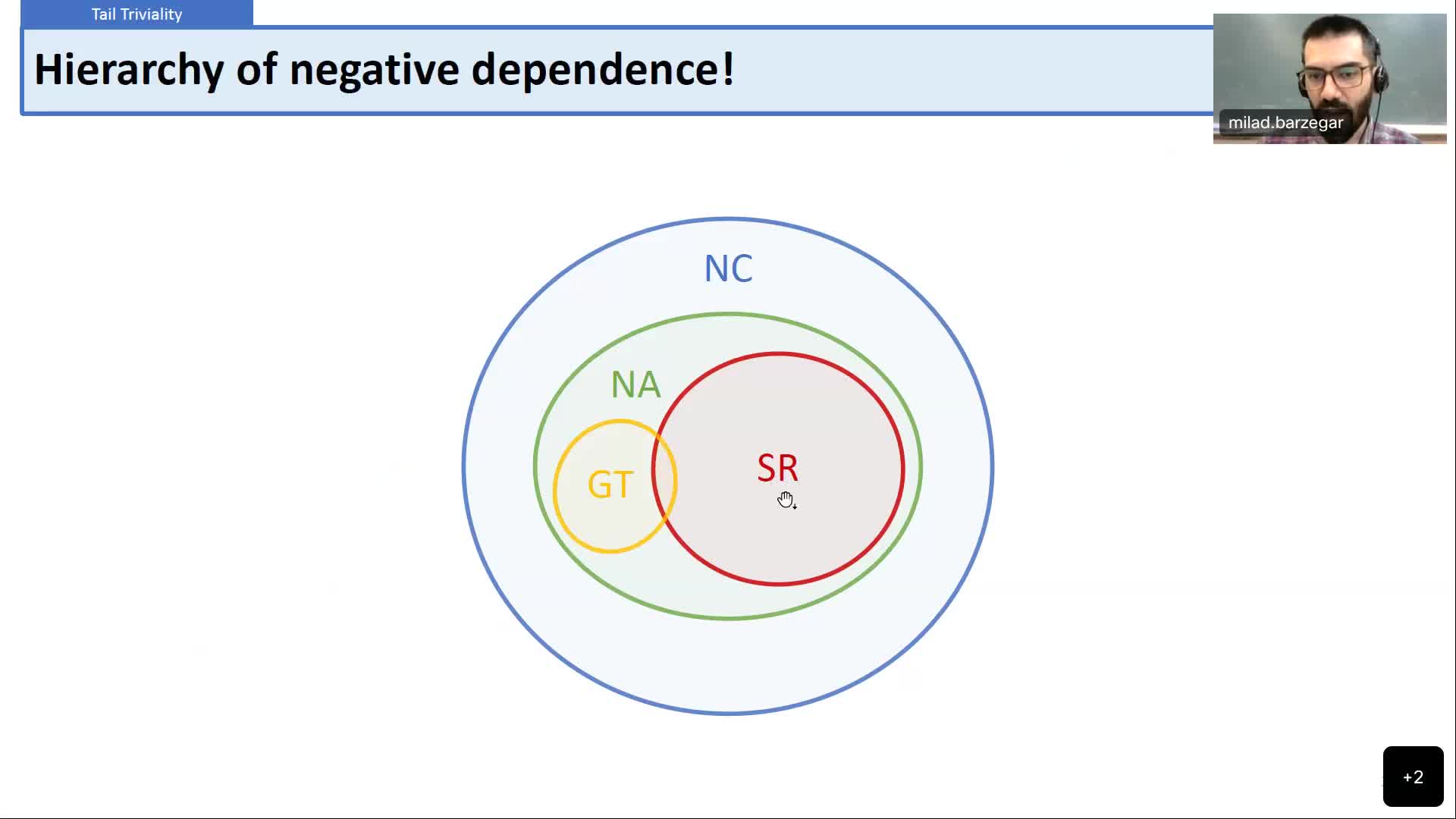
On the dependence structure of negatively dependent measures
A major breakthrough in the theory of negatively dependent – i.e., repulsive – probability measure...
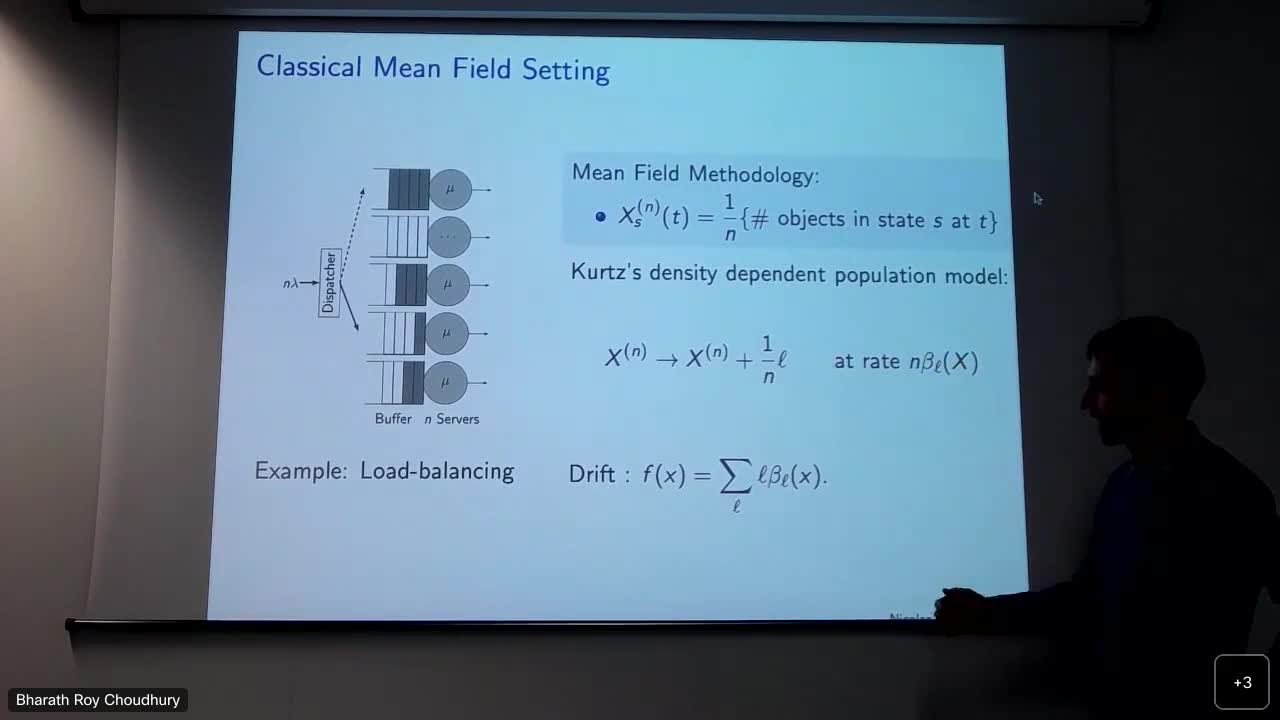
The bias of fluid approximation: Poisson equation, averaging methods and two-timescale processes
Fluid approximation often provide a good tool to study a stochastic process.
Intervenants et intervenantes
Ph. D. (Université de Cambridge, 1977)
Mathématicien. En poste au Department of statistics, University of California, Berkeley, California, USA (en 1985)
Doctorant en ingénierie géophysique et minière. Boursier de la School of Engineering, Deakin University, Geelong (Australie, 2023)
Mathématicien. Chargé de Recherche CNRS à l'Institut de Mathématiques de Marseille (en 2020). Chargé de Recherche CNRS à l'Institut de Mathématiques de Toulouse (en 2018). Auteur d'une thèse en Mathématiques appliquées à l'Ecole Polytechnique, Palaiseau (Essonne).
Professeur des universités en mathématiques et membre de l'unité de recherche "Laboratoire de mathématiques Raphaël Salem" - LMRS - à l'Université de Rouen Normandie (en 2022)
Rapporteur et membre du jury d'une thèse en Mathématiques à Université Côte d'Azur en 2024
Professeur, Université de Rouen Normandie, LMRS en 2017
Titulaire d'un doctorat en Mathématiques (Lyon 1, 2002)
Chargée de recherche au CNRS dans l'équipe SARA au LAAS et dans l'équipe SOLACE à Toulouse. (en 2023). Chercheuse membre du groupe Stochastic Operations Research de l'Université Technologique d'Eindhoven (2019-2022). Auteure d'une thèse en informatiques (en 2019).
Professeur, Équipe Probabilités et Statistique à l'université Paris Saclay. Membre de laboratoire de mathématiques d'Orsay (en 2023). Auteur d'une thèse de : Mathématiques, Université Paris-Sud 11, Orsay (en 2011)
Stagiaire postdoctoral au Laboratoire de mathématiques d’Orsay (LMO), Université Paris-Saclay (en 2023)
Doctorant à l'INRIA Paris/École Normale Supérieure (Paris).
Mathématicien. Post-doctorant à l'University of Bath (en 2023)
Chercheur et enseignant à l’université d'Illinois (en 2023).
Mathématicien. Professeur, department of Actuarial mathematics & statistics, School of mathematical & computer sciences, Heriot-Watt university, Edimbourg, GB. (2024)
Doctorat en mathématiques (Novossibirsk, Russie, Institut Matematiki Imeni S. L. Soboleva, 1982)
Chargé de recherches INRIA, laboratoire LIG (2019). Directeur de thèse en Mathématiques appliquées à l'université Grenoble Alpes (2018). Auteur d'une thèse de doctorat en informatique (Grenoble, 2010).
Membre du jury d'une thèse en Informatique à Université Côte d'Azur en 2024
Mathématicien. Chargé de recherche CNRS (depuis 2009). Auteur d'une thèse en co-tutelle de : Mathématiques, Université Paris-Sud 11, Orsay et Università di Roma Tor Vergata, Italie (en 2006)
Chercheur au département de statistiques et de sciences de données de la National University of Singapore (en 2023)
Mathématicien. Professeur à l'université de Liverpool (Royaume-Uni) (2022)
Mathématicienne, en poste au Centre de mathématiques, Faculté de sciences et technologies, Université Paris XII, Créteil (en 2002)
Professeure à l'Université Paris 1 Panthéon-Sorbonne, chercheuse au sein de l'équipe SAMM, Statistique, Analyse et Modélisation Multidisciplinaire (en 2022)
Mathématicien. Professeur assistant à la Jagiellonian University (en 2023). A été post-doctorant à la McGill University et à l'École Normale Supérieure de Lyon. Docteur en mathématiques de la Central European University.
Professeure assistante au département de mathématiques appliquées et de statistiques de la Johns Hopkins University (en 2023)
Professeur associé au département de mathématiques et de statistiques de la Heriot-Watt University, Edinburgh (en 2023)
Mathématicien. Professeur à l'Institut de sciences, Reykjavik (en 2000)
Mathématicien