Notice
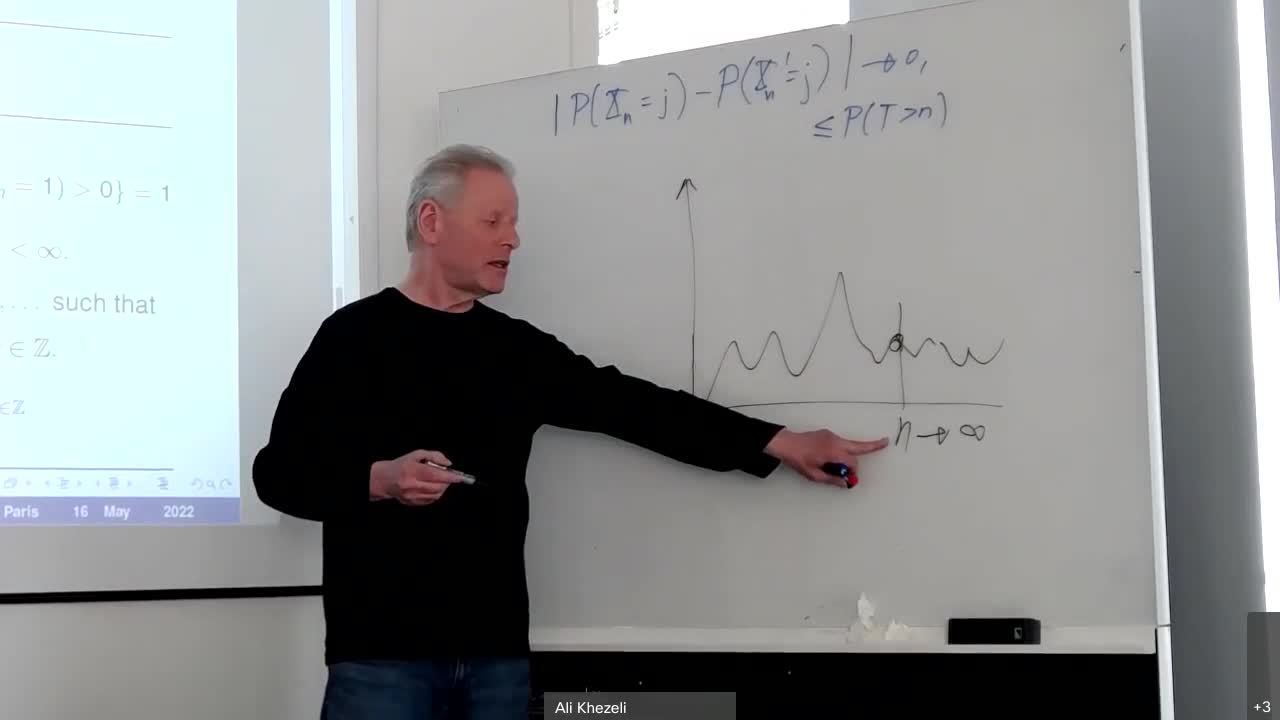
The probability of unusually large components in critical random graphs via ballot theorems
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
We consider the classical Erdős-Rényi random graph as well as percolation on a random regular graph and provide matching upper and lower bounds for the probability of observing unusually large maximal components in these models when considered near criticality. We sketch the proof for the upper bounds, which is based on a ballot-type estimate (that could be of independent interest) for the probability that a random walk stays positive for n steps and finishes at some level j. We also present a simple, yet general result which yields polynomial upper bounds for the probability of observing large components in several models of random graphs when considered at criticality.
Thème
Dans la même collection
-
Sofic entropy of processes on infinite random trees
BORDENAVE Charles
This is a joint work with Agnes Backhausz et Balasz Szegedy. We define a natural notion of micro-state entropy...
-
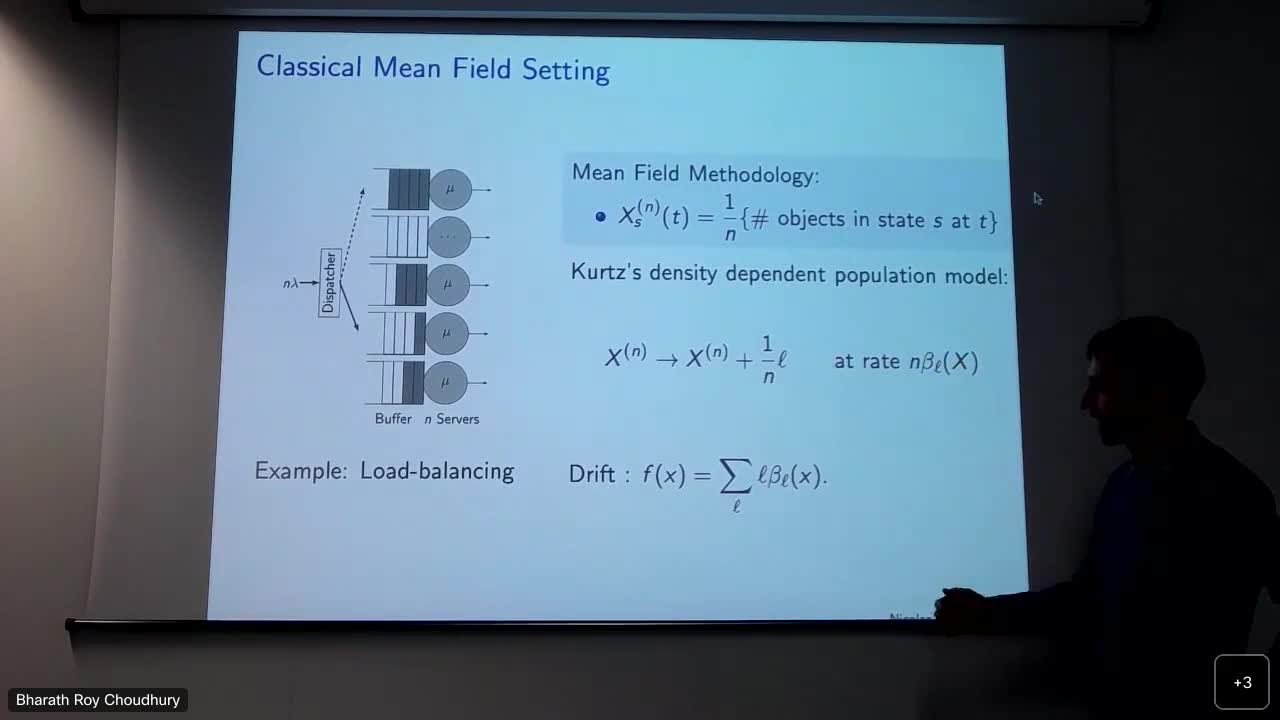
The bias of fluid approximation: Poisson equation, averaging methods and two-timescale processes
GAST Nicolas
Fluid approximation often provide a good tool to study a stochastic process.
-
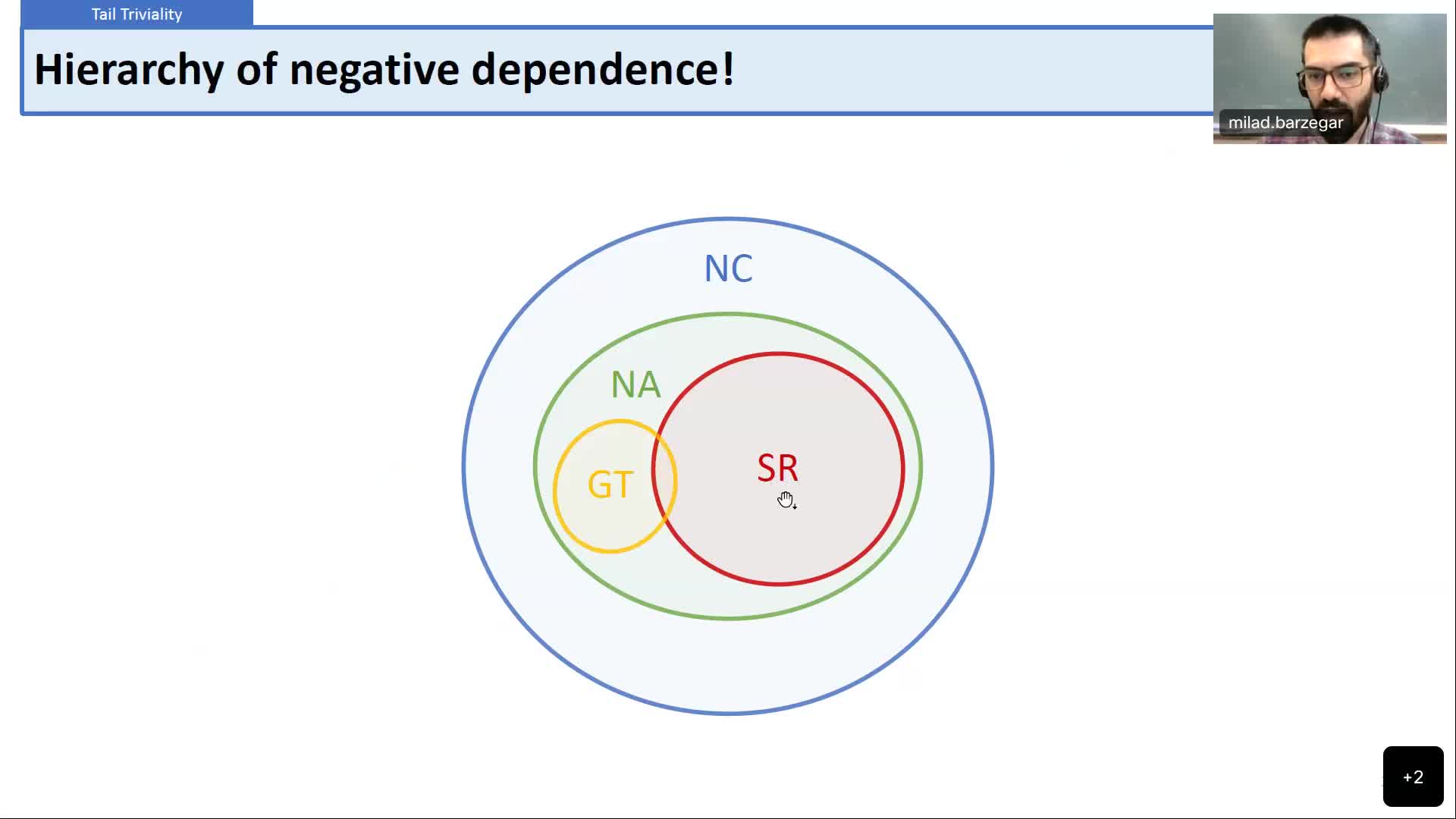
On the dependence structure of negatively dependent measures
BARZEGAR TOUCHANI Milad
A major breakthrough in the theory of negatively dependent – i.e., repulsive – probability measure...
-
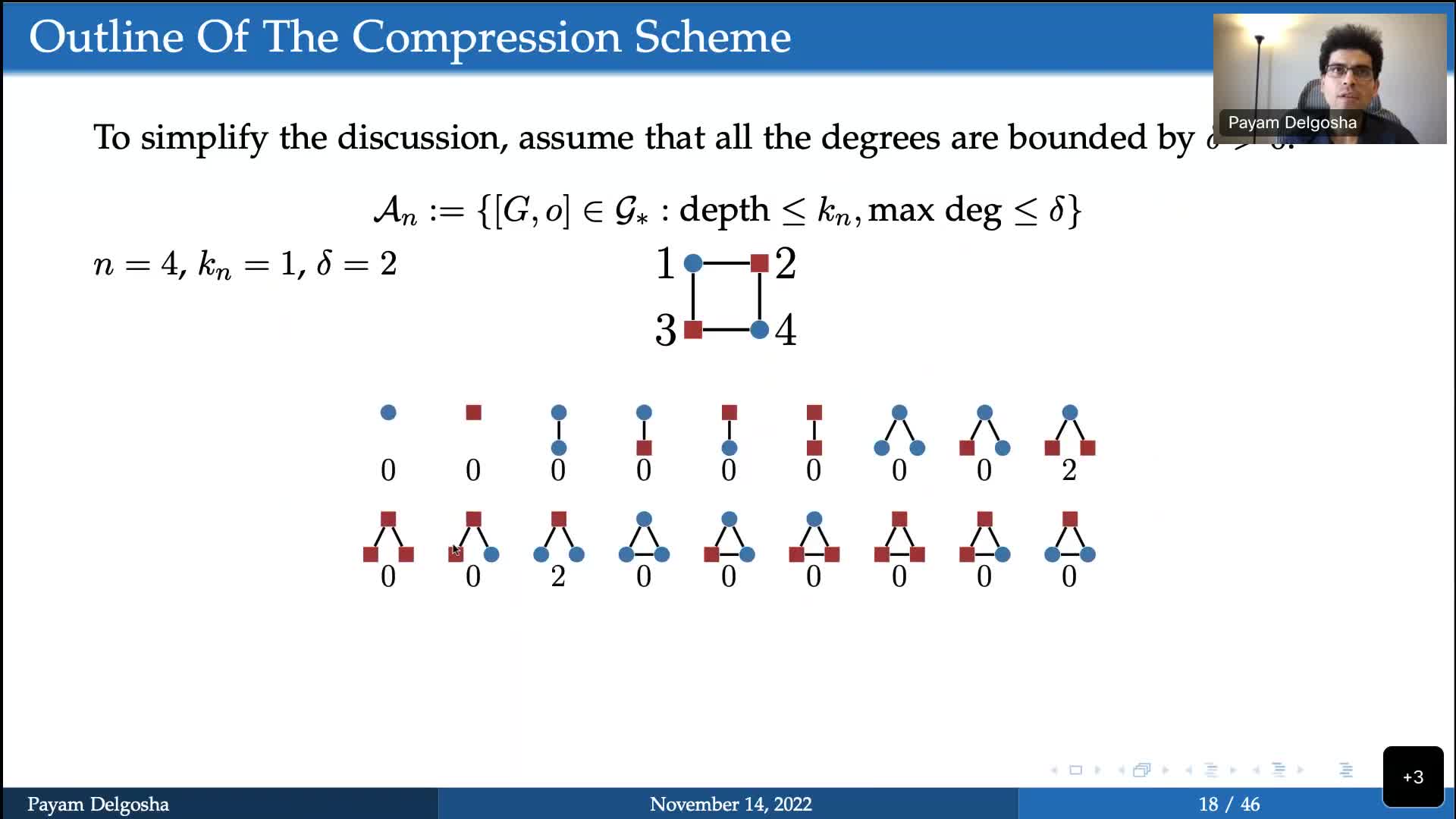
A Notion of Entropy for Sparse Marked Graphs and its Applications in Graphical Data Compression
DELGOSHA Payam
Many modern data sources arising from social networks, biological data, etc. are best viewed...
-
Propagation of chaos and Poisson Hypothesis for replica mean-field models of intensity-based neural…
DAVYDOV Michel
Neural computations arising from myriads of interactions between spiking neurons can be modeled as network dynamics with punctuate interactions.
-
Random Tessellation Forests
O'REILLY Eliza
Random forests are a popular class of algorithms used for regression and classification.
-
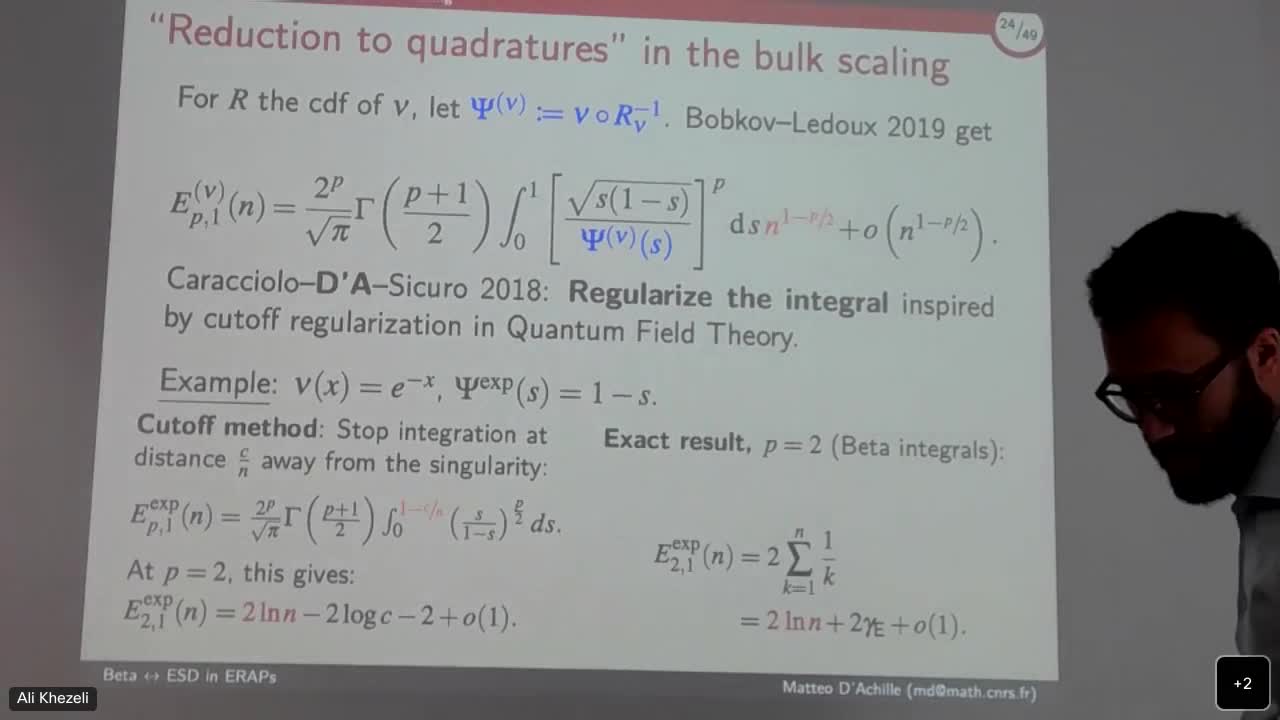
Back and forth between the beta distribution and edge stochastic domination in ERAPs
D'ACHILLE Matteo
I will survey recent and less recent results on the phase diagram of Euclidean Random Assignment Problems (ERAPs)...
-
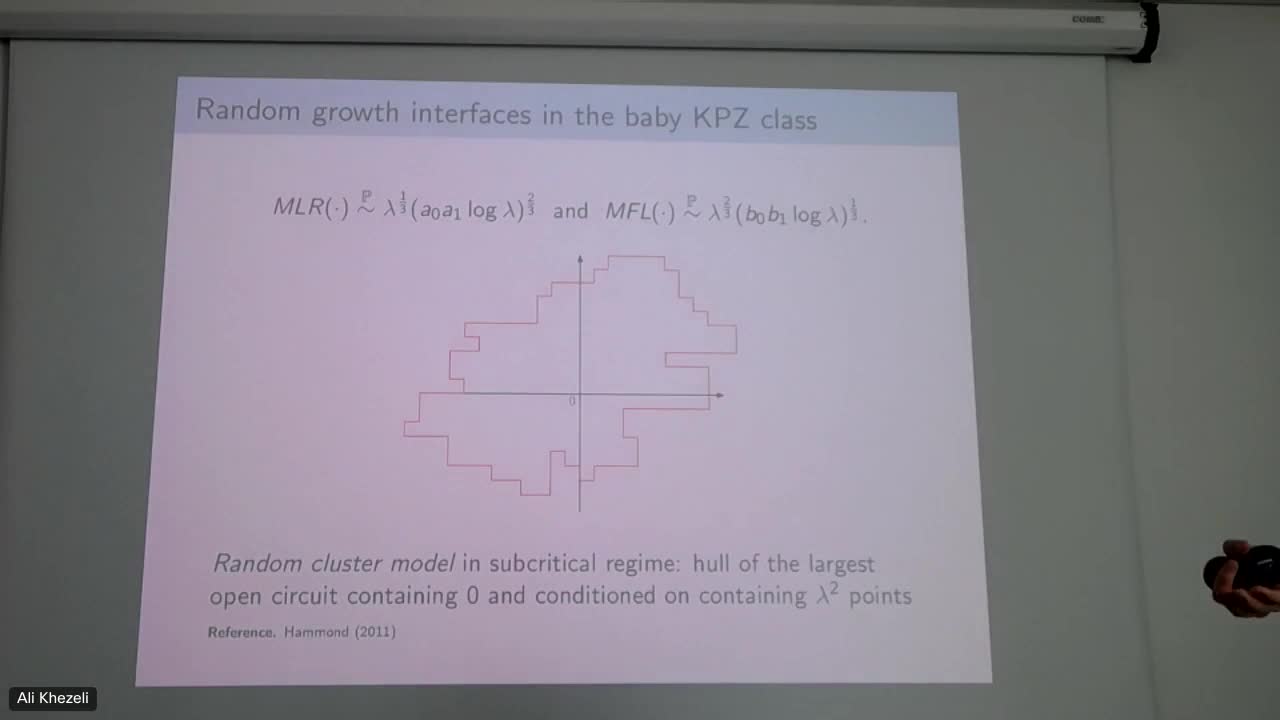
Fluctuations of random convex interfaces
CALKA Pierre
We consider the convex hull of a point set constituted with independent and uniformly distributed points in a smooth convex body K of R^d.
-
The unreasonable effectiveness of determinantal processes
GHOSH Subhroshekhar
In 1960, Wigner published an article famously titled “The Unreasonable Effectiveness of Mathematics in the Natural Sciences.
-
Structural properties of a conditioned random walk on the integer lattice with local constraints
FOSS Serguei
We consider a random walk on a one/multidimensional integer lattice with random bounds on local times, conditioned on the event that it hits a high level before its death.
-
Forward and backward limits
THORISSON Hermann
We start by considering irreducible aperiodic positive recurrent Markov chains, and the proof of the main limit theorem...
-
Sur le même thème
-
Bruit, erreur, anomalie et incertitude dans les données-PUDD
ROSSI Fabrice
Les données collectées sont systématiquement soumises à des perturbations de diverses natures, depuis le bruit de mesure de capteurs jusqu’aux erreurs de saisie.
-
Combinatorial maps in high genus
LOUF Baptiste
Combinatorial maps are a model of discrete geometry: they are surfaces made by gluing polygons along their sides, or equivalently, graphs drawn on surfaces. In this talk, I'll focus on the study of
-
Do there exist expanders with non-negative curvature ?
SALEZ Justin
In this talk I will briefly recall the framework of local weak limits of finite graphs introduced by I. Benjamini and O. Schramm
-
Tail bounds for detection times in mobile hyperbolic graphs
MITSCHE Dieter
Motivated by Krioukov et al.'s model of random hyperbolic graphs for real-world networks, and inspired by the analysis of a dynamic model of graphs in Euclidean space by Peres et al., we introduce a
-
Sofic entropy of processes on infinite random trees
BORDENAVE Charles
This is a joint work with Agnes Backhausz et Balasz Szegedy. We define a natural notion of micro-state entropy...
-
Online matching for the multiclass Stochastic Block Model
SOPRANO LOTO Nahuel
A matching in a graph is a set of edges that do not share endpoints. Developing algorithms that find large matchings is an important problem. An algorithm is said to be online if it has to construct
-
Critical cluster cascades
KIRCHNER Matthias
We consider a sequence of Poisson cluster point processes...
-
Point processes on higher rank symmetric spaces and their cost
MELLICK Samuel
Cost is a natural invariant associated to group actions and invariant point processes on symmetric spaces (such as Euclidean space and hyperbolic space). Informally, it measures how difficult it is to
-
Parallel server systems in extended heavy traffic
CASTIEL Eyal
The standard setting for studying parallel server systems (PSS) at the diffusion scale is based on the heavy traffic condition (HTC)...
-
The Maximal Agreement Subtree problem for random trees
BUDZINSKI Thomas
Consider two binary trees whose leaves are labelled from 1 to n.
-
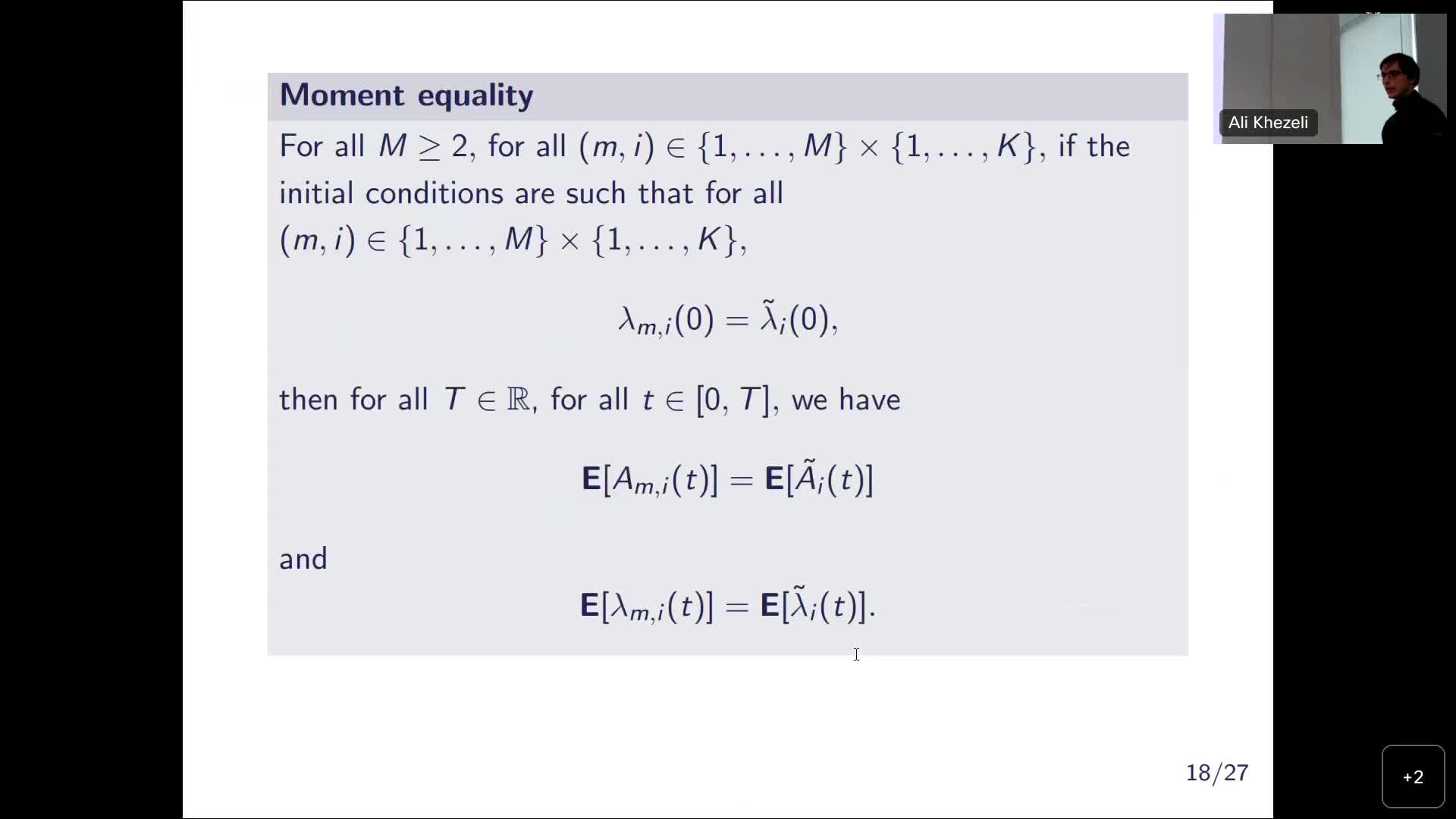
An Improved Lower Bound on the Largest Common Subtree of Random Leaf-Labeled Binary Trees
KHEZELI Ali
It is known that the size of the largest common subtree...
-
Reversible Markov decision processes
ANANTHARAM Venkat
A Markov decision process is called reversible if for every stationary Markov control strategy the resulting Markov chain is reversible.