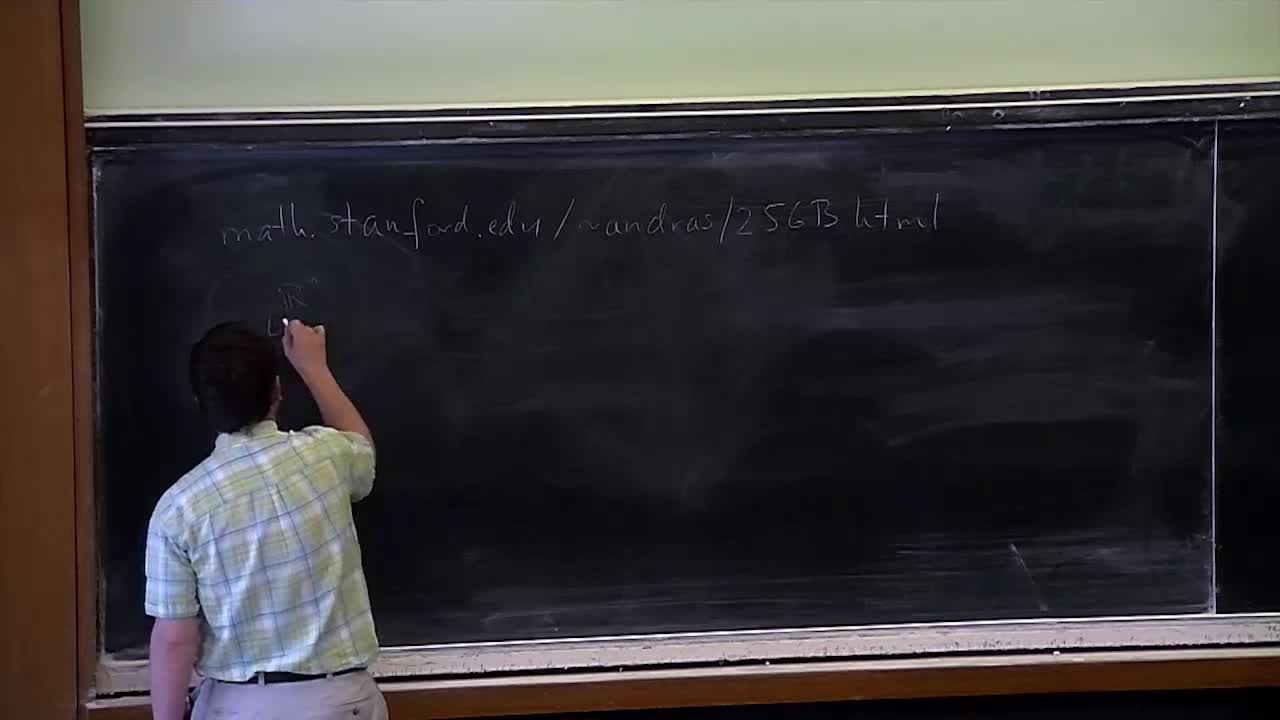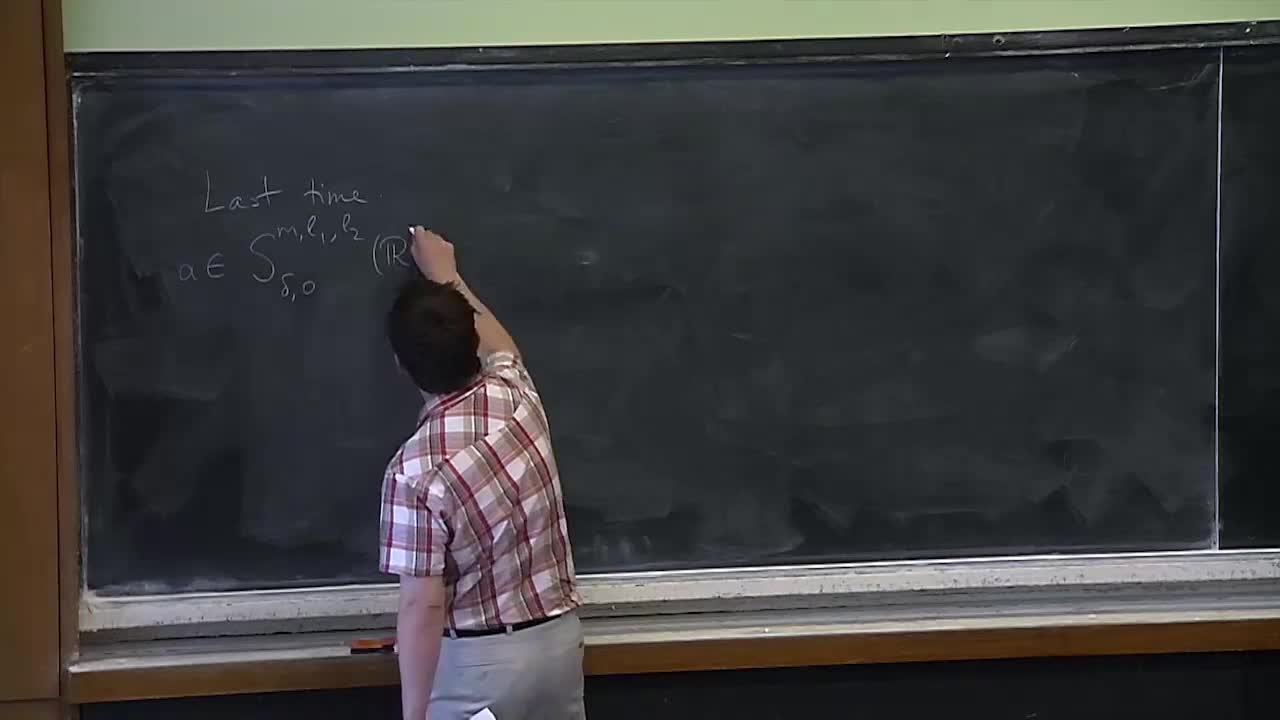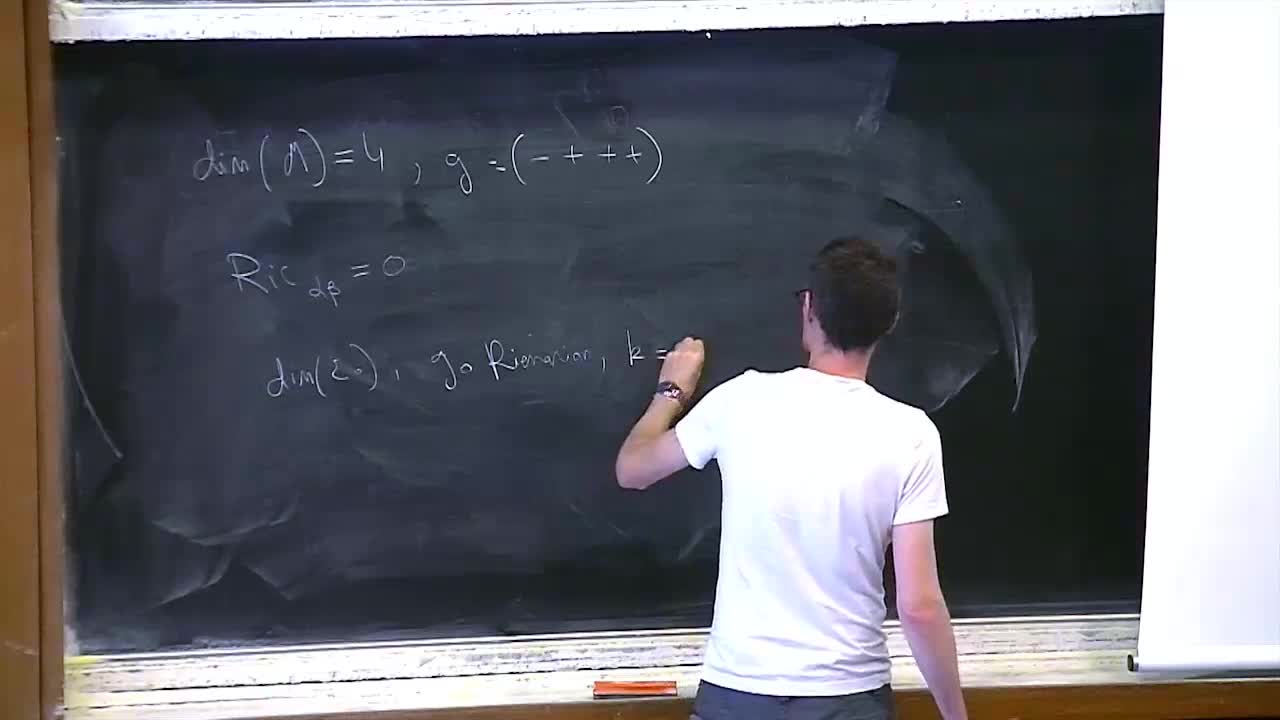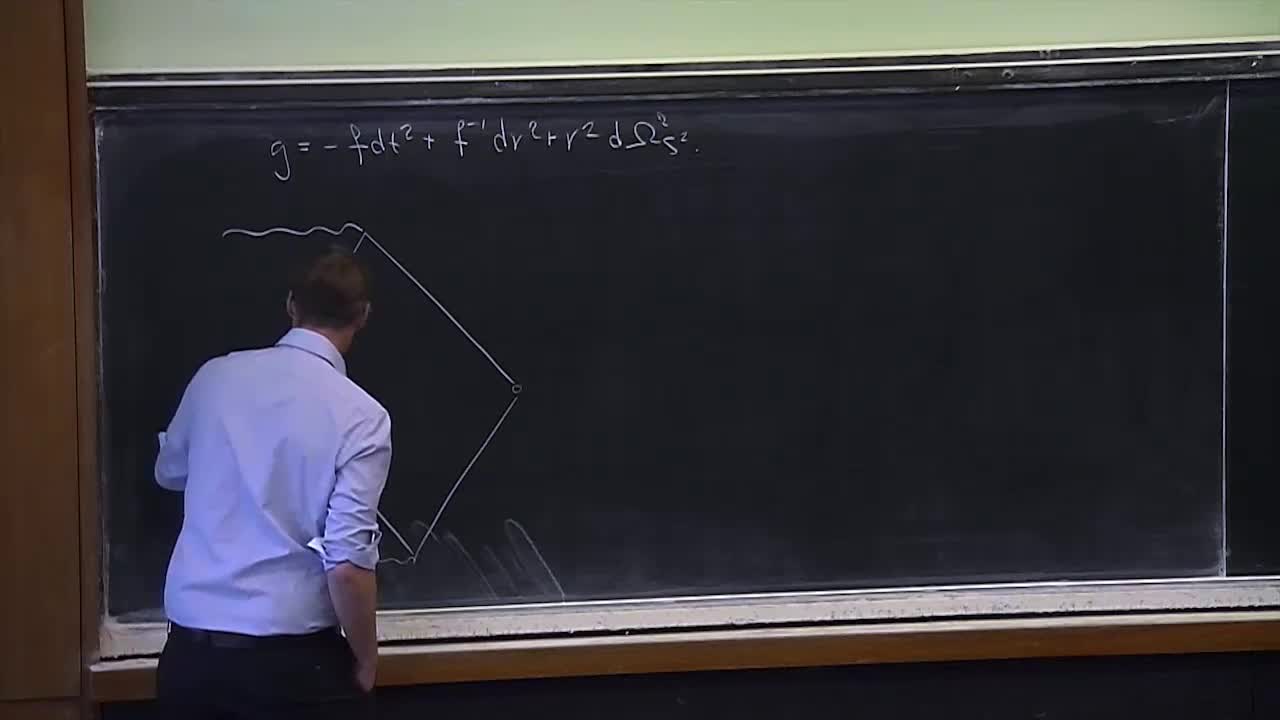2014

Vidéos
Alexander Strohmaier - Workshop
I will explain how one can formulate and formalize the Gupta Bleuler framework for the Quantization of the electromagnetic field in an
Maciej Zworski - From redshift effect to classical dynamics : microlocal proof of Smale's conjecture
Dynamical zeta functions of Selberg, Smale and Ruelle are analogous to the Riemann zeta function with the product over primes replaced by
Jérémie Joudioux - Hertz potentials and the decay of higher spin fields
The study of the asymptotic behavior of higher spin fields has proven to be a key point in understanding the stability properties of
Philippe G LeFloch - Weakly regular spacetimes with T2 symmetry
I will discuss the initial value problem for the Einstein equations and present results concerning the existence and asymptotic behavior of
Lionel Mason - Perturbative formulae for scattering of gravitational wave
The Christodoulou Klainerman proof of existence of asymptotically simple space-times shows that it is reasonable to consider the scattering of
Semyon Dyatlov - Spectral gaps for normally hyperbolic trapping
Motivated by wave decay for Kerr and Kerr de Sitter black holes, we study spectral gaps for codimension 2 normally hyperbolic trapped sets with
Claudio Dappiaggi - On the role of asymptotic structures in the construction of quantum states for …
In the algebraic approach to quantum field theory on curved backgrounds, there exists a special class of quantum states for free fields,
Pieter Blue - Decay for fields outside black holes
I will discuss energy and Morawetz (or integrated local decay) estimates for fields outside black holes. These results build on results for
Thomas Backdahl - Symmetry operators, conserved currents and energy momentum tensors
Conserved quantities, for example energy and momentum, play a fundamental role in the analysis of dynamics of particles and fields. For
Alain Bachelot - Waves in the Anti-de Sitter space-time Ads
In this talk we address some issues concerning the wave propagation in the 4D+1 anti de Sitter space time : the role of the conformal
Andras Vasy - Quasilinear waves and trapping: Kerr‐de Sitter space
In this talk I will describe recent work with Peter Hintz on globally solving quasilinear wave equations in the presence of trapped rays,
Andras Vasy - Microlocal analysis and wave propagation (Part 4)
In these lectures I will explain the basics of microlocal analysis, emphasizing non elliptic problems, such as wave propagation, both on manifolds without boundary, and on manifolds with boundary. In
Andras Vasy - Microlocal analysis and wave propagation (Part 3)
In these lectures I will explain the basics of microlocal analysis, emphasizing non elliptic problems, such as wave propagation, both on manifolds without boundary, and on manifolds with boundary. In
Andras Vasy - Microlocal analysis and wave propagation (Part 2)
In these lectures I will explain the basics of microlocal analysis, emphasizing non elliptic problems, such as wave propagation, both on manifolds without boundary, and on manifolds with boundary. In
Andras Vasy - Microlocal analysis and wave propagation (Part 1)
In these lectures I will explain the basics of microlocal analysis, emphasizing non elliptic problems, such as wave propagation, both on
Jérémie Szeftel - General relativity (Workshop)
In order to control locally a space time which satisfies the Einstein equations, what are the minimal assumptions one should make on its
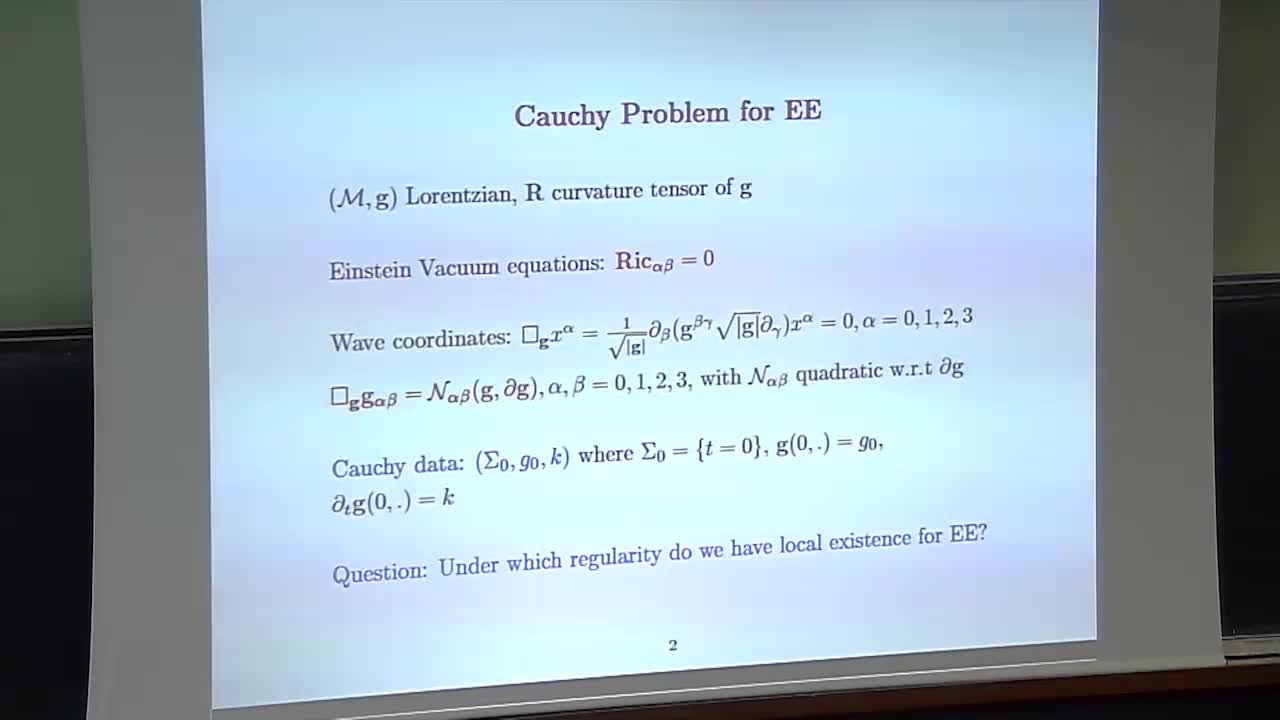
Jérémie Szeftel The resolution of the bounded L2 curvature conjecture in General Relativity (Part 1)
In order to control locally a space time which satisfies the Einstein equations, what are the minimal assumptions one should make on its
Jérémie Szeftel The resolution of the bounded L2 curvature conjecture in General Relativity (Part 2)
In order to control locally a space-‐time which satisfies the Einstein equations, what are the minimal assumptions one should make on its curvature tensor? The bounded L2 curvature conjecture roughly
Jérémie Szeftel The resolution of the bounded L2 curvature conjecture in General Relativity (Part 3)
In order to control locally a space-‐time which satisfies the Einstein equations, what are the minimal assumptions one should make on its curvature tensor? The bounded L2 curvature conjecture roughly
Jérémie Szeftel The resolution of the bounded L2 curvature conjecture in General Relativity (Part 4)
In order to control locally a space-‐time which satisfies the Einstein equations, what are the minimal assumptions one should make on its
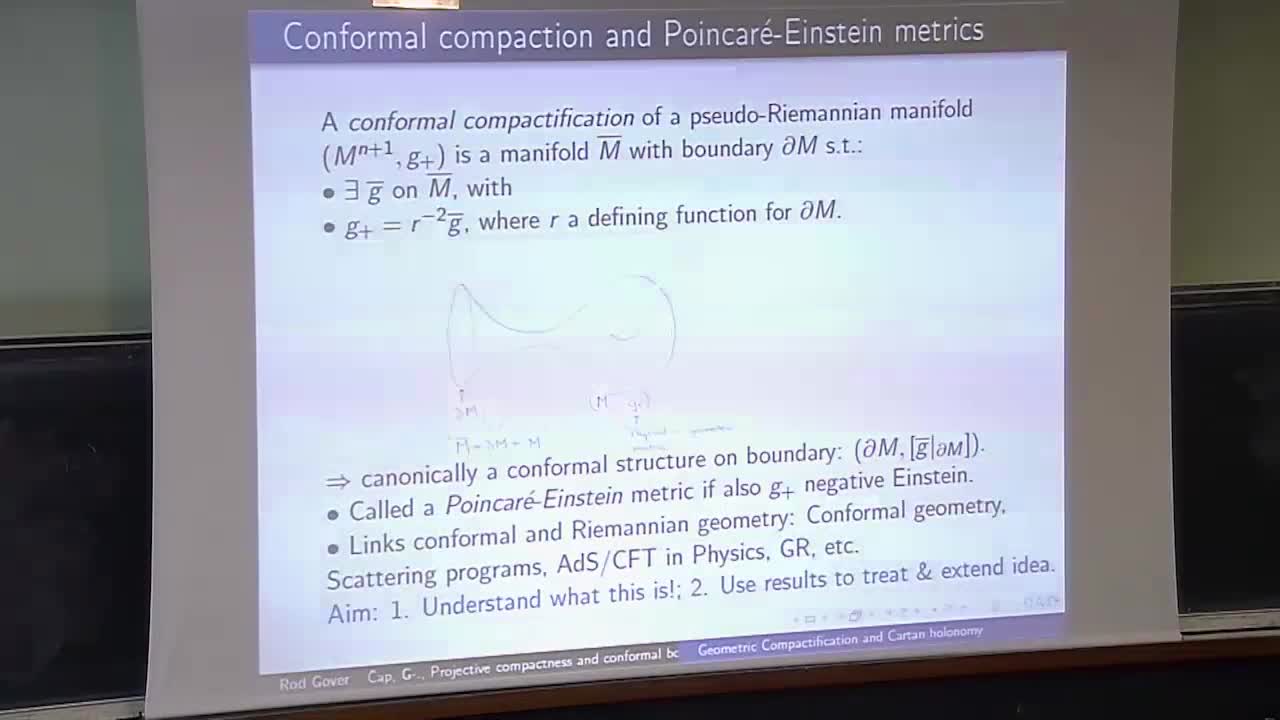
Rod Gover - Geometric Compactification, Cartan holonomy, and asymptotics
Conformal compactification has long been recognised as an effective geometric framework for relating conformal geometry, and associated field theories `
Rod Gover - An introduction to conformal geometry and tractor calculus (Part 4)
After recalling some features (and the value of) the invariant ``Ricci calculus'' of pseudo-‐Riemannian geometry, we look at conformal rescaling from an elementary perspective. The idea of conformal
Rod Gover - An introduction to conformal geometry and tractor calculus (Part 3)
After recalling some features (and the value of) the invariant ``Ricci calculus'' of pseudo-‐Riemannian geometry, we look at conformal rescaling from an elementary perspective. The idea of conformal
Rod Gover - An introduction to conformal geometry and tractor calculus (Part 2)
After recalling some features (and the value of) the invariant ``Ricci calculus'' of pseudo-‐Riemannian geometry, we look at conformal rescaling from an elementary perspective. The idea of conformal
Rod Gover - An introduction to conformal geometry and tractor calculus (Part 1)
After recalling some features (and the value of) the invariant ``Ricci calculus'' of pseudo-‐Riemannian geometry, we look at conformal rescaling
Christian Gérard - Construction of Hadamard states for Klein‐Gordon fields
we will review a new construction of Hadamard states for quantized Klein-‐Gordon fields on curved spacetimes, relying on pseudo differential
Christian Gérard - Introduction to field theory on curved spacetimes (Part 4)
The aim of these lectures is to give an introduction to quantum field theory on curved spacetimes, from the point of view of partial differential equations and microlocal analysis. I will concentrate
Christian Gérard - Introduction to field theory on curved spacetimes (Part 3)
The aim of these lectures is to give an introduction to quantum field theory on curved spacetimes, from the point of view of partial differential equations and microlocal analysis. I will concentrate
Christian Gérard - Introduction to field theory on curved spacetimes (Part 2)
The aim of these lectures is to give an introduction to quantum field theory on curved spacetimes, from the point of view of partial differential equations and microlocal analysis. I will concentrate
Christian Gérard - Introduction to field theory on curved spacetimes (Part 1)
The aim of these lectures is to give an introduction to quantum field theory on curved spacetimes, from the point of view of partial differential equations and microlocal analysis. I will concentrate
Lars Andersson - Symmetry operators and energies
Black holes play a central role in general relativity and astrophysics. The problem of proving the dynamical stability of the Kerr black hole spacetime, which is describes a rotating black hole in
Lars Andersson - Geometry and analysis in black hole spacetimes (Part 4)
Black holes play a central role in general relativity and astrophysics. The problem of proving the dynamical stability of the Kerr black hole spacetime, which is describes a rotating black hole in
Lars Andersson - Geometry and analysis in black hole spacetimes (Part 3)
Black holes play a central role in general relativity and astrophysics. The problem of proving the dynamical stability of the Kerr black hole spacetime, which is describes a rotating black hole in
Lars Andersson - Geometry and analysis in black hole spacetimes (Part 2)
Black holes play a central role in general relativity and astrophysics. The problem of proving the dynamical stability of the Kerr black hole spacetime, which is describes a rotating black hole in
Lars Andersson - Geometry and analysis in black hole spacetimes (Part 1)
Black holes play a central role in general relativity and astrophysics. The problem of proving the dynamical stability of the Kerr black
Intervenants et intervenantes
Professeur à l'Institut Albert Einstein (Postdam, Allemagne) en 2014
Professeur et directeur du laboratoire de Mathématiques Appliquées de Bordeaux (MAB), à l'Université de Bordeaux 1, en 2002. Titulaire d'un doctorat en Mathématiques Appliquées (Bordeaux I, 1981)
Enseigne à la Chalmers University of Technology à Gothenburg (Suède) (2014)
Professeur de mathématiques à l'université d’Édimbourg, Royaume-Uni (en 2020)
Rapporteur d'une thèse en Mathématiques appliqués soutenue à Sorbonne université en 2019
Professeur à l'Université de Princeton
Professeur associé de physique mathématique au département de physique de l'université de Pavie, Italie (en 2019)
Mathématicien. En poste à l'université de Californie, Berkeley (en 2019)
Mathématicien. En poste : Université d'Auckland
Elève-ingénieur de l'Ecole polytechnique, promotion 1979. Enseigne au Département de mathématiques de l'Université de Paris-XI (en 1987)
Auteur d’un mémoire de master recherche 2e année en mathematiques soutenu à l'université Montpellier 2, en 2006
Docteur en mathématiques (Brest, 2010)
Ph.D. (Université de New York, 1978)
Mathématicien. Professeur à l'Université de Princeton (en 1993)
Mathématicien. - Directeur de recherche CNRS, Centre de Mathématiques appliquées, Ecole Polytechnique, Palaiseau (en 2002). - Directeur de thèse à l'Université Pierre et Marie Curie - Paris 6 en 2009
Physicien. Professeur au Mathematical institute, Oxford university, Oxford, GB (en 2017)
Chercheur en Physique mathématiques à l'Université de Leeds (2014)
Mathématicien. Directeur de recherche CNRS, Laboratoire Jacques-Louis Lions, Sorbonne Université, Paris (en 2024)
Professeur à l'Université de Stanford, États-unis (en 2017)
Mathématicien. En poste : Departement of mathematics, MIT, Cambridge, MA, USA (en 2000)
Mathématicien. En poste : Université de Californie à Berkeley, Calif. (depuis 1998)