Notice
Christian Gérard - Introduction to field theory on curved spacetimes (Part 3)
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
The aim of these lectures is to give an introduction to quantum field theory on curved spacetimes, from the point of view of partial differential equations and microlocal analysis. I will concentrate on free fields and quasi-free states, and say very little on interacting fields or perturbative renormalization. I will start by describing the necessary algebraic background, namely CCR and CAR algebras, and the notion of quasi-free states, with their basic properties and characterizations. I will then introduce the notion of globally hyperbolic spacetimes, and its importance for classical field theory (advanced and retarded fundamental solutions, unique solvability of the Cauchy problem). Using these results I will explain the algebraic quantization of the two main examples of quantum fields ona manifold, namely the Klein-Gordon (bosonic) and Dirac (fermionic) fields.In the second part of the lectures I will discuss the important notion of Hadamardstates , which are substitutes in curved spacetimes for the vacuum state in Minkowskispacetime. I will explain its original motivation, related to the definition of therenormalized stress-energy tensor in a quantum field theory. I will then describethe modern characterization of Hadamard states, by the wavefront set of their twopointfunctions, and prove the famous Radzikowski theorem , using the Duistermaat-Hörmander notion of distinguished parametrices . If time allows, I will also describe the quantization of gauge fields, using as example the Maxwell field.
Documentation
Liens
Dans la même collection
-
Lionel Mason - Perturbative formulae for scattering of gravitational wave
MASON Lionel J.
The Christodoulou Klainerman proof of existence of asymptotically simple space-times shows that it is reasonable to consider the scattering of
-
Andras Vasy - Microlocal analysis and wave propagation (Part 4)
In these lectures I will explain the basics of microlocal analysis, emphasizing non elliptic problems, such as wave propagation, both on manifolds without boundary, and on manifolds with boundary. In
-
Alexander Strohmaier - Workshop
STROHMAIER Alexander
I will explain how one can formulate and formalize the Gupta Bleuler framework for the Quantization of the electromagnetic field in an
-
-
Andras Vasy - Microlocal analysis and wave propagation (Part 1)
VASY András
In these lectures I will explain the basics of microlocal analysis, emphasizing non elliptic problems, such as wave propagation, both on
-
-
Alain Bachelot - Waves in the Anti-de Sitter space-time Ads
BACHELOT Alain
In this talk we address some issues concerning the wave propagation in the 4D+1 anti de Sitter space time : the role of the conformal
-
Semyon Dyatlov - Spectral gaps for normally hyperbolic trapping
DYATLOV Semyon
Motivated by wave decay for Kerr and Kerr de Sitter black holes, we study spectral gaps for codimension 2 normally hyperbolic trapped sets with
-
Andras Vasy - Microlocal analysis and wave propagation (Part 3)
In these lectures I will explain the basics of microlocal analysis, emphasizing non elliptic problems, such as wave propagation, both on manifolds without boundary, and on manifolds with boundary. In
-
Maciej Zworski - From redshift effect to classical dynamics : microlocal proof of Smale's conjecture
ZWORSKI Maciej
Dynamical zeta functions of Selberg, Smale and Ruelle are analogous to the Riemann zeta function with the product over primes replaced by
-
Pieter Blue - Decay for fields outside black holes
BLUE Pieter
I will discuss energy and Morawetz (or integrated local decay) estimates for fields outside black holes. These results build on results for
-
Jérémie Szeftel - General relativity (Workshop)
SZEFTEL Jérémie
In order to control locally a space time which satisfies the Einstein equations, what are the minimal assumptions one should make on its
Sur le même thème
-
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HERLéA Alexandre
Alexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
Webinaire sur la rédaction des PGD
LOUVET Violaine
Rédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
A. Mondino - Time-like Ricci curvature bounds via optimal transport
MONDINO Andrea
The goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
-
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass Theorem
LESOURD Martin
The study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in
-
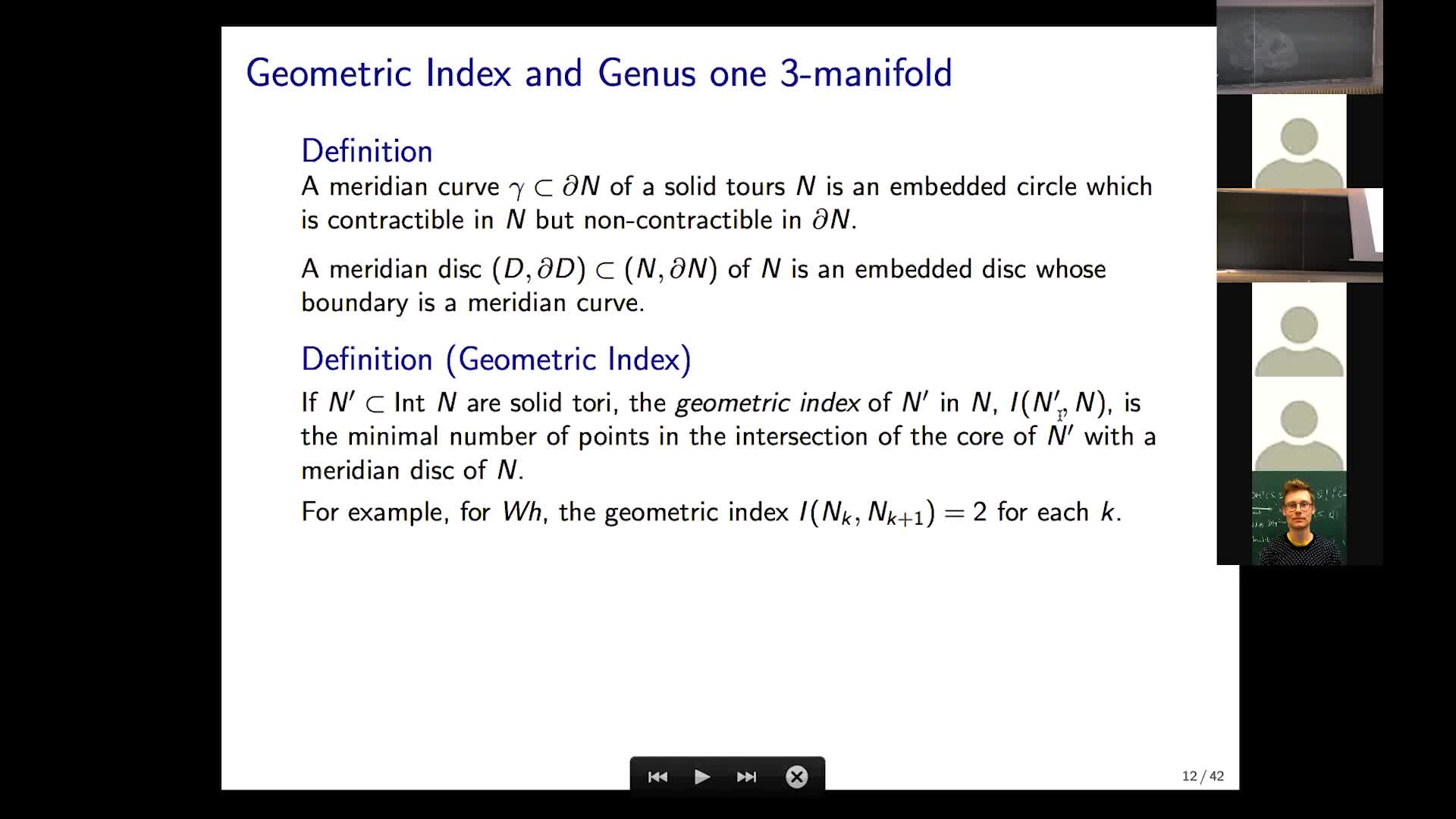
J. Wang - Topological rigidity and positive scalar curvature
WANG Jian
In this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
R. Perales - Recent Intrinsic Flat Convergence Theorems
PERALES Raquel
Théorèmes récents de convergence plane intrinsèque
-
J. Fine - Knots, minimal surfaces and J-holomorphic curves
FINE Joël
I will describe work in progress, parts of which are joint with Marcelo Alves. Let L be a knot or link in the 3-sphere. I will explain how one can count minimal surfaces in hyperbolic 4-space
-
D. Semola - Boundary regularity and stability under lower Ricci bounds
SEMOLA Daniele
The theory of non smooth spaces with lower Ricci Curvature bounds has undergone huge developments in the last thirty years. On the one hand the impetus came from Gromov’s precompactness theorem
-
D. Stern - Harmonic map methods in spectral geometry
STERN Daniel
Over the last fifty years, the problem of finding sharp upper bounds for area-normalized Laplacian eigenvalues on closed surfaces has attracted the attention of many geometers, due in part to
-
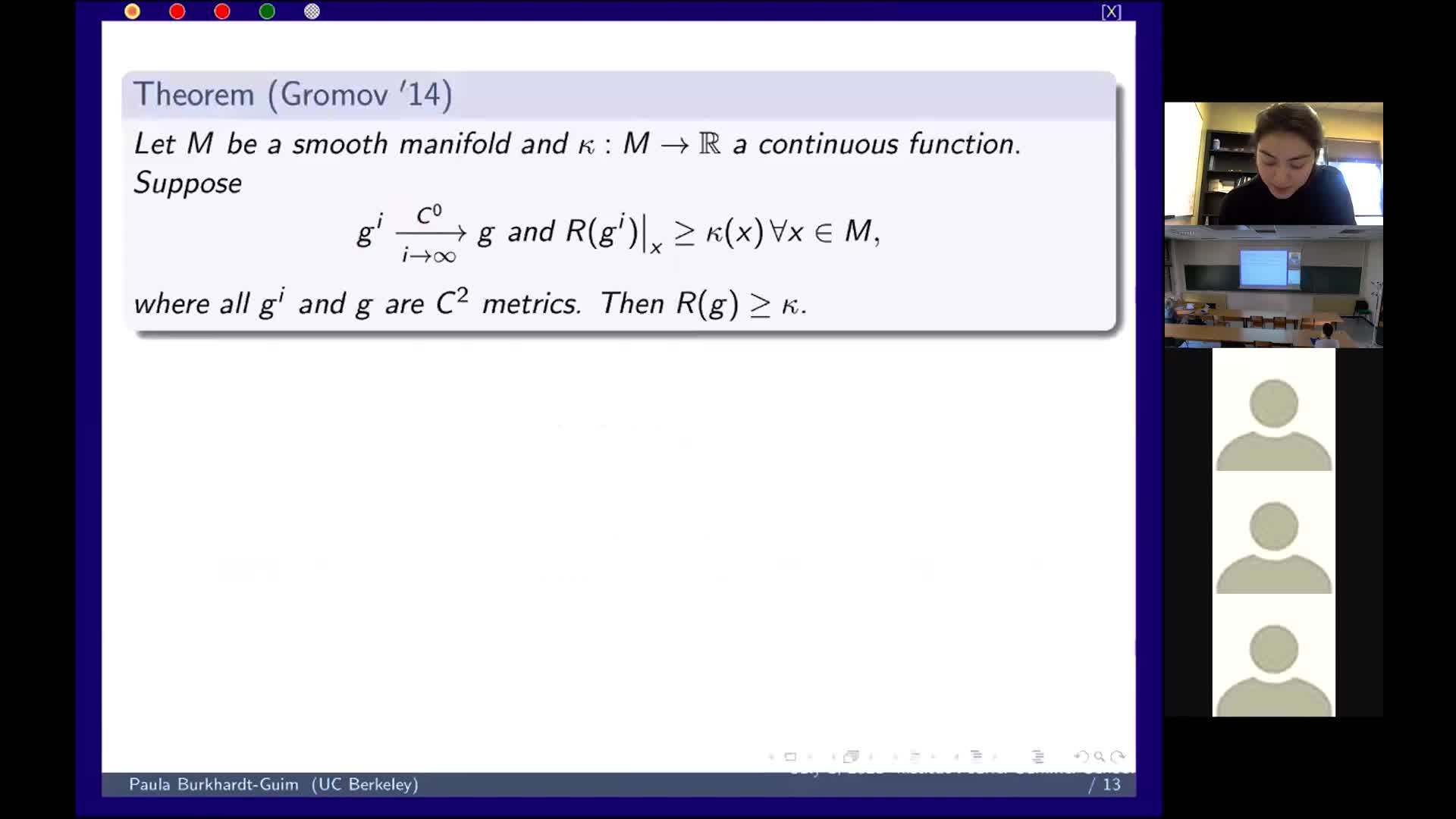
P. Burkhardt - Pointwise lower scalar curvature bounds for C0 metrics via regularizing Ricci flow
BURKHARDT-GUIM Paula
We propose a class of local definitions of weak lower scalar curvature bounds that is well defined for C0 metrics. We show the following: that our definitions are stable under greater-than-second
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BAMLER Richard H.
We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.