Notice
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
Né en Roumanie, en 1893, Petre Sergescu, appartient à une pléiade de Roumains dont l’œuvre fait partie intégrante de la culture française, voire européenne. Il a joué un rôle majeur dans l’organisation institutionnelle de l’Histoire des Sciences, au plan international.
Après de brillantes études à Bucarest et Paris il est Professeur titulaire de mathématiques et Président de l’École Polytechnique de Bucarest. En 1946, après l’occupation de la Roumanie par l’armée soviétique, il se réfugie en France où tout en étant très actif au sein de l’émigration roumaine se consacre à la réanimation de l’Académie Internationale d’Histoire des Sciences - AIHS dont il est, depuis 1937, vice-président, pour être élu Président en 1947 et secrétaire perpétuel, en 1950. En 1947 il est élu également secrétaire général de l’Union Internationale d’Histoire des Sciences - UIHS et devient rédacteur en chef des „Archives Internationales d’Histoire des Sciences”
Petre Sergescu décède à Paris en décembre 1954, en pleine activité.
Thème
Sur le même thème
-
Lancement de la collection "Truchements"
MONOD-BECQUELIN Aurore
CUNILL Caroline
DéLéAGE Pierre
RIVERTI Camille
GHERMAN Vasile
ZIMMERMANN Théo
STOICHITă Victor Alexandre
CHEMILLIER Marc
Lancement de la nouvelle collection "Truchements" des éditions de la Société d'ethnologie de Nanterre, avec la présentation en mots et en musique de son premier titre, "Passeurs de mémoire", de
-
Webinaire sur la rédaction des PGD
LOUVET Violaine
Rédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BAMLER Richard H.
We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.
-
C. Li - Classifying sufficiently connected PSC manifolds in 4 and 5 dimensions
LI Chao
In this talk, I will discuss some recent developments on the topology of closed manifolds admitting Riemannian metrics of positive scalar curvature. In particular, we will prove if a closed PSC
-
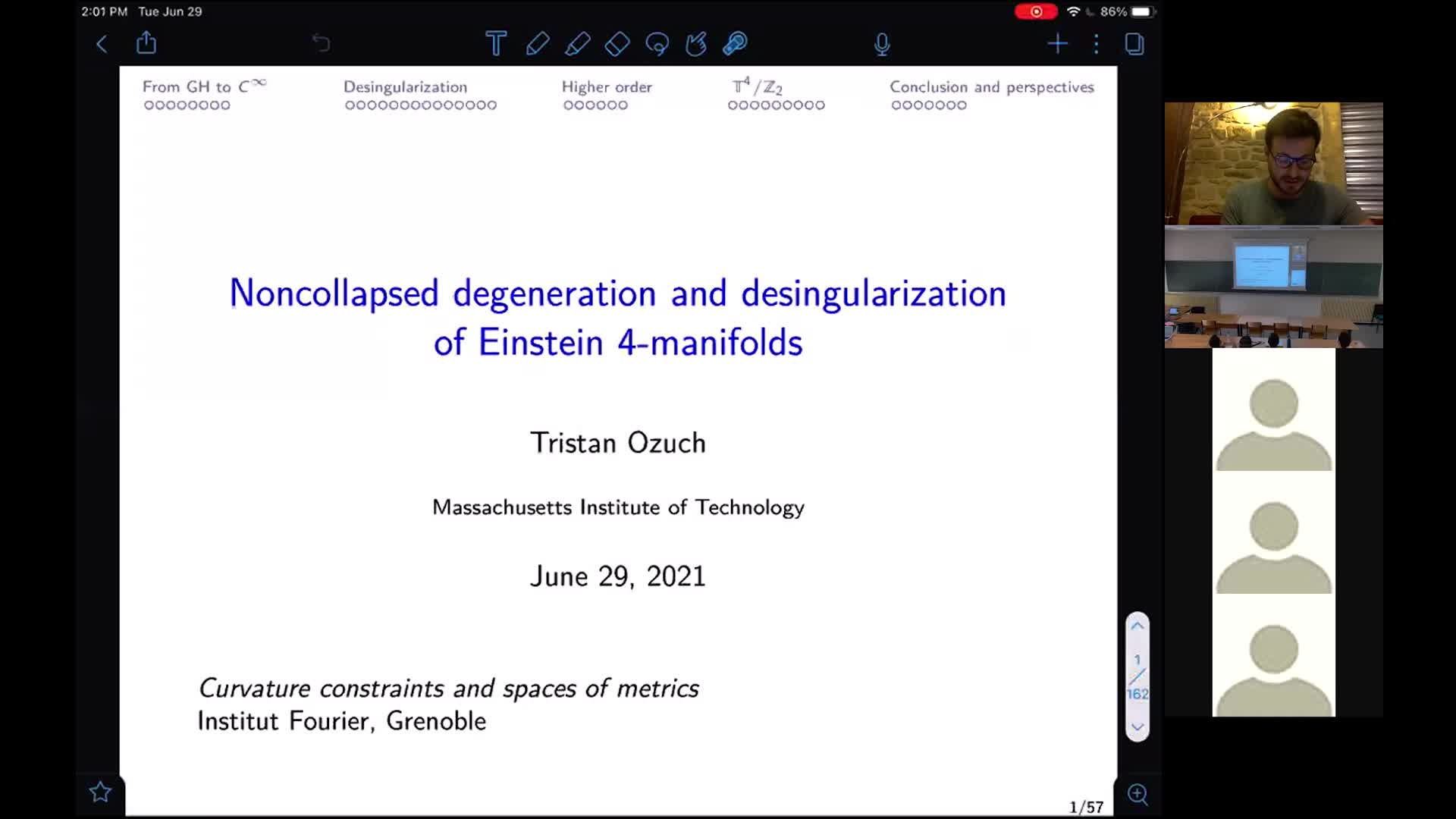
T. Ozuch - Noncollapsed degeneration and desingularization of Einstein 4-manifolds
OZUCH Tristan
We study the noncollapsed singularity formation of Einstein 4-manifolds. We prove that any smooth Einstein 4-manifold close to a singular one in a mere Gromov-Hausdorff (GH) sense is the result
-
D. Tewodrose - Limits of Riemannian manifolds satisfying a uniform Kato condition
TEWODROSE David
Presentation of a joint work with G. Carron and I. Mondello where we study Kato limit spaces.
-
J. Wang - Topological rigidity and positive scalar curvature
WANG Jian
In this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
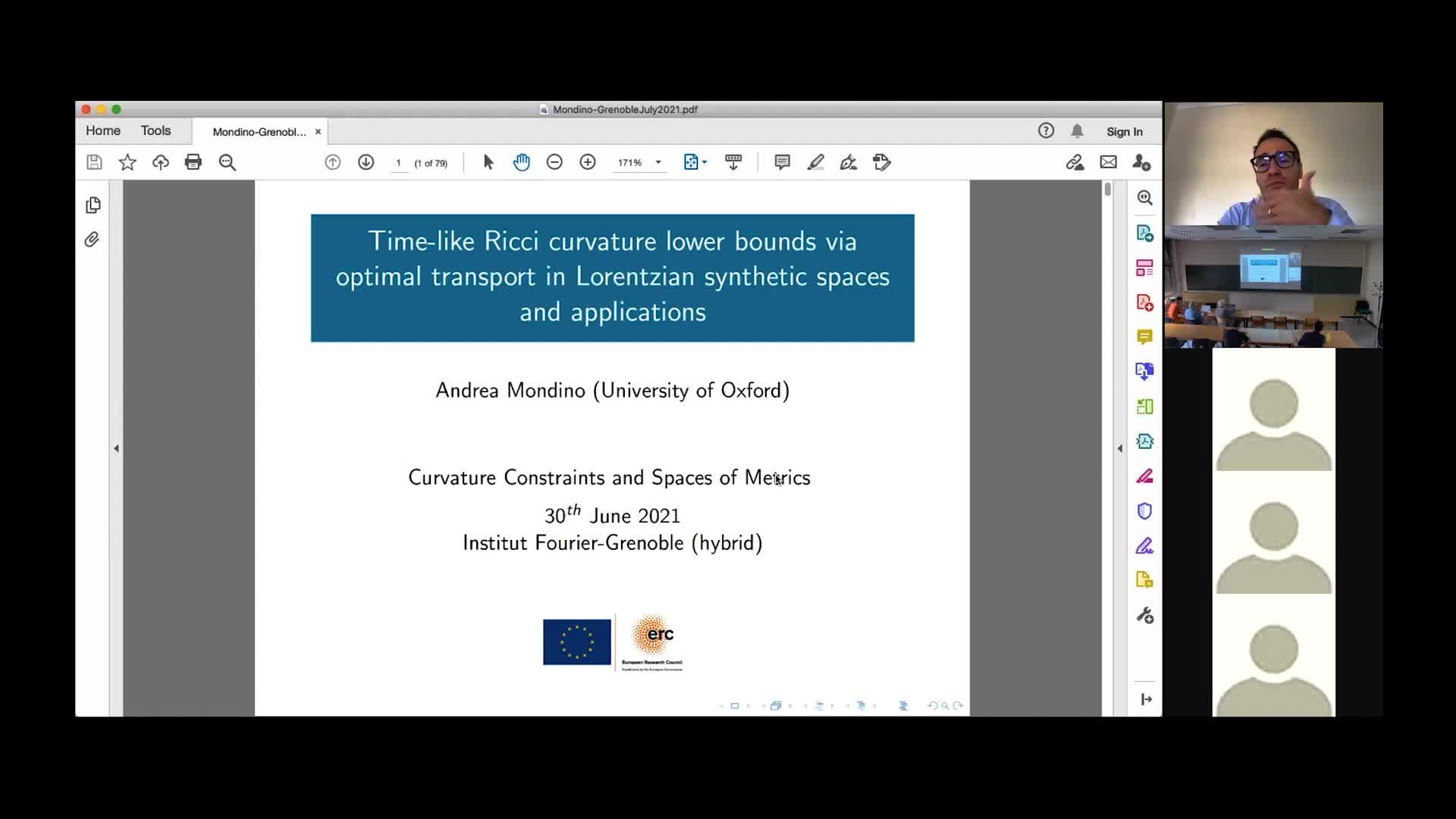
A. Mondino - Time-like Ricci curvature bounds via optimal transport
MONDINO Andrea
The goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
-
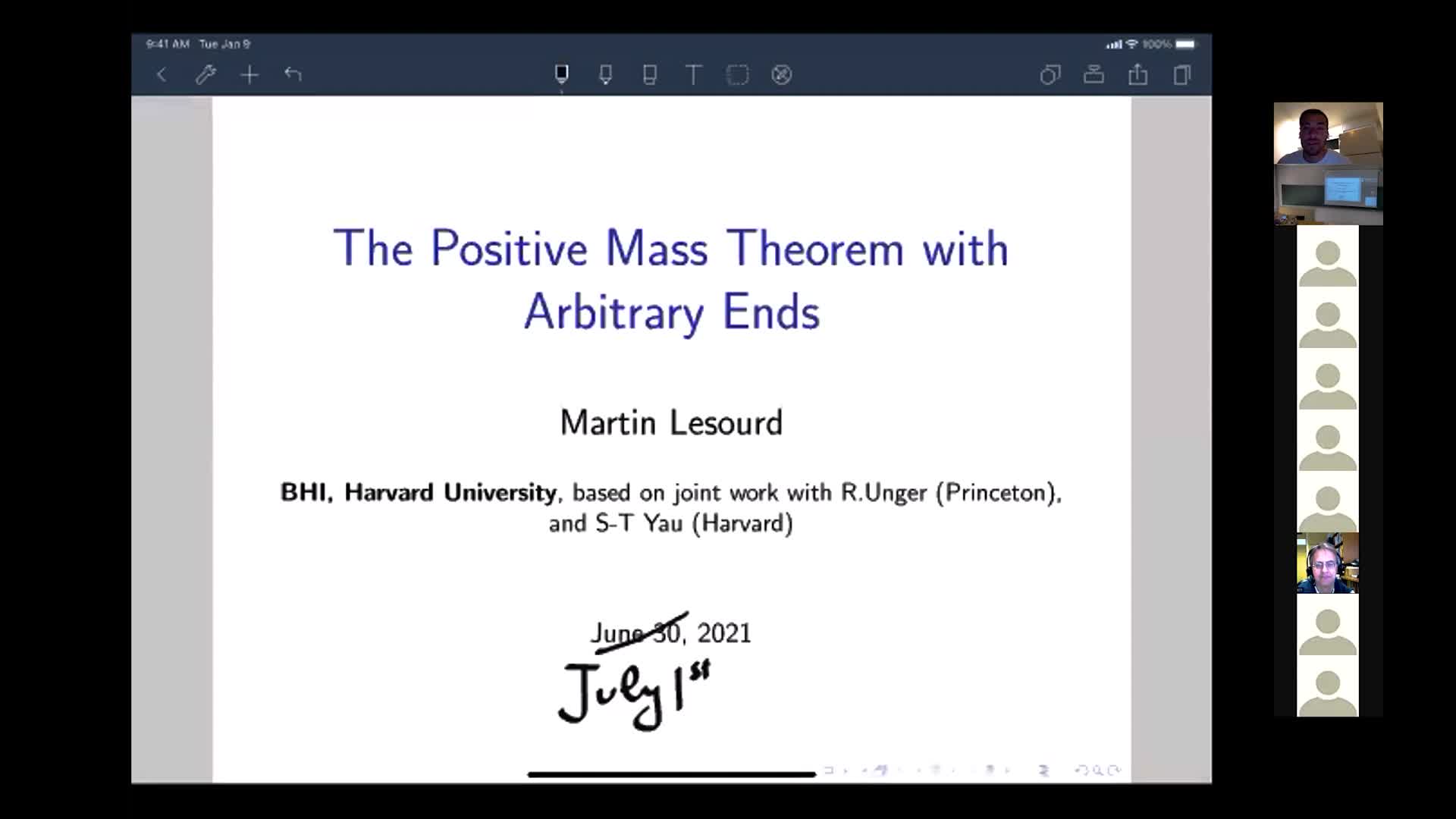
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass Theorem
LESOURD Martin
The study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in
-
R. Perales - Recent Intrinsic Flat Convergence Theorems
PERALES Raquel
Théorèmes récents de convergence plane intrinsèque
-
J. Fine - Knots, minimal surfaces and J-holomorphic curves
FINE Joël
I will describe work in progress, parts of which are joint with Marcelo Alves. Let L be a knot or link in the 3-sphere. I will explain how one can count minimal surfaces in hyperbolic 4-space