I. Pasquinelli - Deligne-Mostow lattices and cone metrics on the sphere
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
- audio 1 audio 2 audio 3
Descriptif
Finding lattices in PU(n,1) has been one of the major challenges of the last decades. One way of constructing a lattice is to give a fundamental domain for its action on the complex hyperbolic space.One approach, successful for some lattices, consists of seeing the complex hyperbolic space as the configuration space of cone metrics on the sphere and of studying the action of some maps exchanging the cone points with same cone angle.In this talk we will see how this construction can be used to build fundamental polyhedra for all Deligne-Mostow lattices in PU(2,1).
Intervenant
Thème
Notice
Dans la même collection
-
V. Gadre - Effective convergence of ergodic averages and cusp excursions of geodesics
Effective convergence of ergodic averages and cusp excursions of geodesics on moduli spaces We survey some applications of effective convergence of ergodic averages to the analysis of cusp ex
-
C.Fougeron - Diffusion rate for windtree modelsFougeronCharles
Recent results on windtree models with polygonal obstacles have linked their diffusion rate with Lyapunov exponents in stata of quadratic differentials. The proves of these theorems follow from the
-
D. Davis - Periodic paths on the pentagonDavisDiana
Mathematicians have long understood periodic trajectories on the square billiard table. In the present work, we describe periodic trajectories on the regular pentagon – their geometry, symbolic
-
R. Santharoubane - Quantum representations of surface groupsSantharoubaneRamanujan Harischandra
I will show how we can produce exotic representations of surface groups from the Witten-Reshetikhin-Turaev TQFT. These representations have infinite images and give points on character varieties that
-
-
P. Apisa - Marked points in genus two and beyond
In the principal stratum in genus two, McMullen observed that something odd happens - there is only one nonarithmetic Teichmuller curve - the one generated by the decagon. This strange phenomenon
-
S.Schleimer - An introduction to veering triangulations
Singular euclidean structures on surfaces are a key tool in the study of the mapping class group, of Teichmüller space, and of kleinian three-manifolds. François Guéritaud, while studying work of Ian
-
R. Gutierrez - Quaternionic monodromies of the Kontsevich–Zorich cocycleGutiérrezRodolfo
The monodromy group of a translation surface M is the Lie group spanned by all symplectic matrices arising from the homological action of closed loops at M (inside its embodying orbit closure). In the
-
Ö. Yurttas - Algorithms for multicurves with Dynnikov coordinates
Multicurves have played a fundamental role in the study of mapping class groups of surfaces since the work of Dehn. A beautiful method of describing such systems on the n-punctured disk is given by
-
-
L. Liechti - Minimal dilatations on nonorientable surfaces
We discuss the problem of finding the minimal dilatation among pseudo-Anosov mapping classes on a fixed closed surface. In particular, for every nonorientable closed surface of even genus, we consider
-
A. Wright - Nearly Fuchsian surface subgroups of finite covolume Kleinian groupsWrightAlexander
Multicurves have played a fundamental role in the study of mapping class groups of surfaces since the work of Dehn. A beautiful method of describing such systems on the n-punctured disk is given by
Sur le même thème
-
Webinaire sur la rédaction des PGDLouvetViolaine
Rédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
J. Fine - Knots, minimal surfaces and J-holomorphic curvesFineJoël
I will describe work in progress, parts of which are joint with Marcelo Alves. Let L be a knot or link in the 3-sphere. I will explain how one can count minimal surfaces in hyperbolic 4-space
-
D. Tewodrose - Limits of Riemannian manifolds satisfying a uniform Kato conditionTewodroseDavid
I will present a joint work with G. Carron and I. Mondello where we study Kato limit spaces. These are metric measure spaces obtained as Gromov-Hausdorff limits of smooth n-dimensional Riemannian
-
D. Stern - Harmonic map methods in spectral geometrySternDaniel
Over the last fifty years, the problem of finding sharp upper bounds for area-normalized Laplacian eigenvalues on closed surfaces has attracted the attention of many geometers, due in part to
-
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass TheoremLesourdMartin
The study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in
-
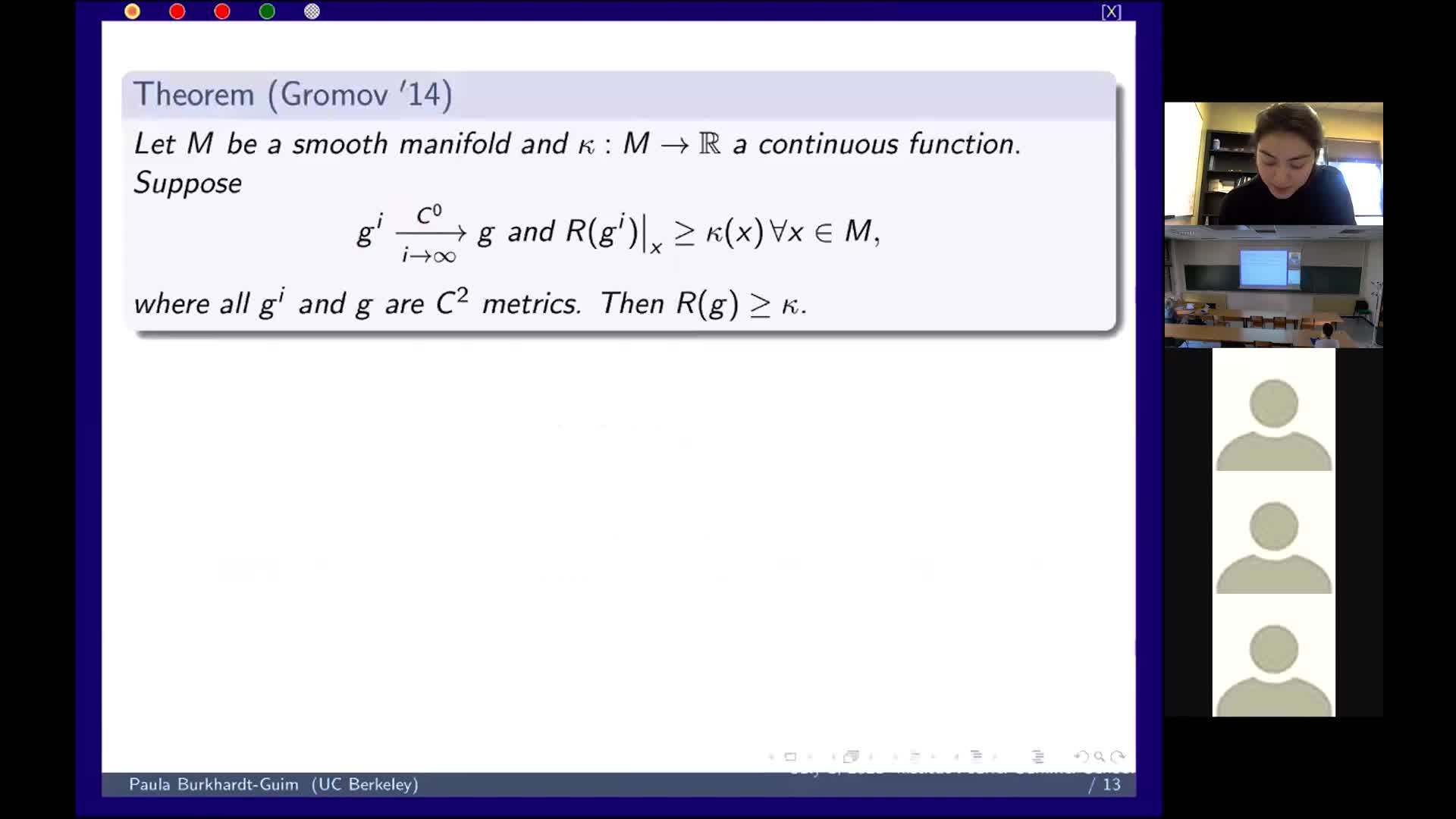
P. Burkhardt - Pointwise lower scalar curvature bounds for C0 metrics via regularizing Ricci flowBurkhardt-GuimPaula
We propose a class of local definitions of weak lower scalar curvature bounds that is well defined for C0 metrics. We show the following: that our definitions are stable under greater-than-second
-
J. Wang - Topological rigidity and positive scalar curvatureWangJian
In this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
D. Semola - Boundary regularity and stability under lower Ricci boundsSemolaDaniele
The theory of non smooth spaces with lower Ricci Curvature bounds has undergone huge developments in the last thirty years. On the one hand the impetus came from Gromov’s precompactness theorem
-
C. Li - Classifying sufficiently connected PSC manifolds in 4 and 5 dimensionsLiChao
In this talk, I will discuss some recent developments on the topology of closed manifolds admitting Riemannian metrics of positive scalar curvature. In particular, we will prove if a closed PSC
-
A. Mondino - Time-like Ricci curvature bounds via optimal transportMondinoAndrea
The goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
-
Y. Lai - A family of 3d steady gradient Ricci solitons that are flying wingsLaiYi
We find a family of 3d steady gradient Ricci solitons that are flying wings. This verifies a conjecture by Hamilton. For a 3d flying wing, we show that the scalar curvature does not vanish at
