Notice
Christian Gérard - Aspects de la théorie quantique des champs en espace-temps courbe
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
La théorie quantique des champs est formulée d'habitude sur l'espace-temps plat de Minkowski.L'extension de ce cadre à des espaces-temps généraux permet de mettre en lumière de nouveaux phénomènes quantiques qui surviennent en présence d'un champ gravitationnel fort.
Nous présenterons tout d'abord le cadre algébrique de la théorie des champs libres en espace-temps courbe, en traitant le cas modèle d'un champ de Klein-Gordon.
Dans une deuxième partie nous aborderons les difficultés nouvelles dues à l'absence d'un groupe d'isométries sur un espace-temps courbe, qui se traduisent physiquement par l'absence d'un état de vide naturel.Nous illustrerons ces difficultés par deux effets emblématiques de la théorie des champs en espace-temps courbe, l'effet Unruh et l'effet Hawking.Enfin nous décrirons les avancées relativement récentes dans la caractérisation d'états physiquement raisonnables en espace-temps courbe, basées sur l'utilisation de l'analyse microlocale.
Thème
Documentation
Avec les mêmes intervenants et intervenantes
-
Christian Gérard - Introduction to field theory on curved spacetimes (Part 1)
GérardChristianThe aim of these lectures is to give an introduction to quantum field theory on curved spacetimes, from the point of view of partial differential equations and microlocal analysis. I will concentrate
Sur le même thème
-
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HerléaAlexandreAlexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
Webinaire sur la rédaction des PGD
LouvetViolaineRédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
D. Semola - Boundary regularity and stability under lower Ricci bounds
SemolaDanieleThe theory of non smooth spaces with lower Ricci Curvature bounds has undergone huge developments in the last thirty years. On the one hand the impetus came from Gromov’s precompactness theorem
-
D. Stern - Harmonic map methods in spectral geometry
SternDanielOver the last fifty years, the problem of finding sharp upper bounds for area-normalized Laplacian eigenvalues on closed surfaces has attracted the attention of many geometers, due in part to
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BamlerRichard H.We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.
-
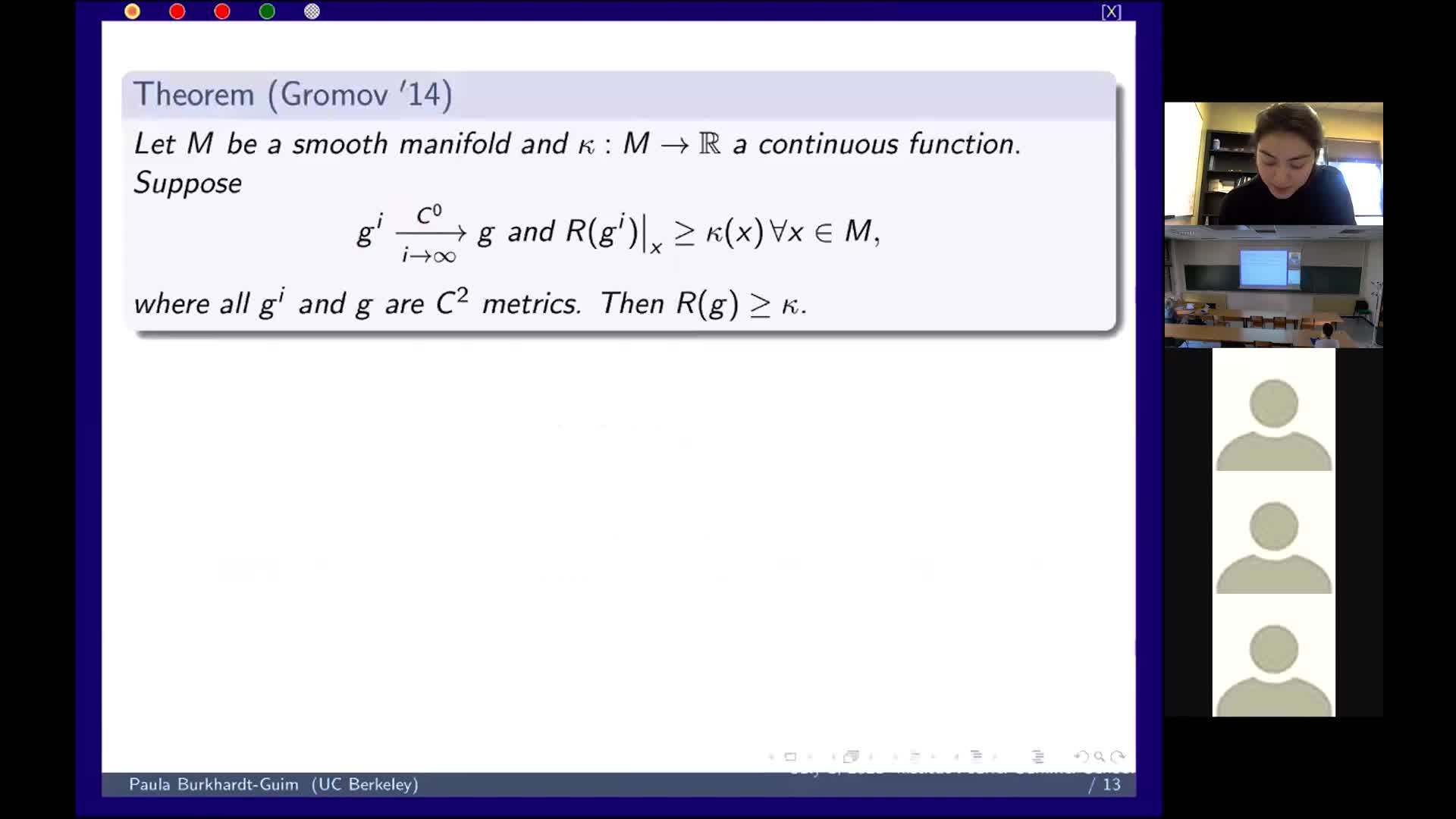
P. Burkhardt - Pointwise lower scalar curvature bounds for C0 metrics via regularizing Ricci flow
Burkhardt-GuimPaulaWe propose a class of local definitions of weak lower scalar curvature bounds that is well defined for C0 metrics. We show the following: that our definitions are stable under greater-than-second
-
C. Li - Classifying sufficiently connected PSC manifolds in 4 and 5 dimensions
LiChaoIn this talk, I will discuss some recent developments on the topology of closed manifolds admitting Riemannian metrics of positive scalar curvature. In particular, we will prove if a closed PSC
-
T. Ozuch - Noncollapsed degeneration and desingularization of Einstein 4-manifolds
OzuchTristanWe study the noncollapsed singularity formation of Einstein 4-manifolds. We prove that any smooth Einstein 4-manifold close to a singular one in a mere Gromov-Hausdorff (GH) sense is the result
-
D. Tewodrose - Limits of Riemannian manifolds satisfying a uniform Kato condition
TewodroseDavidPresentation of a joint work with G. Carron and I. Mondello where we study Kato limit spaces.
-
Y. Lai - A family of 3d steady gradient Ricci solitons that are flying wings
LaiYiWe find a family of 3d steady gradient Ricci solitons that are flying wings. This verifies a conjecture by Hamilton. For a 3d flying wing, we show that the scalar curvature does not vanish at
-
A. Mondino - Time-like Ricci curvature bounds via optimal transport
MondinoAndreaThe goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
