Notice
Alan Turing, génie méconnu
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
Alan Mathison Turing est un mathématicien britannique auteur de l’article fondateur de la science informatique.
Il est à l’origine de la formalisation des concepts d’algorithme et de calculabilité.
Durant la Seconde Guerre mondiale, il a dirigé les recherches de décryptage des codes secrets générés par la machine Enigma utilisée par les allemands.
Il a travaillé sur un des tous premiers ordinateurs, puis a contribué au débat sur sur la capacité que pourraient avoir les machines à penser et sur la fabrication d’un cerveau artificiel auquel on donnera le nom d’« intelligence artificielle ». Vers la fin de sa vie, il s’est intéressé à des modèles de morphogenèse du vivant conduisant aux « structures deTuring ».
Persécuté pour son homosexualité, il évite la prison en choisissant la castration chimique.
Il se suicide le 7 juin 1954 par empoisonnement au cyanure
Sur le même thème
-
The Materiality of Medieval Sources in our Everyday Research
MARLHOUX Romane
ROSENBERGOVá Sabina
SAAR Ortal-Paz
JASKI Bart
(Mai 2023) [Version française en dessous de la version anglaise]
-
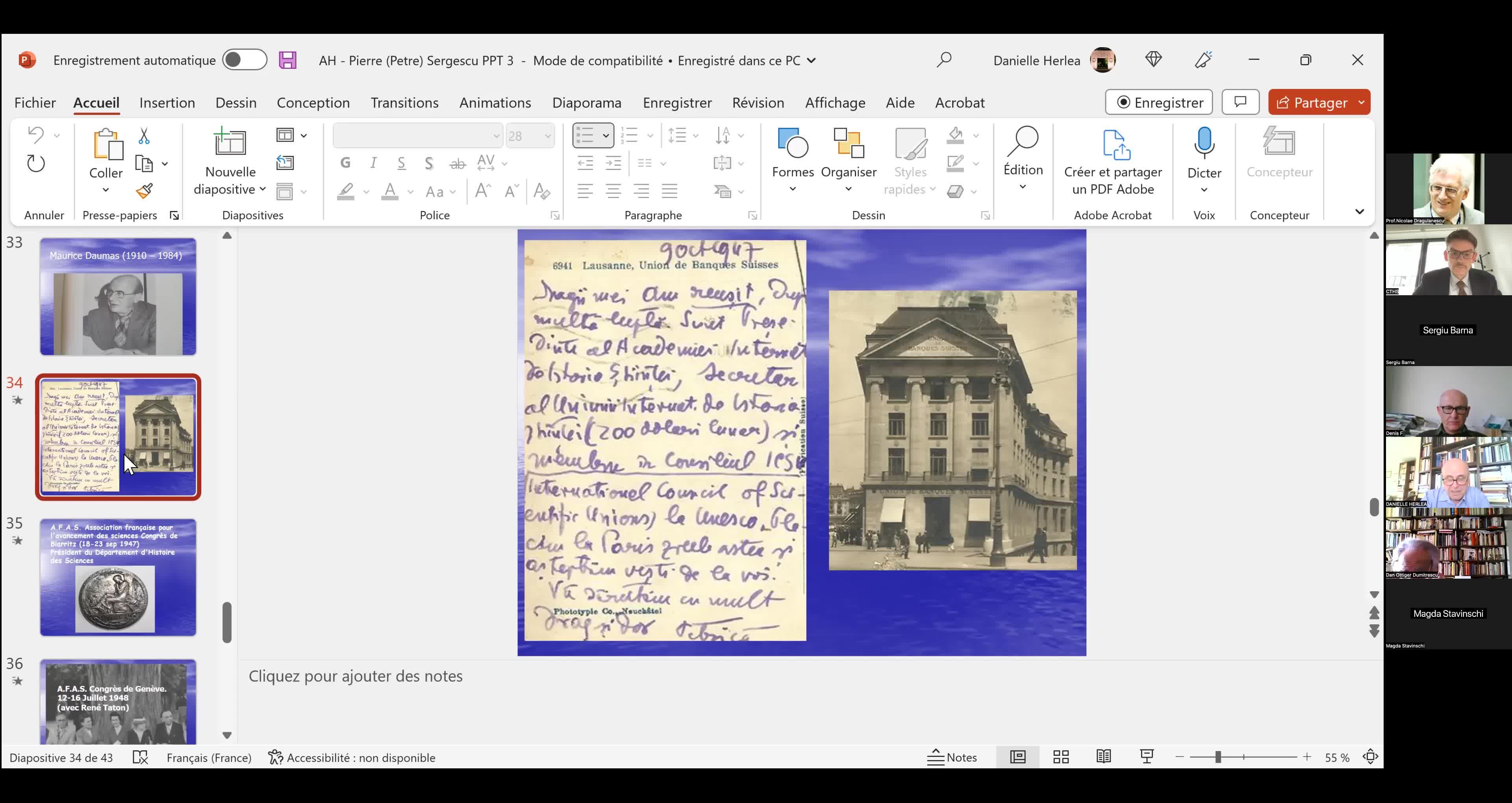
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HERLéA Alexandre
Alexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
L'envers du décor: rhéthorique visionnaire et instrumentalisation politique dans l'art dramatique d…
LEFRANçOIS Frédéric
BERTHET Dominique
"L'envers du décor: rhéthorique visionnaire et instrumentalisation politique dans l'art dramatique de Derek Walcott" Journée d'étude : "Créations, pouvoir et contestation en Caraïbe"
-
Webinaire sur la rédaction des PGD
LOUVET Violaine
Rédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BAMLER Richard H.
We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.
-
C. Li - Classifying sufficiently connected PSC manifolds in 4 and 5 dimensions
LI Chao
In this talk, I will discuss some recent developments on the topology of closed manifolds admitting Riemannian metrics of positive scalar curvature. In particular, we will prove if a closed PSC
-
T. Ozuch - Noncollapsed degeneration and desingularization of Einstein 4-manifolds
OZUCH Tristan
We study the noncollapsed singularity formation of Einstein 4-manifolds. We prove that any smooth Einstein 4-manifold close to a singular one in a mere Gromov-Hausdorff (GH) sense is the result
-
D. Tewodrose - Limits of Riemannian manifolds satisfying a uniform Kato condition
TEWODROSE David
Presentation of a joint work with G. Carron and I. Mondello where we study Kato limit spaces.
-
J. Wang - Topological rigidity and positive scalar curvature
WANG Jian
In this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
A. Mondino - Time-like Ricci curvature bounds via optimal transport
MONDINO Andrea
The goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
-
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass Theorem
LESOURD Martin
The study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in