Notice
3.2. Combinatorial Solutions: Exhaustive Search and Birthday Decoding
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
In this session, I willdetail two combinatorial solutions to the decoding problem. The first one isthe Exhaustive Search. To find our w columns,we will simply enumerate all the tuples j1 tojw and check whether the corresponding columnplus the syndrome is equal to zero modulo 2. In detailhere is how we will do. We have w loops enumerating the indices from j1 to jw, and in the innermost loop,we add the w columns plus the syndrome andeither we test the value of the syndrome or we store itdepending on what is our need. The cost of thisalgorithm is at most w(n,w) column additions plus acertain number of tests. In practice, the costfor the test is negligible and we will just ignorethat term, and we have this cost for the algorithm. But we can do better. Instead, we may keeptrack of partial sums. In the first loop, line 1,we add the syndrome to the first column. In thesecond loop, we add the first partial sum to the second column. We obtain a second partialsum and so on until, in the inner loop, we add theprevious partial sum to the last column. If we dothis, since each line i is executed (n,i)times, we have a total of column additionswhich is given here. And this cost isdominated by (n,w), when w is not too large. So, thetotal cost for the Exhaustive Search is (n,w) column operations. And for that cost, we obtainall the solutions to our problem. We can do better withthe Birthday Decoding. In this algorithm, wesplit the matrix into two equal parts and we willenumerate all solutions by enumerating those twolists using error pattern of weight w/2. If theintersection of those two lists is not empty, then we havea solution to our problem.
Here is the algorithm. We first enumerate allerrors of weight w/2, compute the syndrome and store each of the computed values. Thiswill cost (n/2,w/2), that is the size of the first list. At this point, we may check the intersection of thetwo lists, L1 and L2. This last part of thealgorithm will cost (L1 * L2)/2 to the size of the syndrome. Coming back to theBirthday Decoding slide, the cost of the aboveenumeration will be given by the
formula here with binomialcoefficient which can also be written as 2L + (L²/2^(n-k)) where L is the size common to the two lists. To measure the exactcomplexity of this algorithm, we have to take into accountthat an error pattern of weight w splits evenly between thetwo halves of the matrix with a probability P whichmay be smaller than one.
Intervention / Responsable scientifique
Dans la même collection
-
3.7. May, Meurer, and Thomae Algorithm
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuSo, with the session 7 we are entering the most advanced part of that course. The idea of what I called the Improved Birthday Decoding is to use the so-called "representation technique" introduced
-
3.1. From Generic Decoding to Syndrome Decoding
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuWelcome to the third week of the MOOC on code-based cryptography. This week, we will learn about message attacks. Among the ten sessions of this week, the first six will present the most essential
-
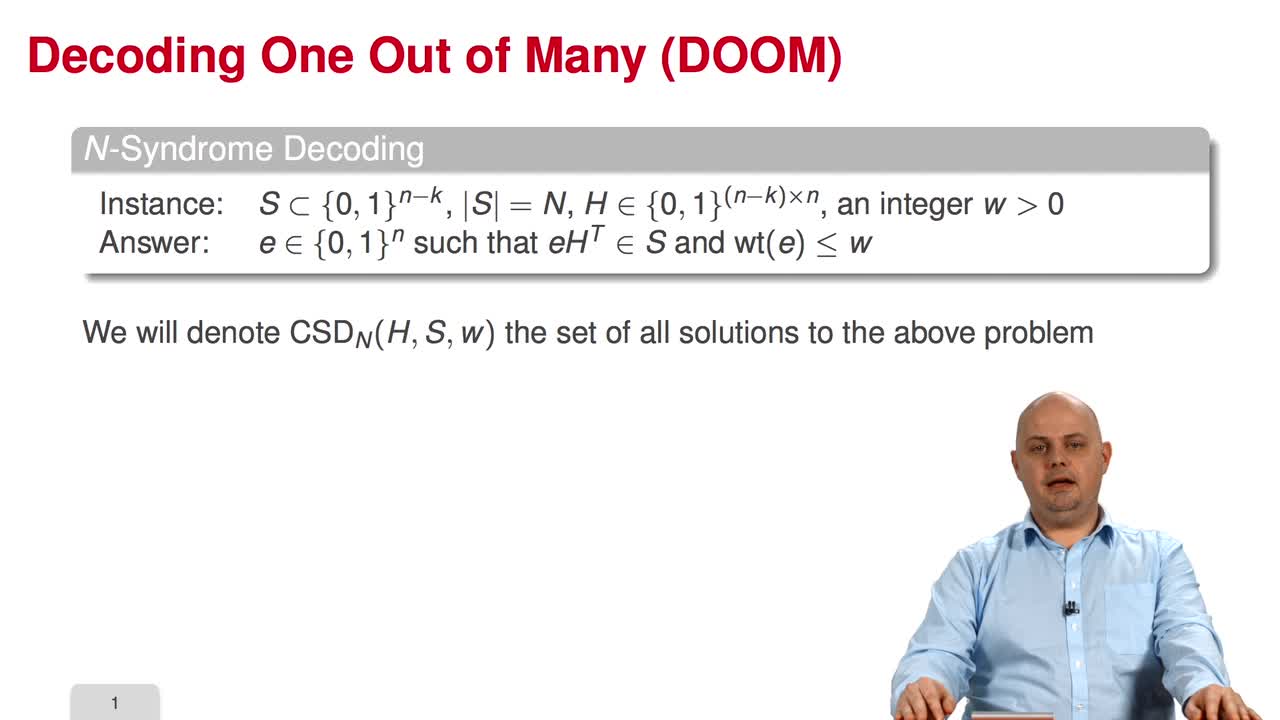
3.10. Decoding One Out of Many
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuThe final session of this week is devoted to Decoding One Out of Many. Decoding One Out of Many is interested in solving the following variant of Syndrome Decoding. In this variant, the only
-
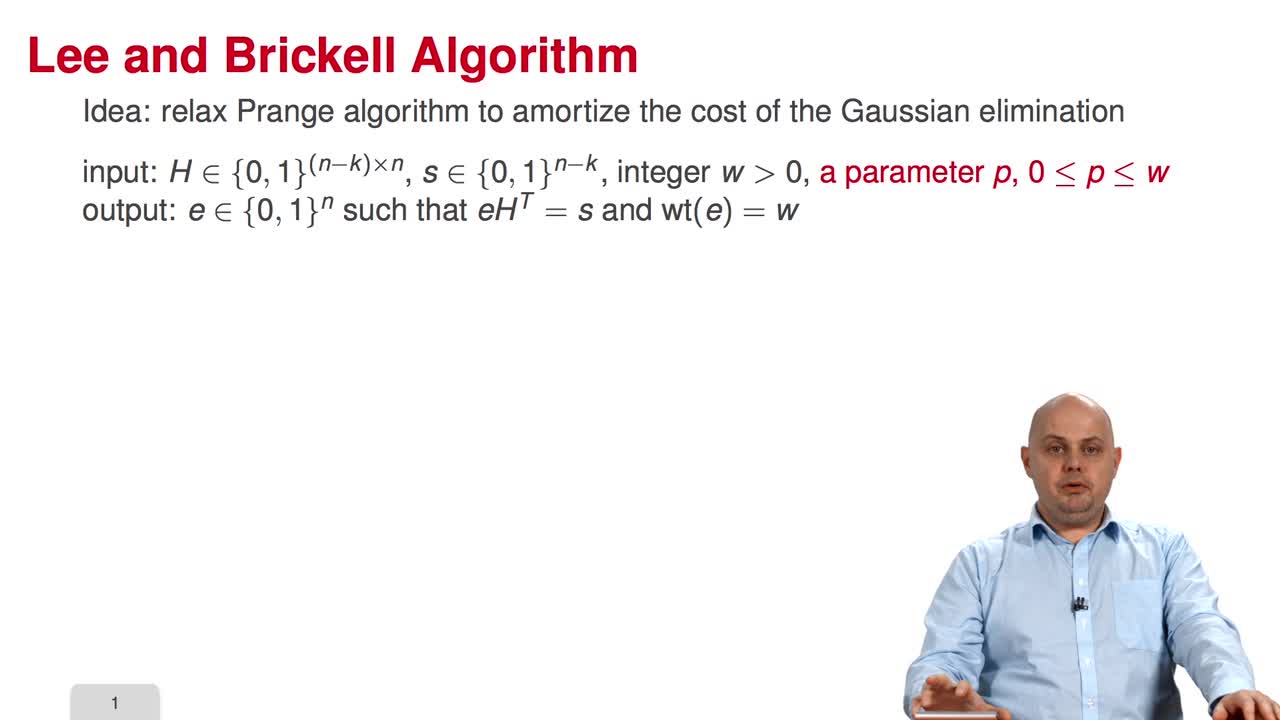
3.5. Lee and Brickell Algorithm
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this fifth session, we will study a variant of information set decoding proposed by Lee and Brickell. So, the main idea consists in relaxing the Prange algorithm to amortize the cost of the
-
3.8. Becker, Joux, May, and Meurer Algorithm
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuNow in session 8, we will present yet another evolution of information set decoding. Before presenting this improvement, we will first improve the Birthday Decoding algorithm what I call a Further
-
3.3. Information Set Decoding: the Power of Linear Algebra
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this third session, we will present the most important concept of the week: Information Set Decoding. The problem of decoding is not only a combinatorial problem. Because we are dealing with
-
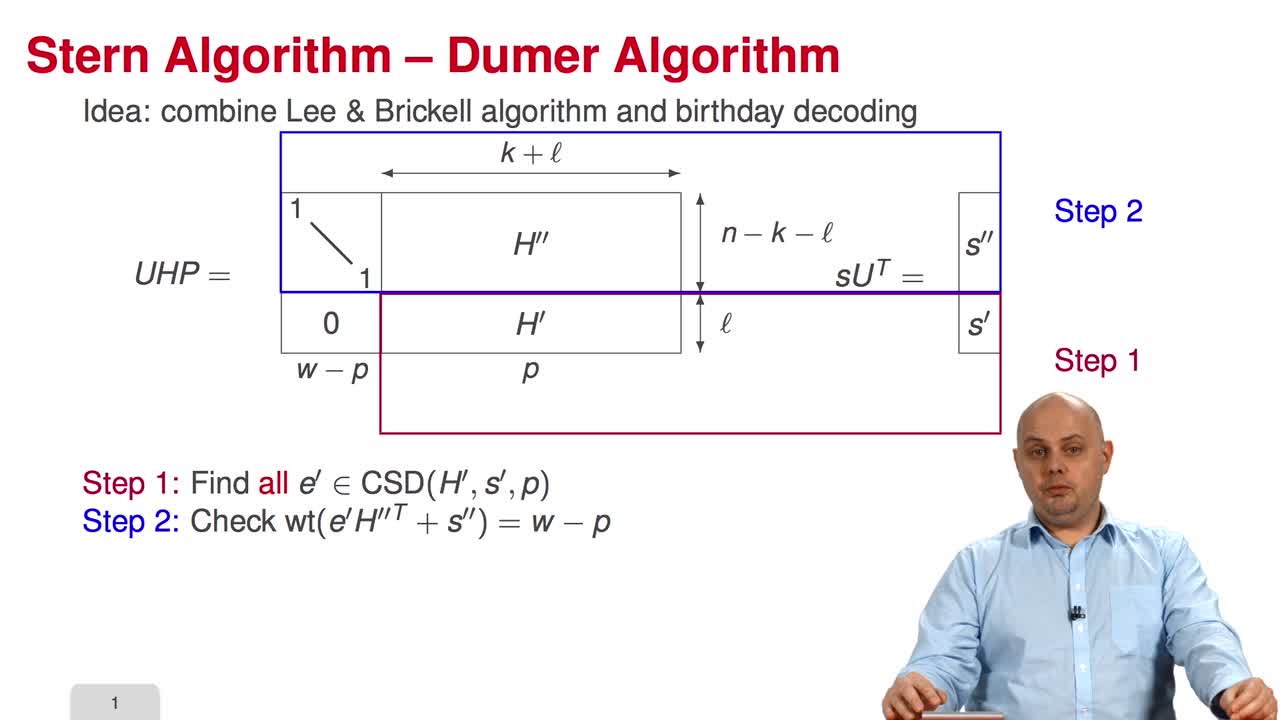
3.6. Stern/Dumer Algorithm
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, we will present the Stern algorithm for decoding. In fact, the idea is to combine two algorithms that we have seen before, the Lee and Brickell algorithm and the Birthday Decoding.
-
3.9. Generalized Birthday Algorithm for Decoding
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuThe session nine is devoted to the application of the Generalized Birthday Algorithm to decoding. The Generalized Birthday Algorithm was presented by David Wagner in 2002, in a more general
-
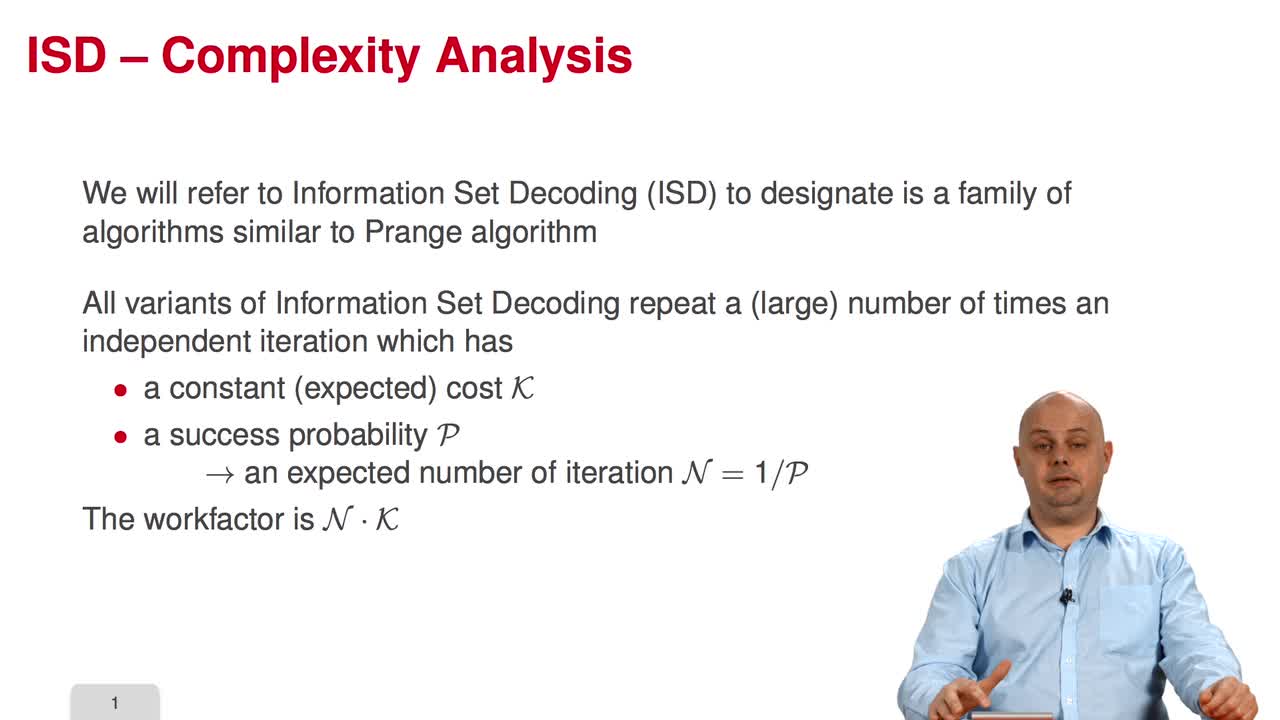
3.4. Complexity Analysis
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, I will present the main technique to make the analysis of the various algorithms presented in this course. So, Information Set Decoding refers to a family of algorithms which is
Avec les mêmes intervenants et intervenantes
-
5.2. The Courtois-Finiasz-Sendrier (CFS) Construction
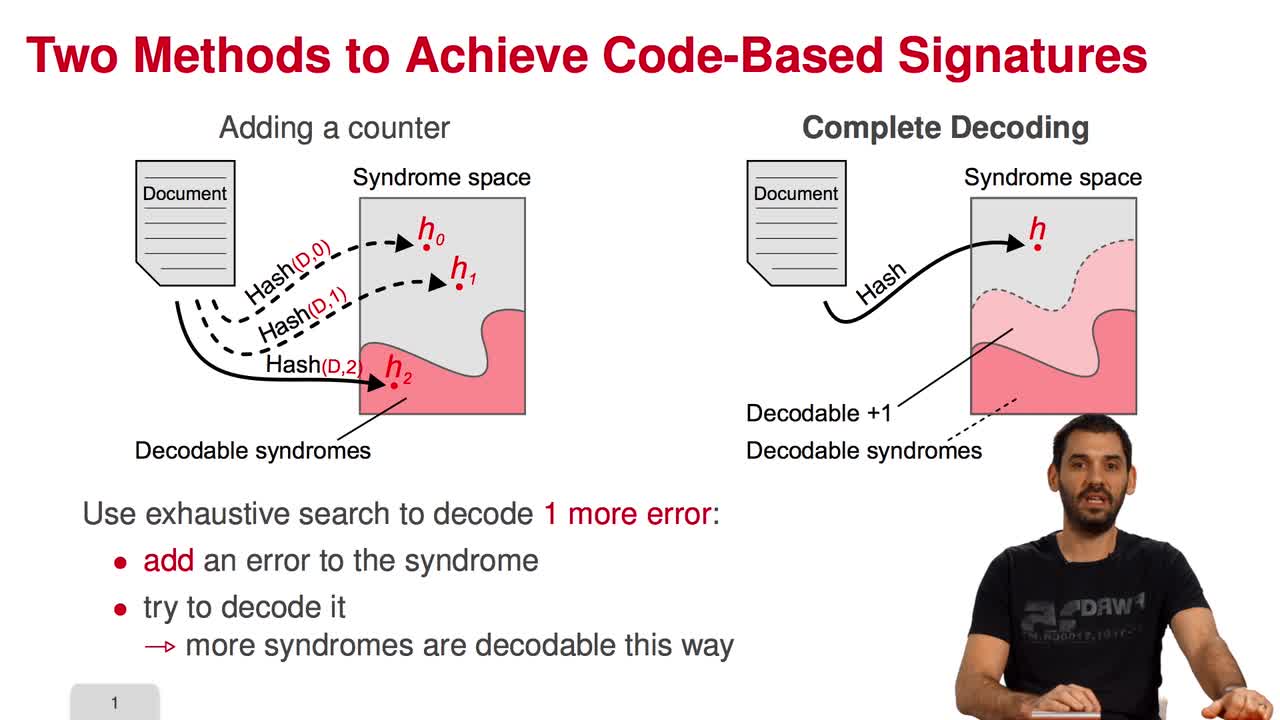
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, I am going to present the Courtois-Finiasz-Sendrier Construction of a code-based digital signature. In the previous session, we have seen that it is impossible to hash a document
-
4.6. Attack against GRS codes
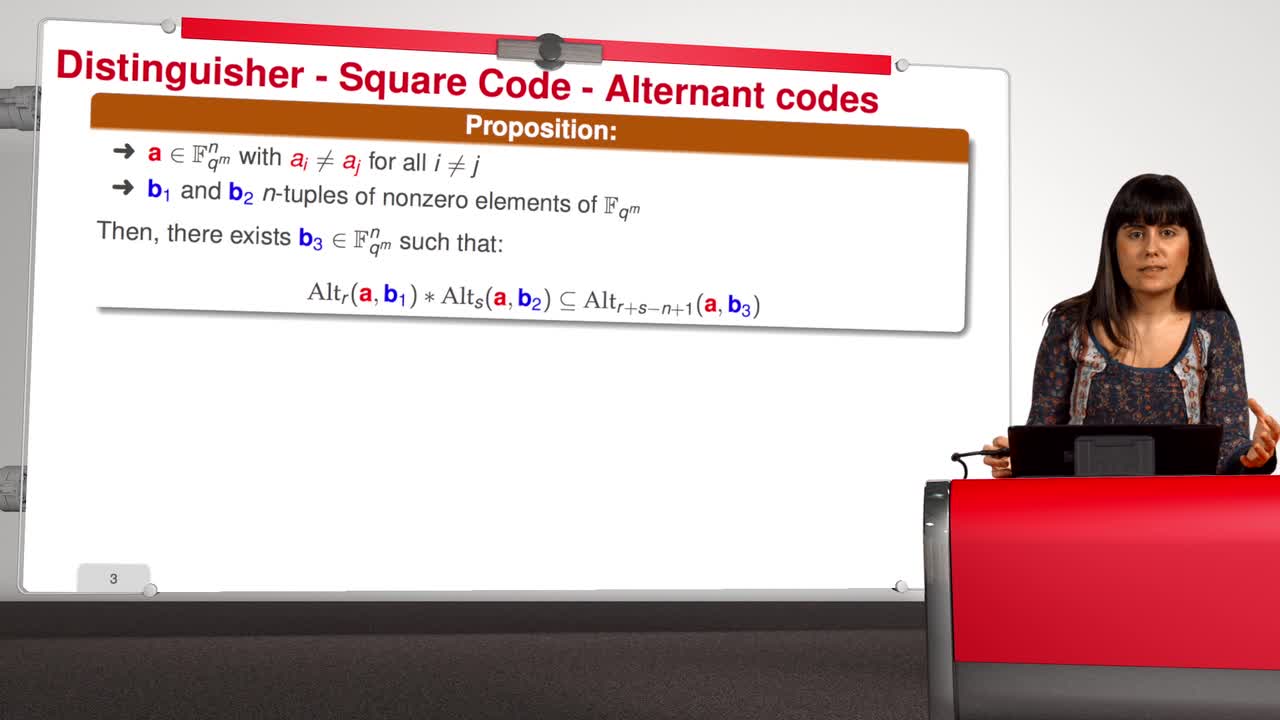
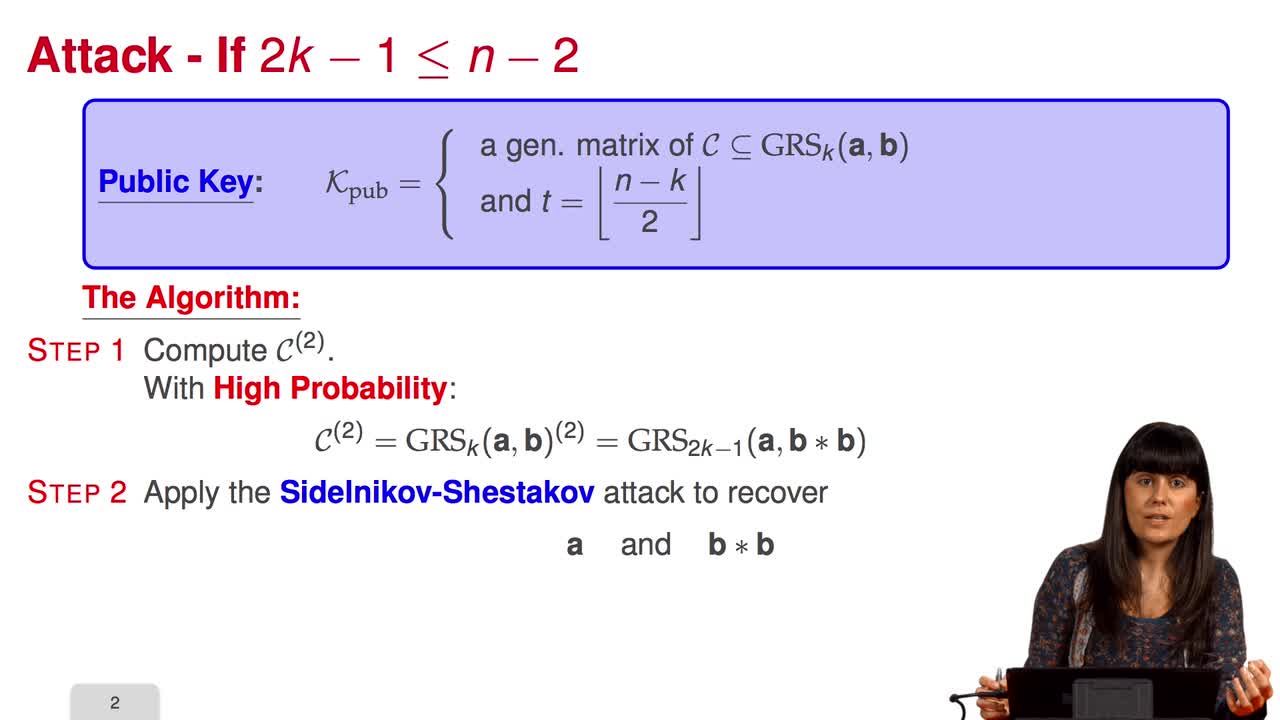
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session we will discuss the proposal of using generalized Reed-Solomon codes for the McEliece cryptosystem. As we have already said, generalized Reed-Solomon codes were proposed in 1986 by
-
5.5. Stern’s Zero-Knowledge Identification Scheme
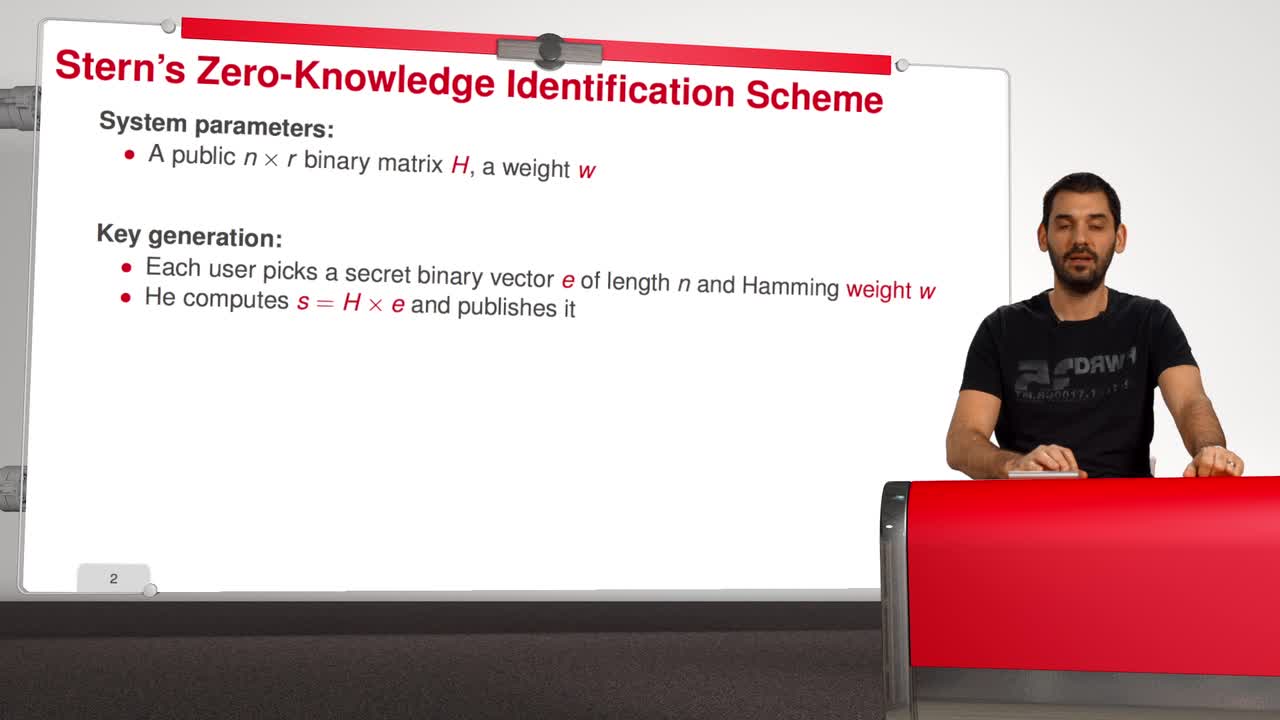
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, we are going to have a look at Stern’s Zero-Knowledge Identification Scheme. So, what is a Zero-Knowledge Identification Scheme? An identification scheme allows a prover to prove
-
4.9. Goppa codes still resist
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuAll the results that we have seen this week doesn't mean that code based cryptography is broken. So in this session we will see that Goppa code still resists to all these attacks. So recall that
-
4.4. Attack against subcodes of GRS codes
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, we will talk about using subcodes of a Generalized Reed–Solomon code for the McEliece Cryptosystem. Recall that to avoid the attack of Sidelnikov and Shestakov, Berger and
-
5.3. Attacks against the CFS Scheme
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, we will have a look at the attacks against the CFS signature scheme. As for public-key encryption, there are two kinds of attacks against signature schemes. First kind of attack is
-
4.7. Attack against Reed-Muller codes
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, we will introduce an attack against binary Reed-Muller codes. Reed-Muller codes were introduced by Muller in 1954 and, later, Reed provided the first efficient decoding algorithm
-
5.6. An Efficient Provably Secure One-Way Function
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, we are going to see how to build an efficient provably secure one-way function from coding theory. As you know, a one-way function is a function which is simple to evaluate and
-
5.1. Code-Based Digital Signatures
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuWelcome to the last week of this MOOC on code-based cryptography. This week, we will be discussing other cryptographic constructions relying on coding theory. We have seen how to do public key
-
4.5. Error-Correcting Pairs
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuWe present in this session a general decoding method for linear codes. And we will see it in an example. Let C be a generalized Reed-Solomon code of dimension k associated to the pair (c, d). Then,
-
5.4. Parallel-CFS
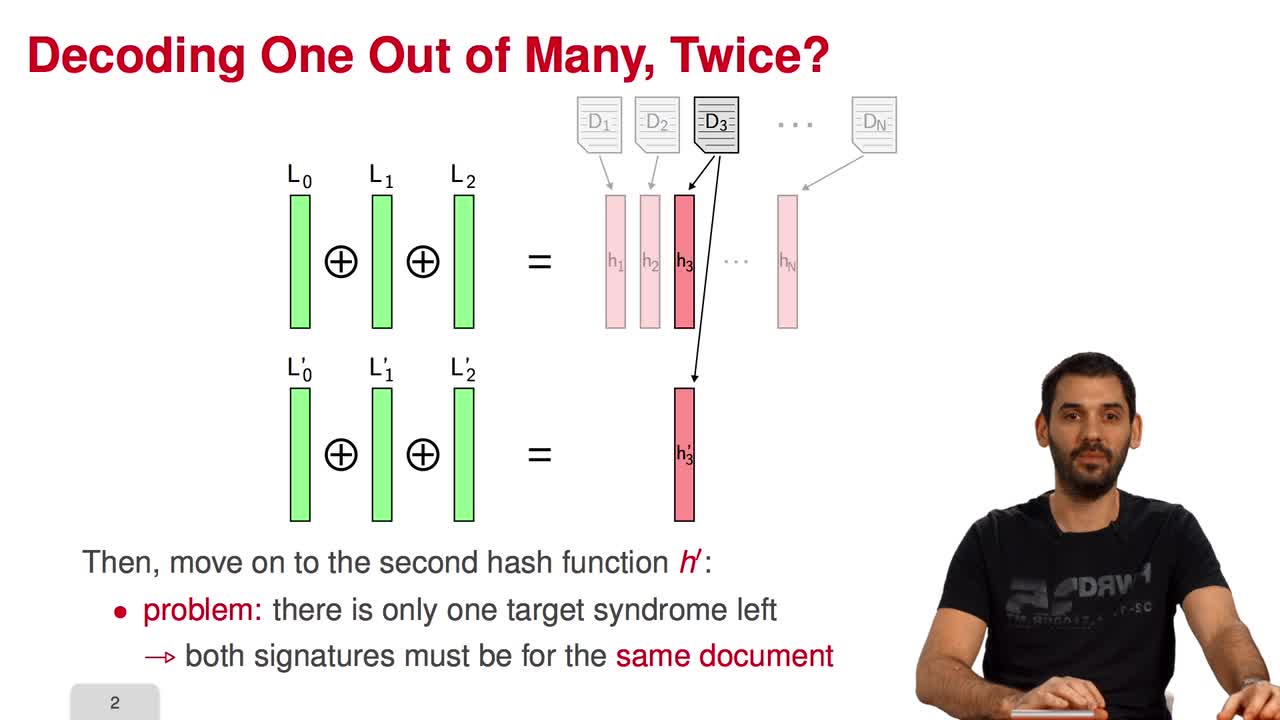
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, I will present a variant of the CFS signature scheme called parallel-CFS. We start from a simple question: what happens if you try to use two different hash functions and compute
-
4.8. Attack against Algebraic Geometry codes
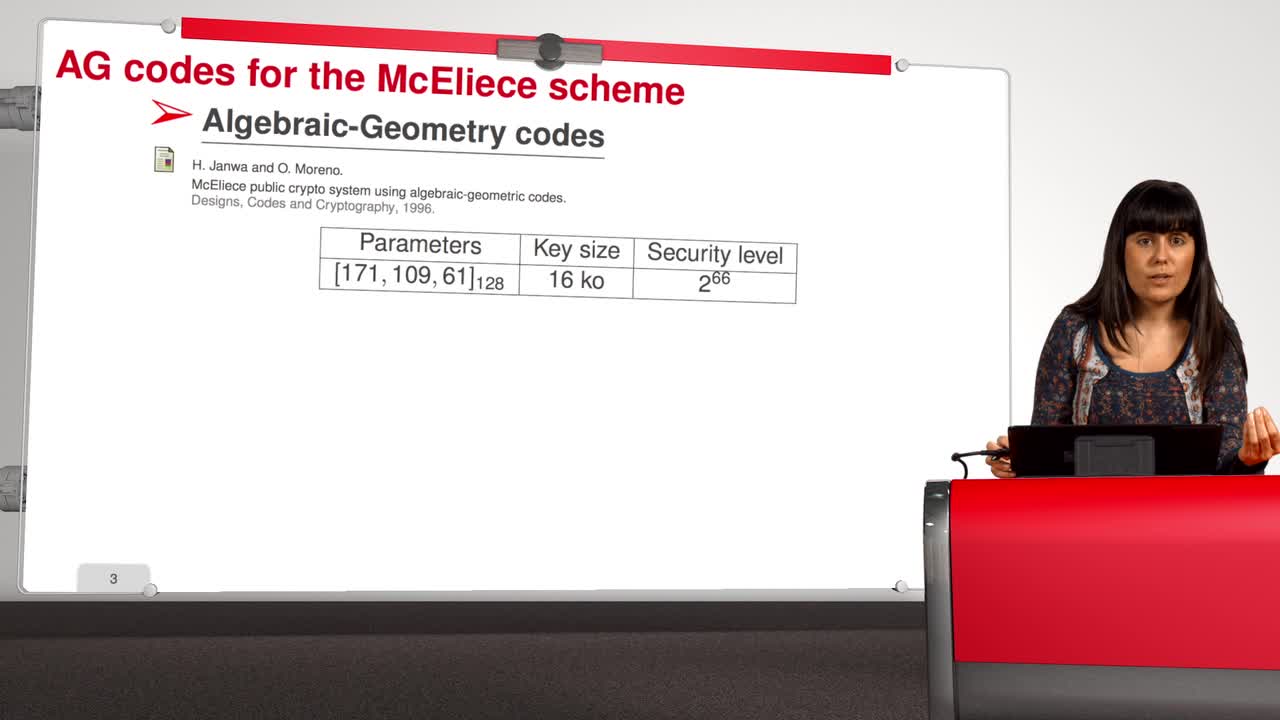
Marquez-CorbellaIreneSendrierNicolasFiniaszMatthieuIn this session, we will present an attack against Algebraic Geometry codes (AG codes). Algebraic Geometry codes is determined by a triple. First of all, an algebraic curve of genus g, then a n
Sur le même thème
-
Machines algorithmiques, mythes et réalités
MazenodVincentVincent Mazenod, informaticien, partage le fruit de ses réflexions sur l'évolution des outils numériques, en lien avec les problématiques de souveraineté, de sécurité et de vie privée...
-
Désassemblons le numérique - #Episode11 : Les algorithmes façonnent-ils notre société ?
SchwartzArnaudLima PillaLaércioEstériePierreSalletFrédéricFerbosAudeRoumanosRayyaChraibi KadoudIkramUn an après le tout premier hackathon sur les méthodologies d'enquêtes journalistiques sur les algorithmes, ce nouvel épisode part à la rencontre de différents points de vue sur les algorithmes.
-
Les machines à enseigner. Du livre à l'IA...
BruillardÉricQue peut-on, que doit-on déléguer à des machines ? C'est l'une des questions explorées par Éric Bruillard qui, du livre aux IA génératives, expose l'évolution des machines à enseigner...
-
Quel est le prix à payer pour la sécurité de nos données ?
MinaudBriceÀ l'ère du tout connecté, la question de la sécurité de nos données personnelles est devenue primordiale. Comment faire pour garder le contrôle de nos données ? Comment déjouer les pièges de plus en
-
Désassemblons le numérique - #Episode9 : Bientôt des supercalculateurs dans nos piscines ?
BeaumontOlivierBouzelRémiDes supercalculateurs feraient-ils bientôt leur apparition dans les piscines municipales pour les chauffer ? Réponses d'Olivier Beaumont, responsable de l'équipe-projet Topal, et Rémi Bouzel,
-
Des systèmes de numération pour le calcul modulaire
BajardJean-ClaudeLe calcul modulaire est utilisé dans de nombreuses applications des mathématiques, telles que la cryptographie...
-
Projection methods for community detection in complex networks
LitvakNellyCommunity detection is one of most prominent tasks in the analysis of complex networks such as social networks, biological networks, and the world wide web. A community is loosely defined as a group
-
Lara Croft. doing fieldwork under surveillance
Dall'AgnolaJasminLara Croft. Doing Fieldwork Under Surveillance Intervention de Jasmin Dall'Agnola (The George Washington University), dans le cadre du Colloque coorganisé par Anders Albrechtslund, professeur en
-
Containing predictive tokens in the EU
CzarnockiJanContaining Predictive Tokens in the EU – Mapping the Laws Against Digital Surveillance, intervention de Jan Czarnocki (KU Leuven), dans le cadre du Colloque coorganisé par Anders Albrechtslund,
-
Inauguration de l'exposition - Vanessa Vitse : Nombres de Sophie Germain et codes secrets
VitseVanessaExposé de Vanessa Vitse (Institut Fourier) : Nombres de Sophie Germain et codes secrets
-
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HerléaAlexandreAlexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
Ivan Murit - Processus de création d'images
MuritIvanJe vais présenter une manière décalée d'aborder les outils d'impression. Pour cela nous ne partirons pas de l'envie d'imprimer une image préexistante, mais d'avant cela : comment se crée une forme