Notice
A. Eskin - Counting closed geodesics on translation surfaces
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
We show that the number of closed geodesics in the flat metric on a translation surface of length at most R is asymptotic to e hR / (hR). This is joint work with Kasra Rafi
Intervention / Responsable scientifique
Dans la même collection
-
Ö. Yurttas - Algorithms for multicurves with Dynnikov coordinates
YurttasÖyküMulticurves have played a fundamental role in the study of mapping class groups of surfaces since the work of Dehn. A beautiful method of describing such systems on the n-punctured disk is given by
-
A. Zorich - Counting simple closed geodesics and volumes of moduli spaces (Part 3)
ZorichAntonIn the first two lectures I will try to tell (or, rather, to give an idea) of how Maryam Mirzakhani has counted simple closed geodesics on hyperbolic surfaces. I plan to briefly mention her
-
A. Zorich - Counting simple closed geodesics and volumes of moduli spaces (Part 1)
ZorichAntonIn the first two lectures I will try to tell (or, rather, to give an idea) of how Maryam Mirzakhani has counted simple closed geodesics on hyperbolic surfaces. I plan to briefly mention her
-
A. Wright - Mirzakhani's work on Earthquakes (Part 2)
WrightAlexanderWe will give the proof of Mirzakhani's theorem that the earthquake flow and Teichmuller unipotent flow are measurably isomorphic. We will assume some familiarity with quadratic differentials, but no
-
L. Liechti - Minimal dilatations on nonorientable surfaces
LiechtiLivioWe discuss the problem of finding the minimal dilatation among pseudo-Anosov mapping classes on a fixed closed surface. In particular, for every nonorientable closed surface of even genus, we consider
-
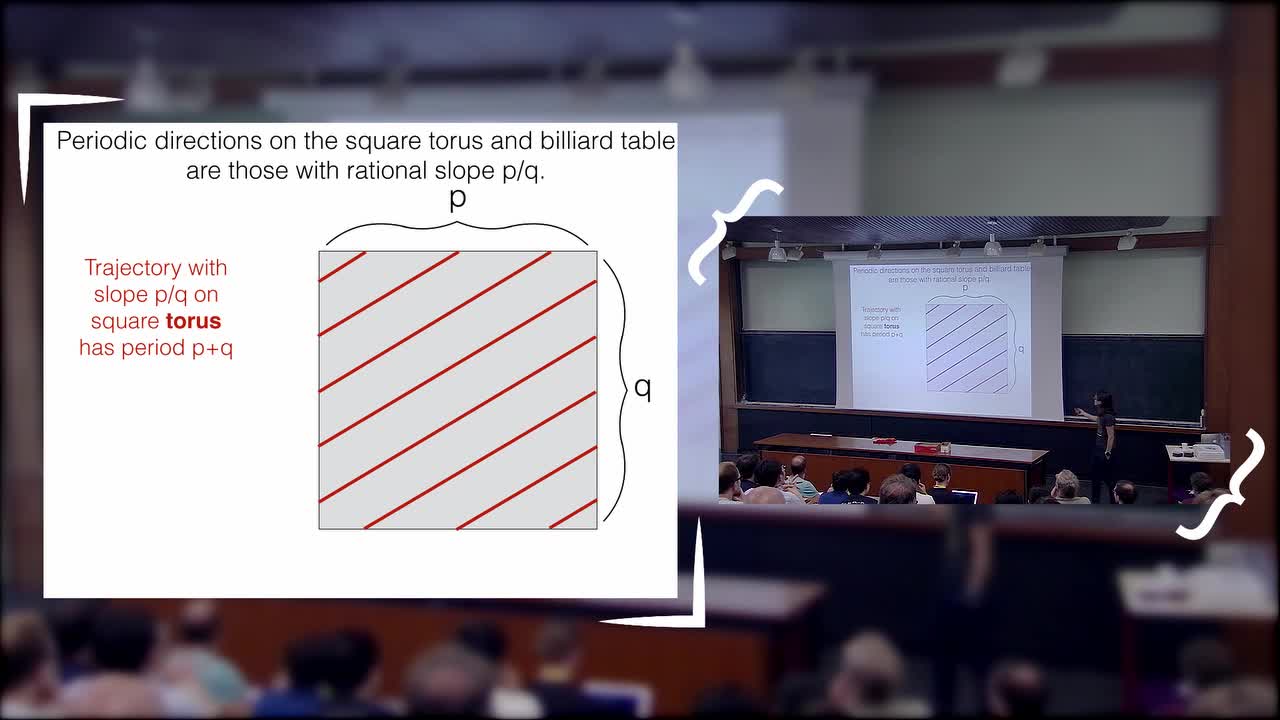
D. Davis - Periodic paths on the pentagon
DavisDianaMathematicians have long understood periodic trajectories on the square billiard table. In the present work, we describe periodic trajectories on the regular pentagon – their geometry, symbolic
-
S. Skripchenko - Rauzy gasket, Arnoux-Yoccoz interval exchange map, Novikov's problem (Part 2)
SkripchenkoAlexandra1. Symbolic dynamics: Arnoux - Rauzy words and Rauzy gasket 2. Topology: Arnoux - Yoccoz example and its generalization 3. Novikov’s problem: how dynamics meets topology and together they help to
-
V. Gadre - Effective convergence of ergodic averages and cusp excursions of geodesics
Effective convergence of ergodic averages and cusp excursions of geodesics on moduli spaces We survey some applications of effective convergence of ergodic averages to the analysis of cusp ex
-
P. Apisa - Marked points in genus two and beyond
ApisaPaulIn the principal stratum in genus two, McMullen observed that something odd happens - there is only one nonarithmetic Teichmuller curve - the one generated by the decagon. This strange phenomenon
-
C. Matheus - Square tiled surfaces (Part 2)
MatheusCarlosa) basic definitions and examples b) strata and genus c) reduced and primitive origamis, SL(2,R) action, Veech groups d) automorphisms and affine homeomorphisms e) homology of origamis f) Kontsevich
-
S. Filip - K3 surfaces and Dynamics (Part 1)
SimionFilipK3 surfaces provide a meeting ground for geometry (algebraic, differential), arithmetic, and dynamics. I hope to discuss: Basic definitions and examples - Geometry (algebraic, differential, etc.) of
-
A. Wright - Mirzakhani's work on Earthquakes (Part 1)
WrightAlexanderWe will give the proof of Mirzakhani's theorem that the earthquake flow and Teichmuller unipotent flow are measurably isomorphic. We will assume some familiarity with quadratic differentials, but no
Sur le même thème
-
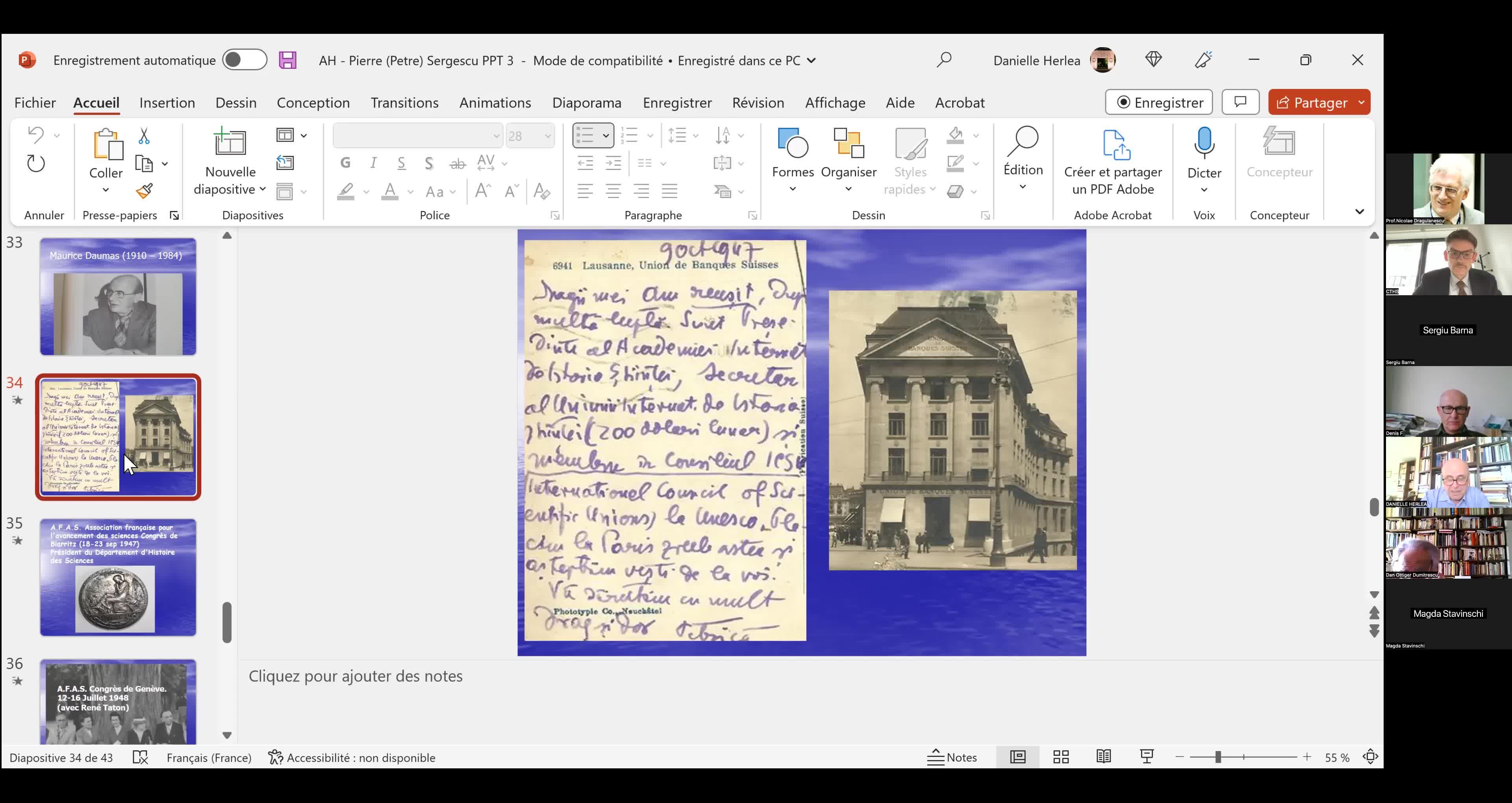
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HerléaAlexandreAlexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
Webinaire sur la rédaction des PGD
LouvetViolaineRédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
R. Bamler - Compactness and partial regularity theory of Ricci flows in higher dimensions
BamlerRichard H.We present a new compactness theory of Ricci flows. This theory states that any sequence of Ricci flows that is pointed in an appropriate sense, subsequentially converges to a synthetic flow.
-
C. Li - Classifying sufficiently connected PSC manifolds in 4 and 5 dimensions
LiChaoIn this talk, I will discuss some recent developments on the topology of closed manifolds admitting Riemannian metrics of positive scalar curvature. In particular, we will prove if a closed PSC
-
T. Ozuch - Noncollapsed degeneration and desingularization of Einstein 4-manifolds
OzuchTristanWe study the noncollapsed singularity formation of Einstein 4-manifolds. We prove that any smooth Einstein 4-manifold close to a singular one in a mere Gromov-Hausdorff (GH) sense is the result
-
D. Tewodrose - Limits of Riemannian manifolds satisfying a uniform Kato condition
TewodroseDavidPresentation of a joint work with G. Carron and I. Mondello where we study Kato limit spaces.
-
J. Wang - Topological rigidity and positive scalar curvature
WangJianIn this talk, we shall describe some topological rigidity and its relationship with positive scalar curvature. Precisely, we will present a proof that a complete contractible 3-manifold with
-
A. Mondino - Time-like Ricci curvature bounds via optimal transport
MondinoAndreaThe goal of the talk is to present a recent work in collaboration with Cavalletti (SISSA) on optimal transport in Lorentzian synthetic spaces. The aim is to set up a “Lorentzian analog” of the
-
M. Lesourd - Positive Scalar Curvature on Noncompact Manifolds and the Positive Mass Theorem
LesourdMartinThe study of positive scalar curvature on noncompact manifolds has seen significant progress in the last few years. A major role has been played by Gromov's results and conjectures, and in
-
R. Perales - Recent Intrinsic Flat Convergence Theorems
PeralesRaquelThéorèmes récents de convergence plane intrinsèque
-
J. Fine - Knots, minimal surfaces and J-holomorphic curves
FineJoëlI will describe work in progress, parts of which are joint with Marcelo Alves. Let L be a knot or link in the 3-sphere. I will explain how one can count minimal surfaces in hyperbolic 4-space