Notice
1.2. Introduction II - Coding Theory
- document 1 document 2 document 3
- niveau 1 niveau 2 niveau 3
Descriptif
In this session, we will give a brief introduction to Coding Theory. Claude Shannon's paper from 1948 entitled "A Mathematical Theory of Communication" gave birth to the disciplines of Information Theory and Coding Theory. The main goal of these disciplines is efficient transfer of reliable information. To be efficient, the transfer of information must not require a big amount of time and effort. To be reliable, the transmitted and received data must resemble. However, during the transmission over a noisy channel, the information will be damaged. So, it has become necessary to develop ways of detecting when an error has occurred and to correct it. For example, we may think of the transmission of satellite photos taken in space and sent back to the earth. So, here is an example of communication. We begin by selecting an alphabet. Then, every original message is presented, as a set of k-tuples of the chosen alphabet. We have k symbols of information and we add n - k redundant symbols to obtain a codeword of length n with n greater than k.
Intervention
Dans la même collection
-
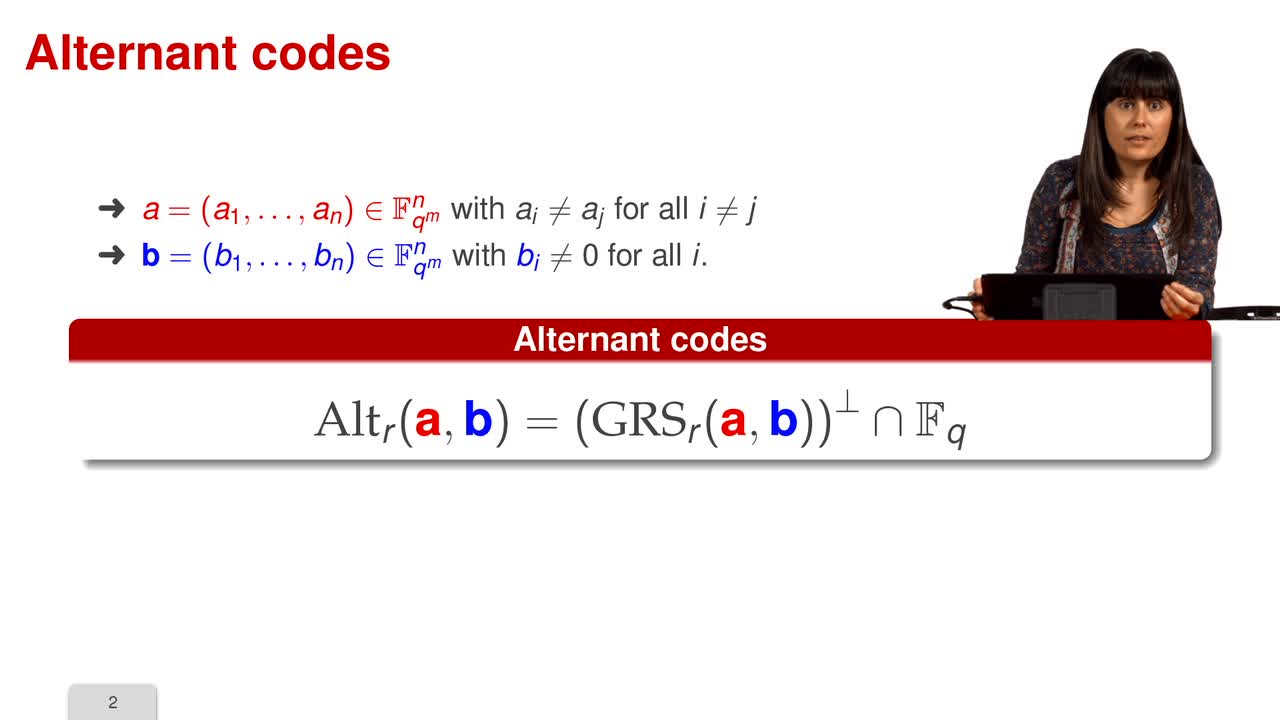
1.7. Reed-Solomon Codes
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
Reed-Solomon codes were introduced by Reed and Solomon in the 1960s. These codes are still used in storage device, from compact-disc player to deep-space application. And they are widely used
-
1.5. Error Correcting Capacity
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
This sequence will be about the error-correcting capacity of a linear code. We describe the way of considering the space Fq^n as a metric space. This metric is necessary to justify the principle of
-
1.8. Goppa Codes
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
In this session, we will talk about another family of codes that have an efficient decoding algorithm: the Goppa codes. One limitation of the generalized Reed-Solomon codes is the fact that the
-
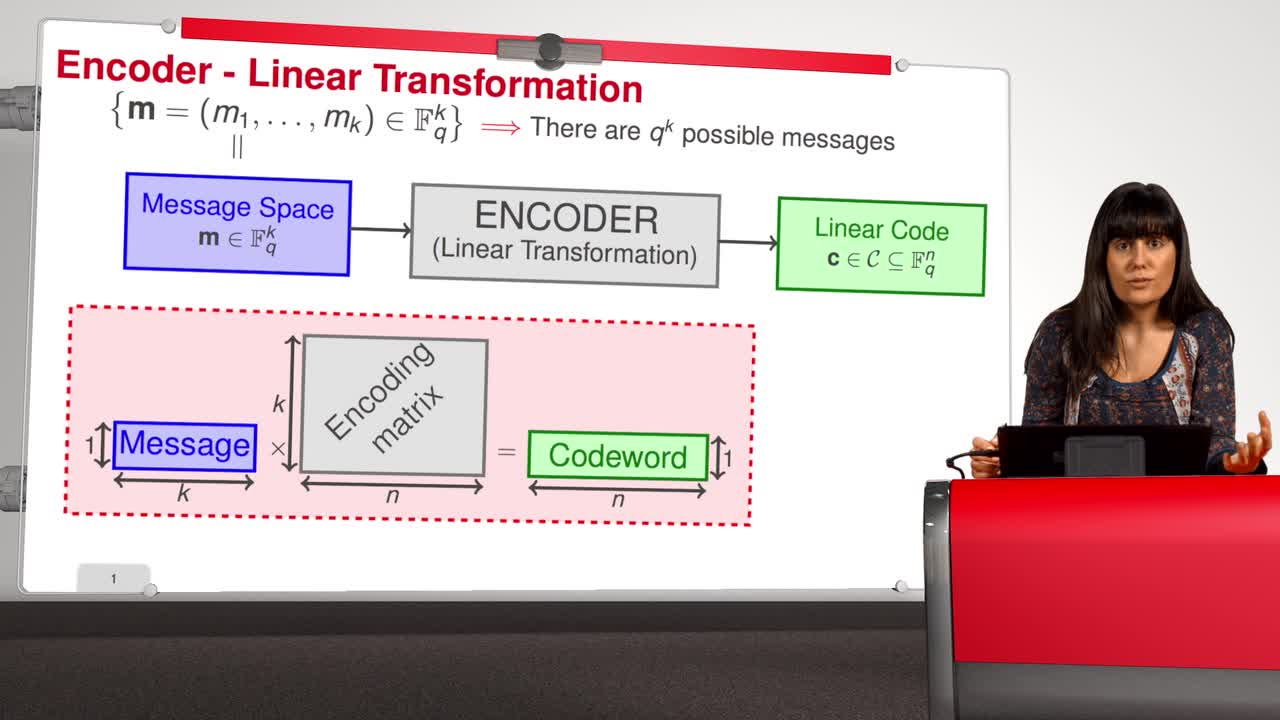
1.3. Encoding (Linear Transformation)
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
In this session, we will talk about the easy map of the - one-way trapdoor functions based on error-correcting codes. We suppose that the set of all messages that we wish to transmit is the set
-
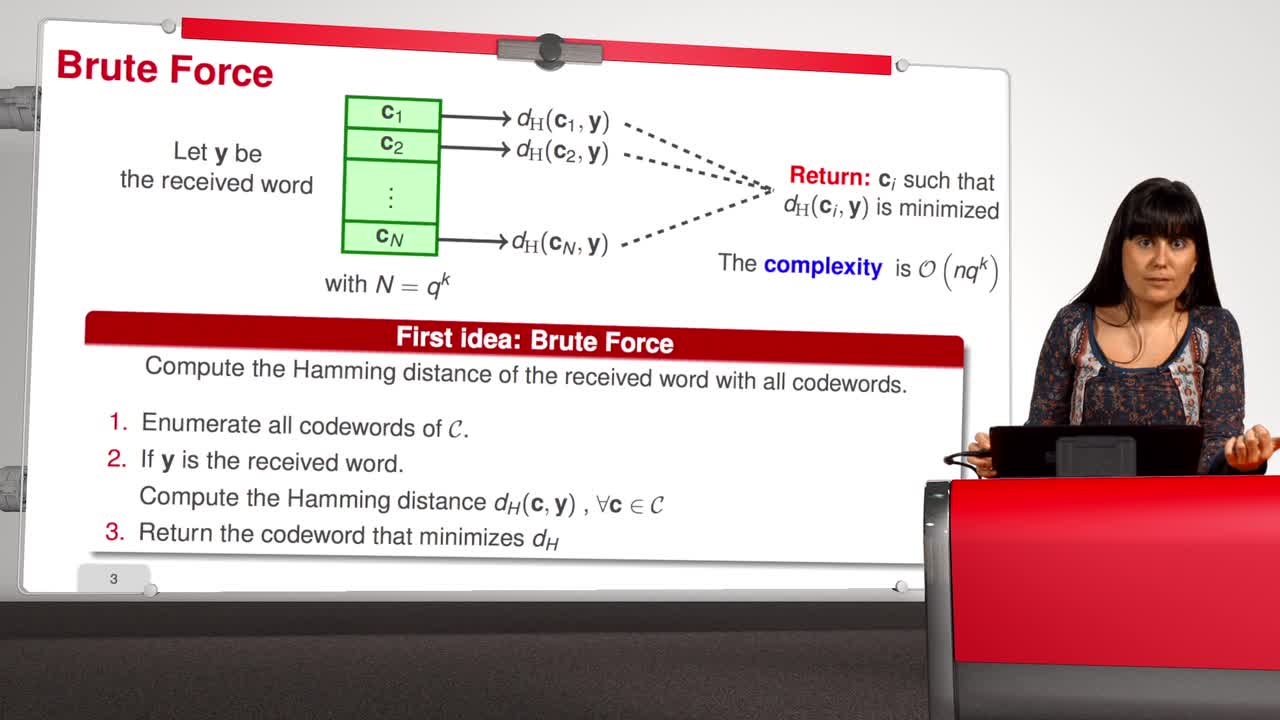
1.6. Decoding (A Difficult Problem)
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
The process of correcting errors and obtaining back the message is called decoding. In this sequence, we will focus on this process, the decoding. We would like that the decoder of the received
-
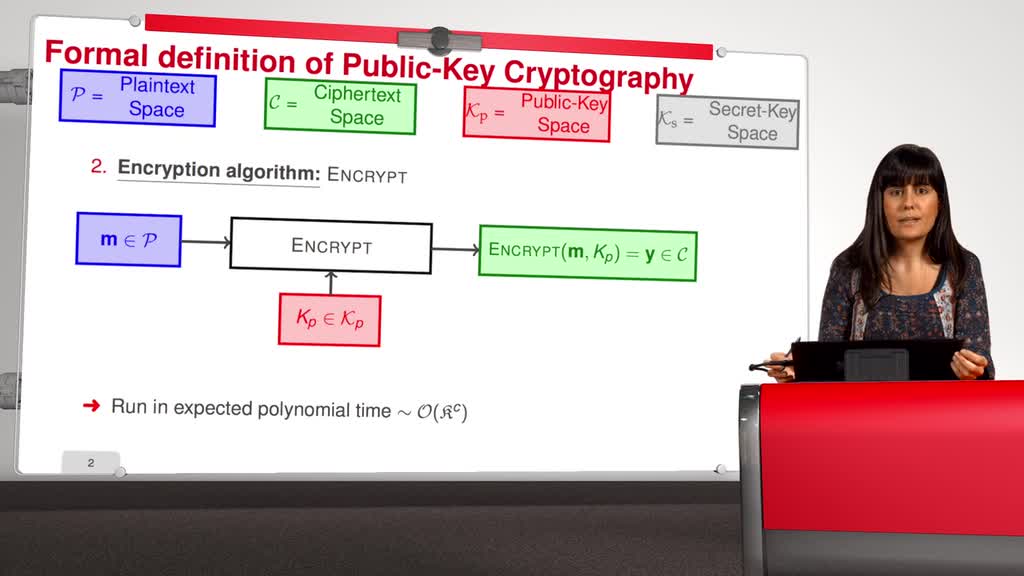
1.9. McEliece Cryptosystem
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
This is the last session of the first week of this MOOC. We have already all the ingredients to talk about code-based cryptography. Recall that in 1976 Diffie and Hellman published their famous
-
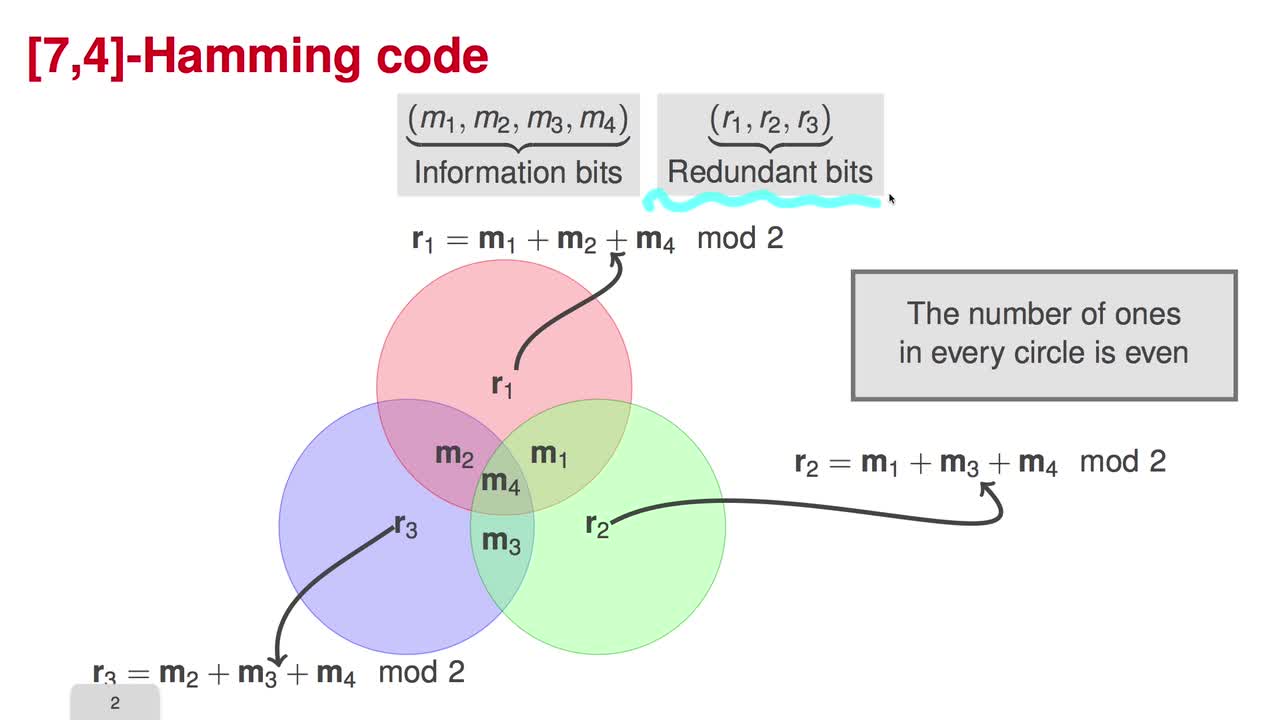
1.4. Parity Checking
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
There are two standard ways to describe a subspace, explicitly by giving a basis, or implicitly, by the solution space of the set of homogeneous linear equations. Therefore, there are two ways of
-
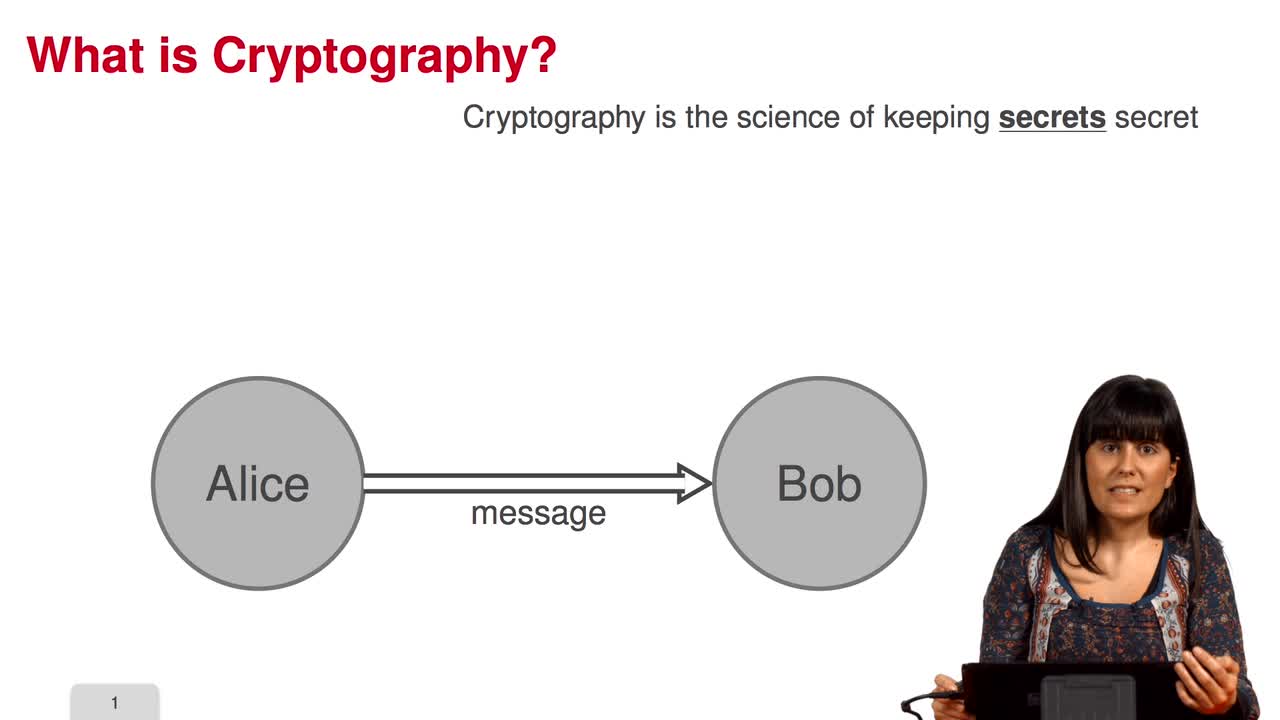
1.1. Introduction I - Cryptography
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
Welcome to this MOOC which is entitled: code-based cryptography. This MOOC is divided in five weeks. The first week, we will talk about error-correcting codes and cryptography, this is an introduction
Avec les mêmes intervenants et intervenantes
-
4.9. Goppa codes still resist
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
All the results that we have seen this week doesn't mean that code based cryptography is broken. So in this session we will see that Goppa code still resists to all these attacks. So recall that
-
4.4. Attack against subcodes of GRS codes
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
In this session, we will talk about using subcodes of a Generalized Reed–Solomon code for the McEliece Cryptosystem. Recall that to avoid the attack of Sidelnikov and Shestakov, Berger and
-
5.3. Attacks against the CFS Scheme
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
In this session, we will have a look at the attacks against the CFS signature scheme. As for public-key encryption, there are two kinds of attacks against signature schemes. First kind of attack is
-
4.7. Attack against Reed-Muller codes
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
In this session, we will introduce an attack against binary Reed-Muller codes. Reed-Muller codes were introduced by Muller in 1954 and, later, Reed provided the first efficient decoding algorithm
-
5.6. An Efficient Provably Secure One-Way Function
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
In this session, we are going to see how to build an efficient provably secure one-way function from coding theory. As you know, a one-way function is a function which is simple to evaluate and
-
5.1. Code-Based Digital Signatures
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
Welcome to the last week of this MOOC on code-based cryptography. This week, we will be discussing other cryptographic constructions relying on coding theory. We have seen how to do public key
-
4.5. Error-Correcting Pairs
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
We present in this session a general decoding method for linear codes. And we will see it in an example. Let C be a generalized Reed-Solomon code of dimension k associated to the pair (c, d). Then,
-
5.4. Parallel-CFS
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
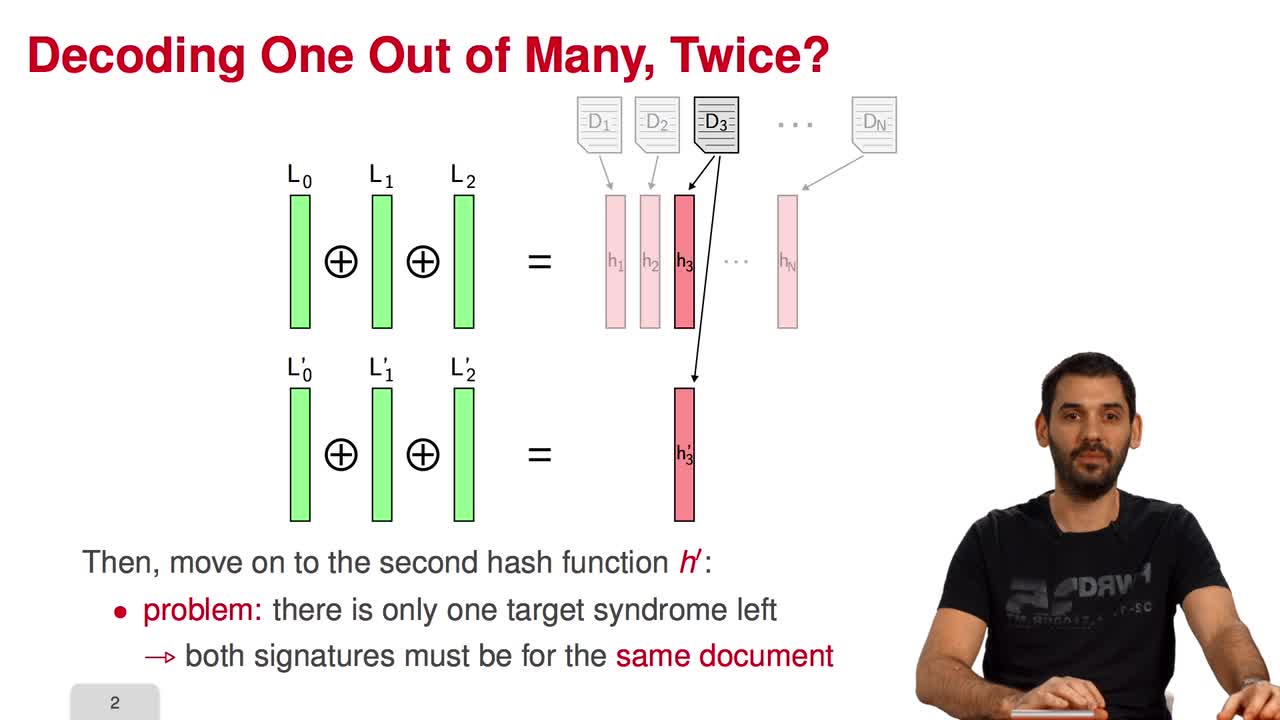
In this session, I will present a variant of the CFS signature scheme called parallel-CFS. We start from a simple question: what happens if you try to use two different hash functions and compute
-
4.8. Attack against Algebraic Geometry codes
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
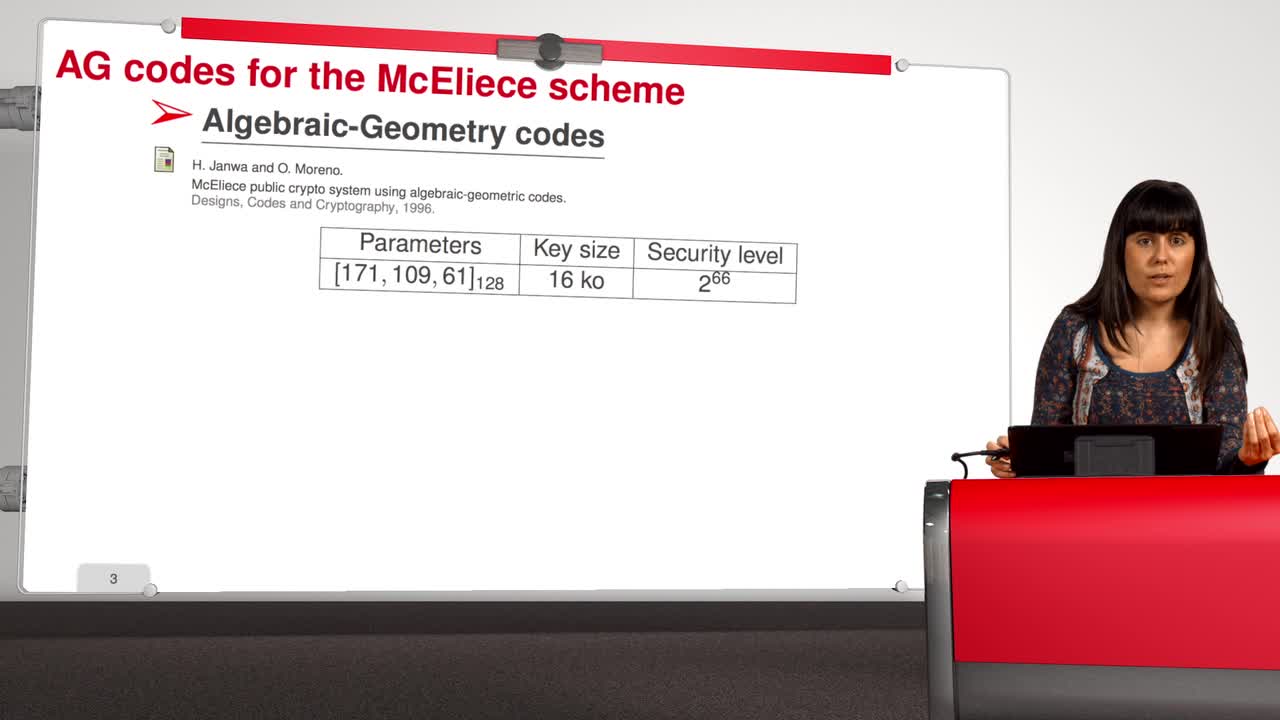
In this session, we will present an attack against Algebraic Geometry codes (AG codes). Algebraic Geometry codes is determined by a triple. First of all, an algebraic curve of genus g, then a n
-
5.7. The Fast Syndrome-Based (FSB) Hash Function
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
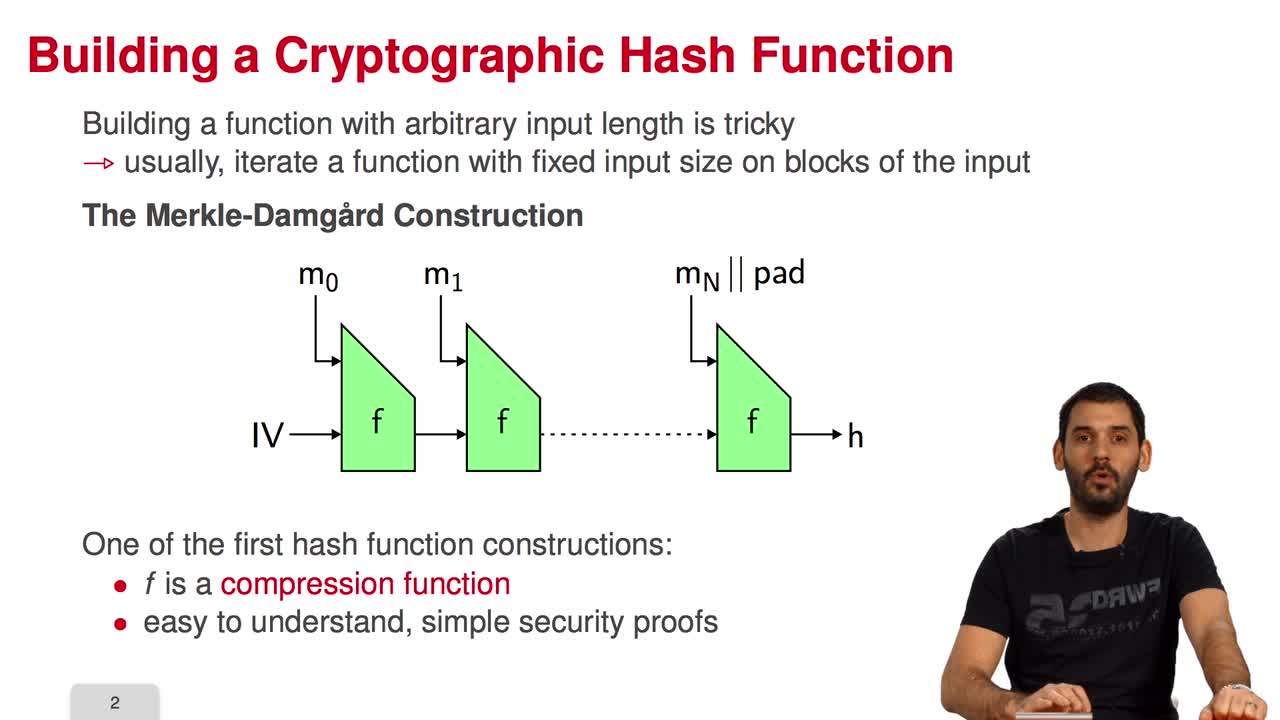
In the last session of this week, we will have a look at the FSB Hash Function which is built using the one-way function we saw in the previous session. What are the requirements for a
-
5.2. The Courtois-Finiasz-Sendrier (CFS) Construction
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
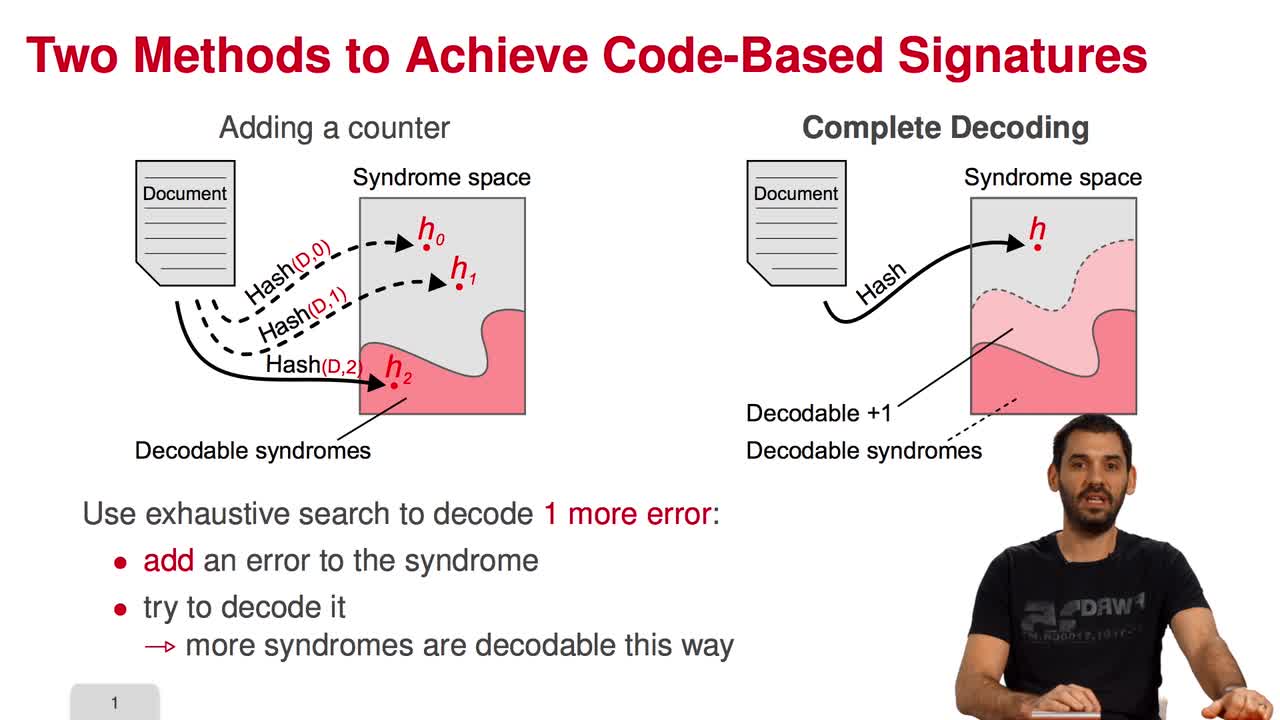
In this session, I am going to present the Courtois-Finiasz-Sendrier Construction of a code-based digital signature. In the previous session, we have seen that it is impossible to hash a document
-
4.6. Attack against GRS codes
MARQUEZ-CORBELLA Irene
SENDRIER Nicolas
FINIASZ Matthieu
In this session we will discuss the proposal of using generalized Reed-Solomon codes for the McEliece cryptosystem. As we have already said, generalized Reed-Solomon codes were proposed in 1986 by
Sur le même thème
-
Quel est le prix à payer pour la sécurité de nos données ?
MINAUD Brice
À l'ère du tout connecté, la question de la sécurité de nos données personnelles est devenue primordiale. Comment faire pour garder le contrôle de nos données ? Comment déjouer les pièges de plus en
-
Des systèmes de numération pour le calcul modulaire
BAJARD Jean-Claude
Le calcul modulaire est utilisé dans de nombreuses applications des mathématiques, telles que la cryptographie...
-
Inauguration de l'exposition - Vanessa Vitse : Nombres de Sophie Germain et codes secrets
VITSE Vanessa
Exposé de Vanessa Vitse (Institut Fourier) : Nombres de Sophie Germain et codes secrets
-
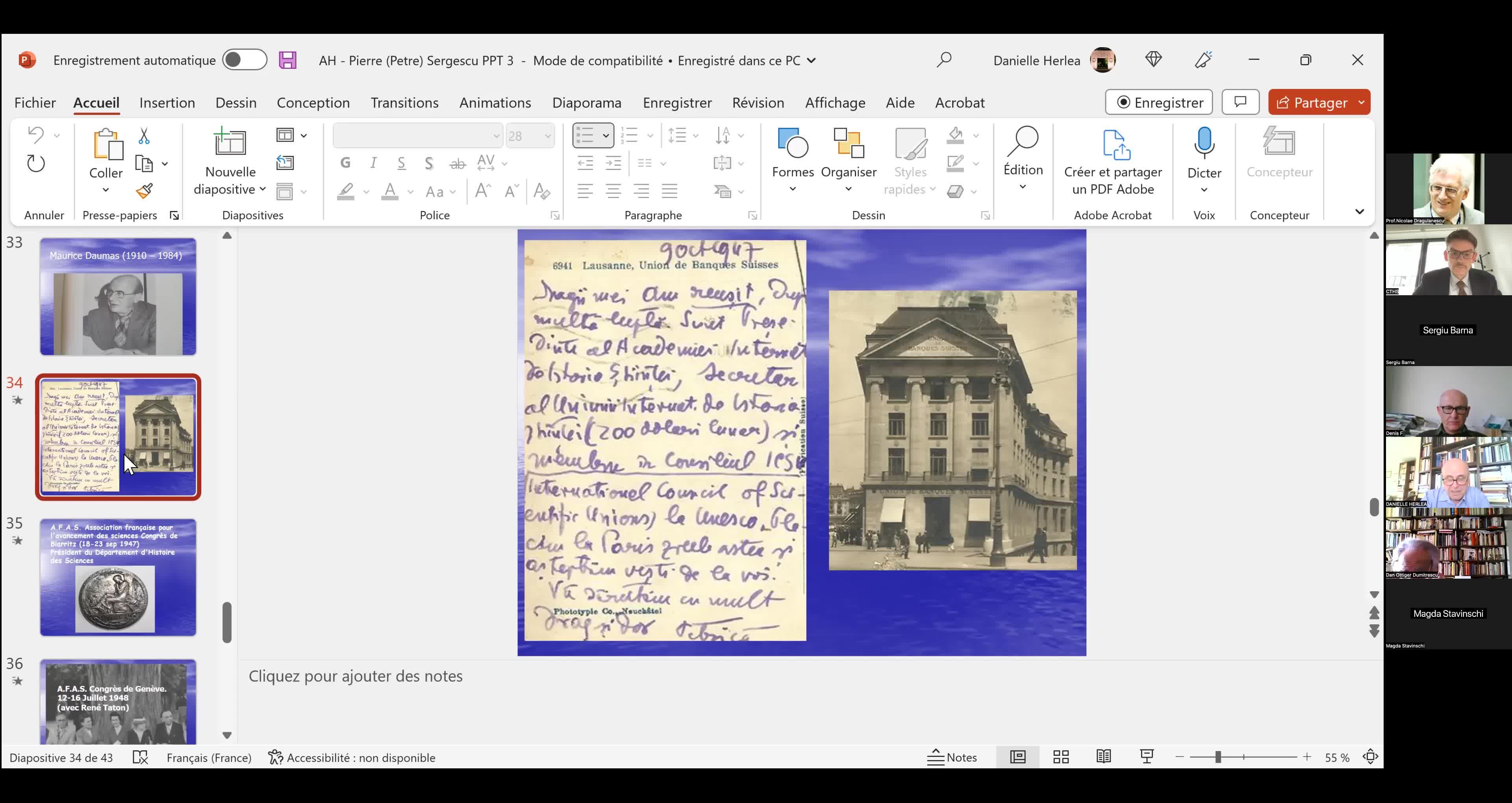
"Le mathématicien Petre (Pierre) Sergescu, historien des sciences, personnalité du XXe siècle"
HERLéA Alexandre
Alexandre HERLEA est membre de la section « Sciences, histoire des sciences et des techniques et archéologie industrielle » du CTHS. Professeur émérite des universités, membre effectif de l'Académie
-
Retour d'expérience sur l'utilisation croisée de plusieurs archives de fouilles
TUFFéRY Christophe
Dans le cadre d'une thèse de doctorat engagée depuis 2019, une étude historiographique et épistémologique des effets des dispositifs numériques sur l'archéologie et sur les archéologues au cours des
-
Information Structures for Privacy and Fairness
PALAMIDESSI Catuscia
Information Structures for Privacy and Fairness
-
AI and Human Decision-Making: An Interdisciplinary Perspective
CASTELLUCCIA Claude
This seminar will talk about some of the privacy risks of these systems and will describe some recent attacks. It will also discuss why they sometimes fail to deliver. Finally, we will also show that
-
Webinaire sur la rédaction des PGD
LOUVET Violaine
Rédaction des Plans de Gestion de Données (PGD) sous l’angle des besoins de la communauté mathématique.
-
Alexandre Booms : « Usage de matériel pédagogique adapté en géométrie : une transposition à interro…
« Usage de matériel pédagogique adapté en géométrie : une transposition à interroger ». Alexandre Booms, doctorant (Université de Reims Champagne-Ardenne - Cérep UR 4692)
-
Présentation de la rencontre. A l’heure du numérique « Quelles mesures pour la mesure ? ». Le relev…
BADIE Alain
TARDY Dominique
MALMARY Jean-Jacques
ZUGMEYER Stéphanie
Rencontre-Atelier de l'ANR Ornementation Architecturale des Gaules. À l’heure du numérique, « Quelles mesures pour la mesure ? ». Le relevé des blocs d’architecture décorés et l’apport des outils
-
Photogrammétrie : Performances et limitations
EGELS Yves
Rencontre-Atelier de l'ANR Ornementation Architecturale des Gaules. À l’heure du numérique, « Quelles mesures pour la mesure ? ». Le relevé des blocs d’architecture décorés et l’apport des outils
-
Relever et publier des blocs d’architecture : objectifs et méthodes
BADIE Alain
Rencontre-Atelier de l'ANR Ornementation Architecturale des Gaules. À l’heure du numérique, « Quelles mesures pour la mesure ? ». Le relevé des blocs d’architecture décorés et l’apport des outils